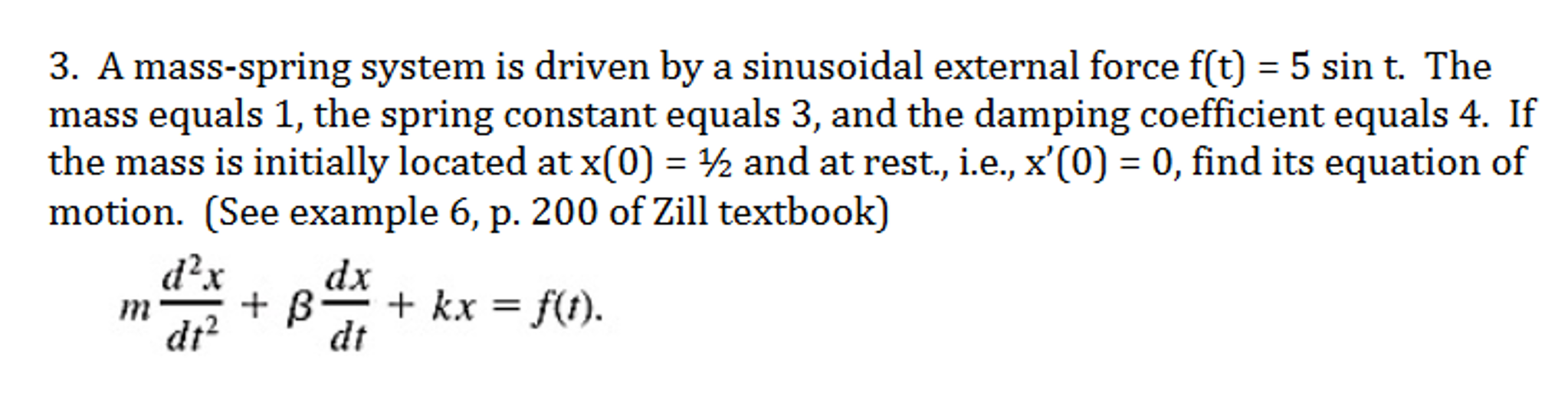Spring Differential Equation - Dt2 hooke's law is a principle of physics that states that the force f needed to extend or compress a spring by some distance. We will do this by equating forces. We want to find all the forces on each mass. F = ma = m = my00: So we can solve it by the methods discussed in. Suppose a \(64\) lb weight stretches a spring \(6\) inches in equilibrium and a dashpot provides a damping force of \(c\) lb for.
We want to find all the forces on each mass. So we can solve it by the methods discussed in. Suppose a \(64\) lb weight stretches a spring \(6\) inches in equilibrium and a dashpot provides a damping force of \(c\) lb for. F = ma = m = my00: We will do this by equating forces. Dt2 hooke's law is a principle of physics that states that the force f needed to extend or compress a spring by some distance.
So we can solve it by the methods discussed in. F = ma = m = my00: Suppose a \(64\) lb weight stretches a spring \(6\) inches in equilibrium and a dashpot provides a damping force of \(c\) lb for. We will do this by equating forces. We want to find all the forces on each mass. Dt2 hooke's law is a principle of physics that states that the force f needed to extend or compress a spring by some distance.
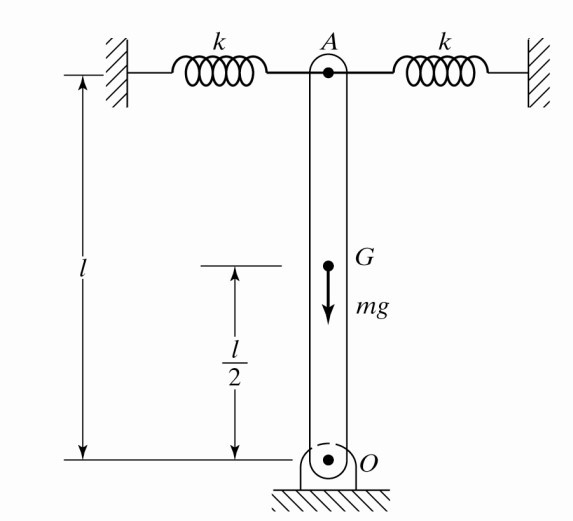
Solved Write the differential equation for the spring bar
F = ma = m = my00: We will do this by equating forces. So we can solve it by the methods discussed in. We want to find all the forces on each mass. Dt2 hooke's law is a principle of physics that states that the force f needed to extend or compress a spring by some distance.
Solved A massspring system is modelled by the differential
So we can solve it by the methods discussed in. Dt2 hooke's law is a principle of physics that states that the force f needed to extend or compress a spring by some distance. We want to find all the forces on each mass. F = ma = m = my00: Suppose a \(64\) lb weight stretches a spring \(6\).
The differential equation obtained by the student Download Scientific
Suppose a \(64\) lb weight stretches a spring \(6\) inches in equilibrium and a dashpot provides a damping force of \(c\) lb for. We want to find all the forces on each mass. We will do this by equating forces. So we can solve it by the methods discussed in. Dt2 hooke's law is a principle of physics that states.
35 Likes, 6 Comments parthasarathi (physik.files) on Instagram
We want to find all the forces on each mass. Dt2 hooke's law is a principle of physics that states that the force f needed to extend or compress a spring by some distance. So we can solve it by the methods discussed in. F = ma = m = my00: Suppose a \(64\) lb weight stretches a spring \(6\).
[Solved] The following differential equation with init
We want to find all the forces on each mass. F = ma = m = my00: Suppose a \(64\) lb weight stretches a spring \(6\) inches in equilibrium and a dashpot provides a damping force of \(c\) lb for. We will do this by equating forces. Dt2 hooke's law is a principle of physics that states that the force.
Solved A massspring system is driven by a sinusoidal
We will do this by equating forces. So we can solve it by the methods discussed in. We want to find all the forces on each mass. Suppose a \(64\) lb weight stretches a spring \(6\) inches in equilibrium and a dashpot provides a damping force of \(c\) lb for. Dt2 hooke's law is a principle of physics that states.
Modeling differential equation systems merybirthday
Suppose a \(64\) lb weight stretches a spring \(6\) inches in equilibrium and a dashpot provides a damping force of \(c\) lb for. F = ma = m = my00: So we can solve it by the methods discussed in. We will do this by equating forces. Dt2 hooke's law is a principle of physics that states that the force.
physics Showing force acting on a spring as a differential equation
So we can solve it by the methods discussed in. We will do this by equating forces. We want to find all the forces on each mass. Dt2 hooke's law is a principle of physics that states that the force f needed to extend or compress a spring by some distance. F = ma = m = my00:
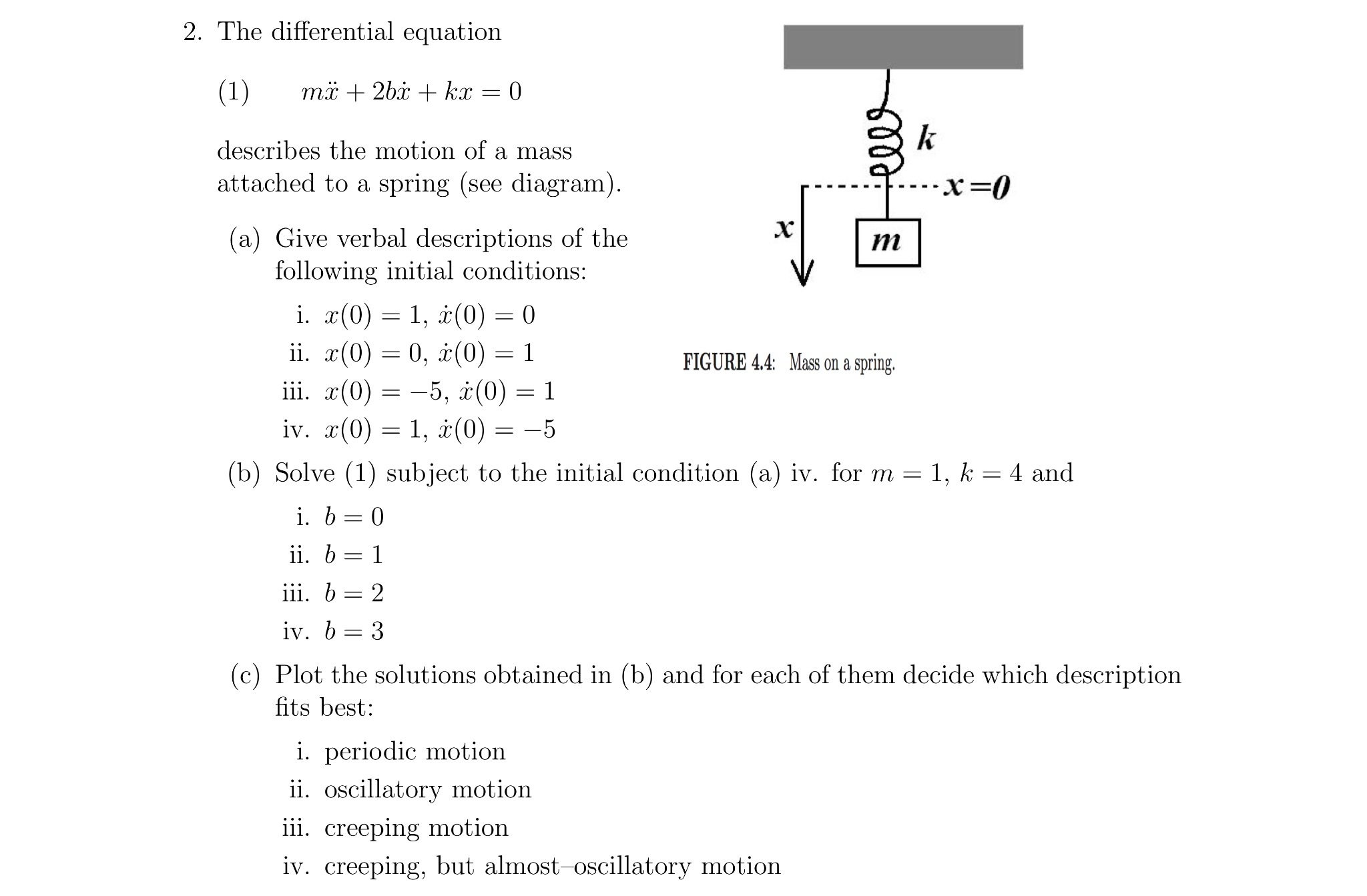
Solved 2. The Differential Equation Describes The Motion
Suppose a \(64\) lb weight stretches a spring \(6\) inches in equilibrium and a dashpot provides a damping force of \(c\) lb for. We want to find all the forces on each mass. F = ma = m = my00: Dt2 hooke's law is a principle of physics that states that the force f needed to extend or compress a.
Introduction of Differential Equation.pptx
Dt2 hooke's law is a principle of physics that states that the force f needed to extend or compress a spring by some distance. We want to find all the forces on each mass. Suppose a \(64\) lb weight stretches a spring \(6\) inches in equilibrium and a dashpot provides a damping force of \(c\) lb for. So we can.
We Will Do This By Equating Forces.
Dt2 hooke's law is a principle of physics that states that the force f needed to extend or compress a spring by some distance. So we can solve it by the methods discussed in. We want to find all the forces on each mass. Suppose a \(64\) lb weight stretches a spring \(6\) inches in equilibrium and a dashpot provides a damping force of \(c\) lb for.
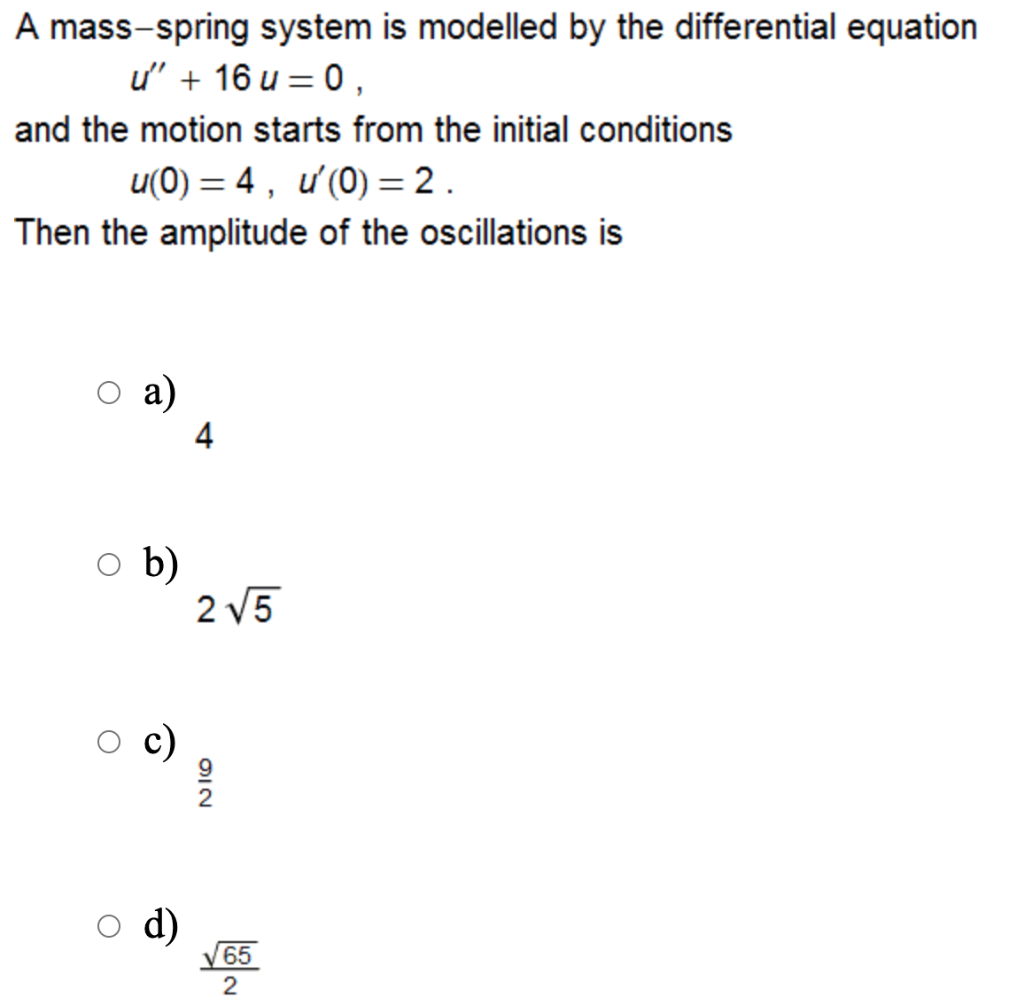


![[Solved] The following differential equation with init](https://media.cheggcdn.com/study/404/40491dae-5546-4779-879b-64bdce42d6ef/image.jpg)