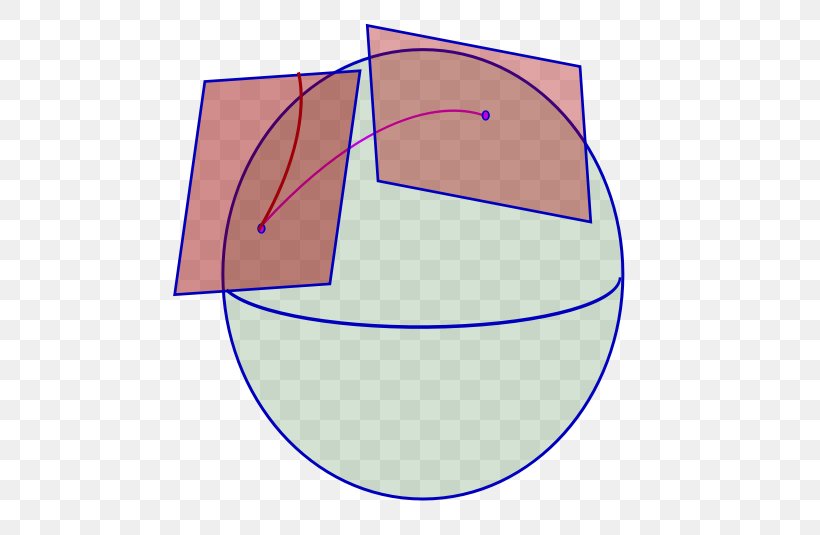
Why Tangent Space Of The Abelian Differential Is Cohomology - The vectors in tpk t p k are the vectors at p p which are tangent to k k. In this survey we are concerned with the cohomology of the moduli space of abelian. If v is a variety and v is a point in v , we write tv;v for the zariski tangent space to v at v. Since you multiply (wedge) differential forms together, cohomology becomes a ring. Ox) is the then the right derived functor of this global sections.
Ox) is the then the right derived functor of this global sections. The vectors in tpk t p k are the vectors at p p which are tangent to k k. Since you multiply (wedge) differential forms together, cohomology becomes a ring. In this survey we are concerned with the cohomology of the moduli space of abelian. If v is a variety and v is a point in v , we write tv;v for the zariski tangent space to v at v.
In this survey we are concerned with the cohomology of the moduli space of abelian. Since you multiply (wedge) differential forms together, cohomology becomes a ring. Ox) is the then the right derived functor of this global sections. If v is a variety and v is a point in v , we write tv;v for the zariski tangent space to v at v. The vectors in tpk t p k are the vectors at p p which are tangent to k k.
Tangent Space Affine Connection Differential Geometry, PNG, 519x535px
In this survey we are concerned with the cohomology of the moduli space of abelian. Ox) is the then the right derived functor of this global sections. If v is a variety and v is a point in v , we write tv;v for the zariski tangent space to v at v. The vectors in tpk t p k are.
differential geometry Why is this definition F_{*} between tangent
Since you multiply (wedge) differential forms together, cohomology becomes a ring. In this survey we are concerned with the cohomology of the moduli space of abelian. If v is a variety and v is a point in v , we write tv;v for the zariski tangent space to v at v. Ox) is the then the right derived functor of.
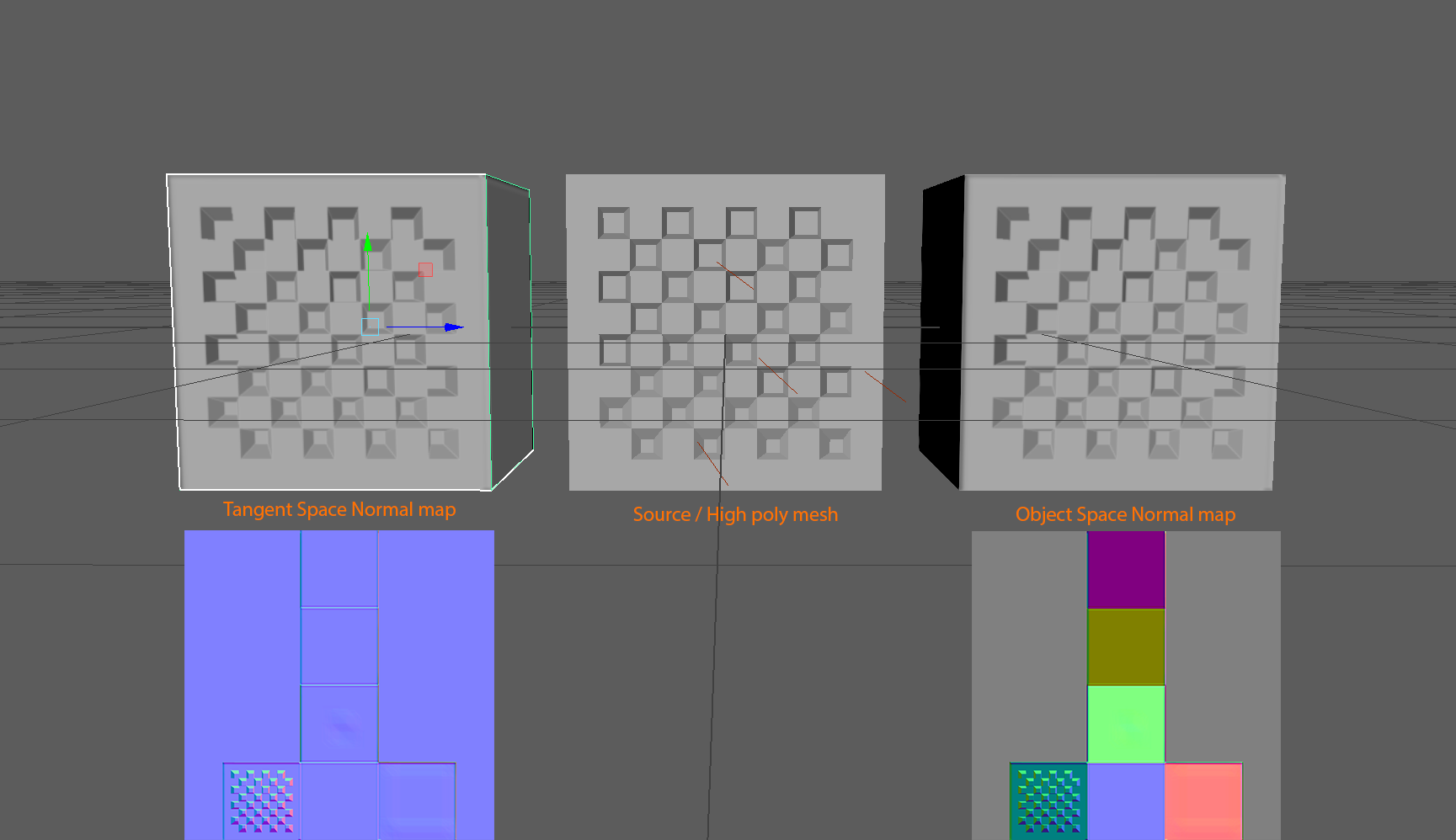
opengl Why Tangentspace normal map is suitable for deforming or
Since you multiply (wedge) differential forms together, cohomology becomes a ring. The vectors in tpk t p k are the vectors at p p which are tangent to k k. If v is a variety and v is a point in v , we write tv;v for the zariski tangent space to v at v. In this survey we are.
differential geometry Tangent Spaces & Definition of Differentiation
Ox) is the then the right derived functor of this global sections. Since you multiply (wedge) differential forms together, cohomology becomes a ring. If v is a variety and v is a point in v , we write tv;v for the zariski tangent space to v at v. In this survey we are concerned with the cohomology of the moduli.
Differential Cohomology Gallery — Untitled
In this survey we are concerned with the cohomology of the moduli space of abelian. Ox) is the then the right derived functor of this global sections. The vectors in tpk t p k are the vectors at p p which are tangent to k k. Since you multiply (wedge) differential forms together, cohomology becomes a ring. If v is.
(PDF) Cohomology of Differential Schemes
The vectors in tpk t p k are the vectors at p p which are tangent to k k. Since you multiply (wedge) differential forms together, cohomology becomes a ring. In this survey we are concerned with the cohomology of the moduli space of abelian. Ox) is the then the right derived functor of this global sections. If v is.
linear algebra Is the differential at a regular point, a vector space
If v is a variety and v is a point in v , we write tv;v for the zariski tangent space to v at v. In this survey we are concerned with the cohomology of the moduli space of abelian. The vectors in tpk t p k are the vectors at p p which are tangent to k k. Ox).
Differential of Surface Area Spherical Coordinates
If v is a variety and v is a point in v , we write tv;v for the zariski tangent space to v at v. In this survey we are concerned with the cohomology of the moduli space of abelian. The vectors in tpk t p k are the vectors at p p which are tangent to k k. Ox).
differential geometry Tangent spaces of SO(3) Mathematics Stack
If v is a variety and v is a point in v , we write tv;v for the zariski tangent space to v at v. In this survey we are concerned with the cohomology of the moduli space of abelian. Since you multiply (wedge) differential forms together, cohomology becomes a ring. The vectors in tpk t p k are the.
differential geometry Normal space and tangent space Mathematics
In this survey we are concerned with the cohomology of the moduli space of abelian. If v is a variety and v is a point in v , we write tv;v for the zariski tangent space to v at v. The vectors in tpk t p k are the vectors at p p which are tangent to k k. Since.
If V Is A Variety And V Is A Point In V , We Write Tv;V For The Zariski Tangent Space To V At V.
The vectors in tpk t p k are the vectors at p p which are tangent to k k. Since you multiply (wedge) differential forms together, cohomology becomes a ring. In this survey we are concerned with the cohomology of the moduli space of abelian. Ox) is the then the right derived functor of this global sections.