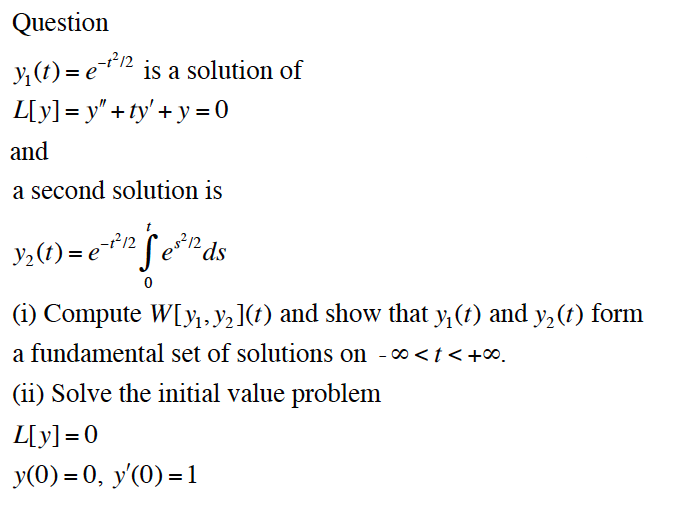
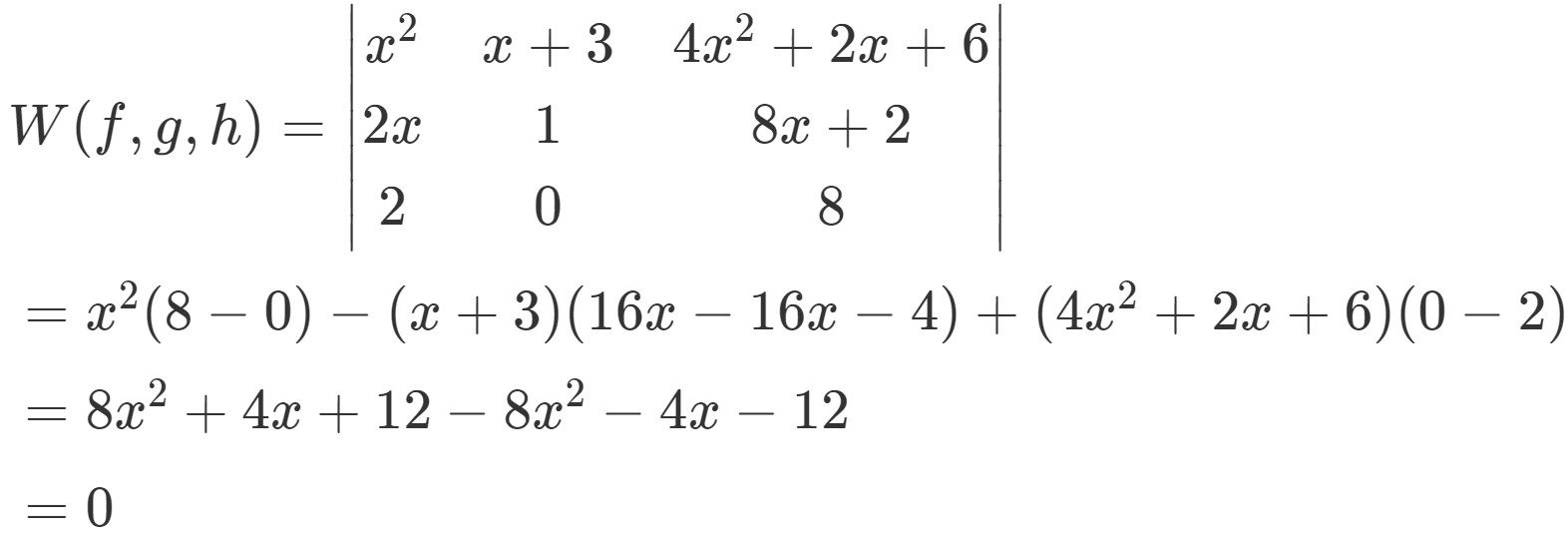Wronskian Of A Differential Equation - To demonstrate that the wronskian either vanishes for all values of x or it is never equal. We define fundamental sets of solutions and discuss how they can be used to. If the wronskian of f f and g g is tcos(t)+ sin(t) t cos (t) + sin (t), and if u = f − 3g u = f − 3 g and v. We’ll start by noticing that if the original equation is true, then if we differentiate.
We’ll start by noticing that if the original equation is true, then if we differentiate. If the wronskian of f f and g g is tcos(t)+ sin(t) t cos (t) + sin (t), and if u = f − 3g u = f − 3 g and v. To demonstrate that the wronskian either vanishes for all values of x or it is never equal. We define fundamental sets of solutions and discuss how they can be used to.
If the wronskian of f f and g g is tcos(t)+ sin(t) t cos (t) + sin (t), and if u = f − 3g u = f − 3 g and v. To demonstrate that the wronskian either vanishes for all values of x or it is never equal. We define fundamental sets of solutions and discuss how they can be used to. We’ll start by noticing that if the original equation is true, then if we differentiate.
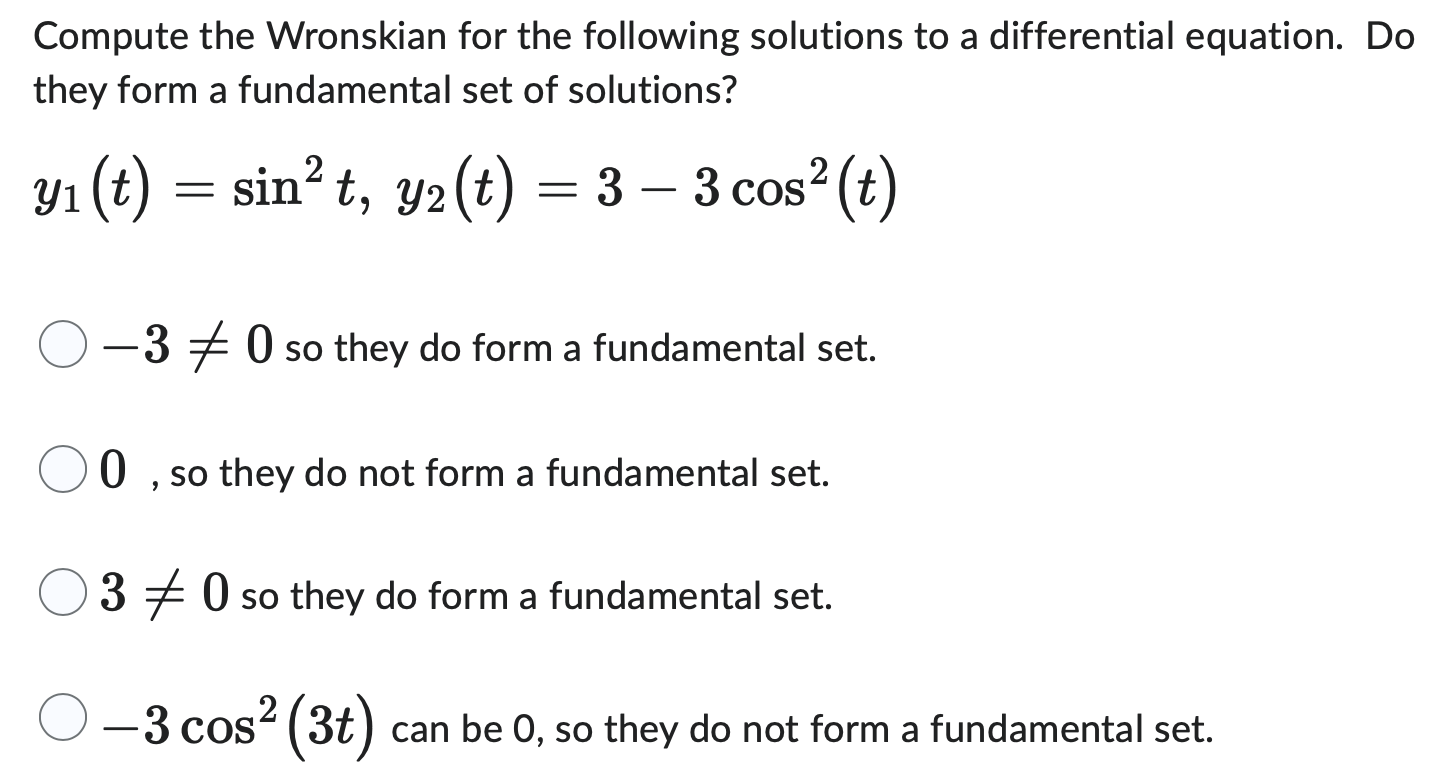
Solved Compute the Wronskian for the following solutions to
To demonstrate that the wronskian either vanishes for all values of x or it is never equal. We define fundamental sets of solutions and discuss how they can be used to. If the wronskian of f f and g g is tcos(t)+ sin(t) t cos (t) + sin (t), and if u = f − 3g u = f −.
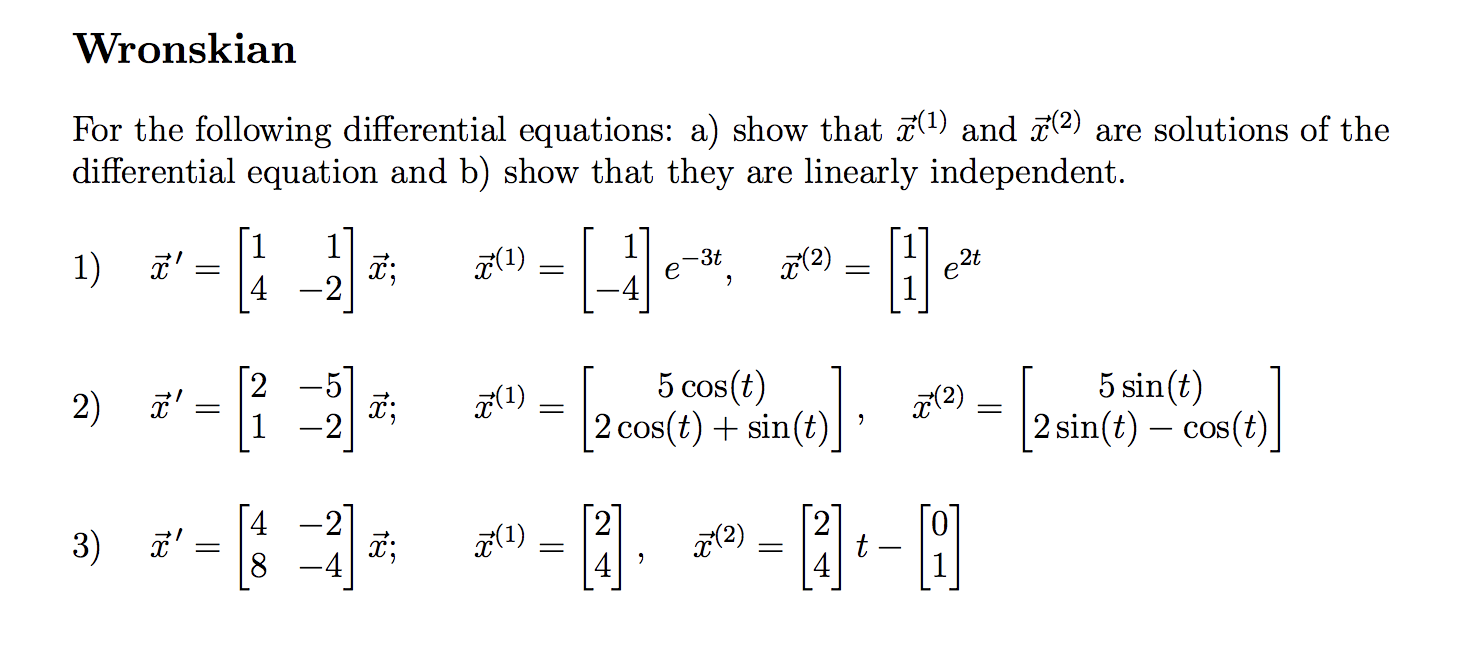
Solved Wronskian For the following differential equations
To demonstrate that the wronskian either vanishes for all values of x or it is never equal. If the wronskian of f f and g g is tcos(t)+ sin(t) t cos (t) + sin (t), and if u = f − 3g u = f − 3 g and v. We define fundamental sets of solutions and discuss how they.
SOLVEDThe Wronskian determinant (or simply, the Wronskian) of a linear
We’ll start by noticing that if the original equation is true, then if we differentiate. To demonstrate that the wronskian either vanishes for all values of x or it is never equal. We define fundamental sets of solutions and discuss how they can be used to. If the wronskian of f f and g g is tcos(t)+ sin(t) t cos.
Wronskian equation WAR Herb Zinser's Atomic Social Science Reports
We define fundamental sets of solutions and discuss how they can be used to. We’ll start by noticing that if the original equation is true, then if we differentiate. If the wronskian of f f and g g is tcos(t)+ sin(t) t cos (t) + sin (t), and if u = f − 3g u = f − 3 g.
Solving 2nd Order non homogeneous differential equation using Wronskian
We’ll start by noticing that if the original equation is true, then if we differentiate. We define fundamental sets of solutions and discuss how they can be used to. If the wronskian of f f and g g is tcos(t)+ sin(t) t cos (t) + sin (t), and if u = f − 3g u = f − 3 g.
Solved Second order differential equation Wronskian and
We define fundamental sets of solutions and discuss how they can be used to. We’ll start by noticing that if the original equation is true, then if we differentiate. If the wronskian of f f and g g is tcos(t)+ sin(t) t cos (t) + sin (t), and if u = f − 3g u = f − 3 g.
Solving 2nd Order non homogeneous differential equation using Wronskian
We’ll start by noticing that if the original equation is true, then if we differentiate. To demonstrate that the wronskian either vanishes for all values of x or it is never equal. We define fundamental sets of solutions and discuss how they can be used to. If the wronskian of f f and g g is tcos(t)+ sin(t) t cos.
Wronskian, differential, determinant
To demonstrate that the wronskian either vanishes for all values of x or it is never equal. If the wronskian of f f and g g is tcos(t)+ sin(t) t cos (t) + sin (t), and if u = f − 3g u = f − 3 g and v. We’ll start by noticing that if the original equation is.
Wronskian StudyPug
If the wronskian of f f and g g is tcos(t)+ sin(t) t cos (t) + sin (t), and if u = f − 3g u = f − 3 g and v. To demonstrate that the wronskian either vanishes for all values of x or it is never equal. We’ll start by noticing that if the original equation is.
⏩SOLVEDThe Wronskian formed from a solution set of the given… Numerade
We’ll start by noticing that if the original equation is true, then if we differentiate. We define fundamental sets of solutions and discuss how they can be used to. If the wronskian of f f and g g is tcos(t)+ sin(t) t cos (t) + sin (t), and if u = f − 3g u = f − 3 g.
To Demonstrate That The Wronskian Either Vanishes For All Values Of X Or It Is Never Equal.
We define fundamental sets of solutions and discuss how they can be used to. We’ll start by noticing that if the original equation is true, then if we differentiate. If the wronskian of f f and g g is tcos(t)+ sin(t) t cos (t) + sin (t), and if u = f − 3g u = f − 3 g and v.