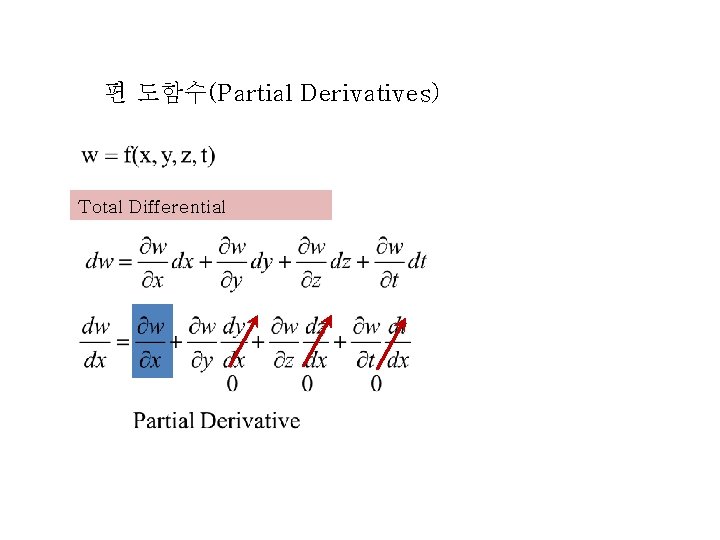
What Is A Total Differential - Total differentials can be generalized. Let \(dx\) and \(dy\) represent changes in \(x\) and. For a function f = f(x, y, z) whose partial derivatives exists, the total. Let \(z=f(x,y)\) be continuous on an open set \(s\). F(x + ∆x, y + ∆y) = f(x, y) + ∆z. If $x$ is secretly a function of $t$, then the notation $\frac{d}{dt}f(x,t)$ is called the total derivative and is an abbreviation for.
For a function f = f(x, y, z) whose partial derivatives exists, the total. If $x$ is secretly a function of $t$, then the notation $\frac{d}{dt}f(x,t)$ is called the total derivative and is an abbreviation for. Let \(z=f(x,y)\) be continuous on an open set \(s\). Total differentials can be generalized. Let \(dx\) and \(dy\) represent changes in \(x\) and. F(x + ∆x, y + ∆y) = f(x, y) + ∆z.
If $x$ is secretly a function of $t$, then the notation $\frac{d}{dt}f(x,t)$ is called the total derivative and is an abbreviation for. Total differentials can be generalized. For a function f = f(x, y, z) whose partial derivatives exists, the total. Let \(z=f(x,y)\) be continuous on an open set \(s\). Let \(dx\) and \(dy\) represent changes in \(x\) and. F(x + ∆x, y + ∆y) = f(x, y) + ∆z.
partial derivative Total differential definition help Mathematics
Let \(z=f(x,y)\) be continuous on an open set \(s\). F(x + ∆x, y + ∆y) = f(x, y) + ∆z. Let \(dx\) and \(dy\) represent changes in \(x\) and. For a function f = f(x, y, z) whose partial derivatives exists, the total. Total differentials can be generalized.
SOLUTION 3 6 the total differential Studypool
Let \(z=f(x,y)\) be continuous on an open set \(s\). F(x + ∆x, y + ∆y) = f(x, y) + ∆z. Total differentials can be generalized. Let \(dx\) and \(dy\) represent changes in \(x\) and. If $x$ is secretly a function of $t$, then the notation $\frac{d}{dt}f(x,t)$ is called the total derivative and is an abbreviation for.
Total Differential from Wolfram MathWorld
If $x$ is secretly a function of $t$, then the notation $\frac{d}{dt}f(x,t)$ is called the total derivative and is an abbreviation for. Let \(z=f(x,y)\) be continuous on an open set \(s\). Total differentials can be generalized. F(x + ∆x, y + ∆y) = f(x, y) + ∆z. Let \(dx\) and \(dy\) represent changes in \(x\) and.
Partial Differential Total Differential Total Differential of Function
For a function f = f(x, y, z) whose partial derivatives exists, the total. If $x$ is secretly a function of $t$, then the notation $\frac{d}{dt}f(x,t)$ is called the total derivative and is an abbreviation for. Let \(dx\) and \(dy\) represent changes in \(x\) and. F(x + ∆x, y + ∆y) = f(x, y) + ∆z. Let \(z=f(x,y)\) be continuous.
3.6 The Total Differential PDF
For a function f = f(x, y, z) whose partial derivatives exists, the total. If $x$ is secretly a function of $t$, then the notation $\frac{d}{dt}f(x,t)$ is called the total derivative and is an abbreviation for. Total differentials can be generalized. Let \(z=f(x,y)\) be continuous on an open set \(s\). F(x + ∆x, y + ∆y) = f(x, y) +.
Exact differential equation Alchetron, the free social encyclopedia
If $x$ is secretly a function of $t$, then the notation $\frac{d}{dt}f(x,t)$ is called the total derivative and is an abbreviation for. For a function f = f(x, y, z) whose partial derivatives exists, the total. Let \(dx\) and \(dy\) represent changes in \(x\) and. F(x + ∆x, y + ∆y) = f(x, y) + ∆z. Total differentials can be.
SOLUTION 3 6 the total differential Studypool
Let \(dx\) and \(dy\) represent changes in \(x\) and. For a function f = f(x, y, z) whose partial derivatives exists, the total. Total differentials can be generalized. Let \(z=f(x,y)\) be continuous on an open set \(s\). F(x + ∆x, y + ∆y) = f(x, y) + ∆z.
calculus Visualizing the total differential Mathematics Stack Exchange
If $x$ is secretly a function of $t$, then the notation $\frac{d}{dt}f(x,t)$ is called the total derivative and is an abbreviation for. Total differentials can be generalized. For a function f = f(x, y, z) whose partial derivatives exists, the total. Let \(dx\) and \(dy\) represent changes in \(x\) and. F(x + ∆x, y + ∆y) = f(x, y) +.
Partial Differential Total Differential Total Differential of Function
For a function f = f(x, y, z) whose partial derivatives exists, the total. F(x + ∆x, y + ∆y) = f(x, y) + ∆z. If $x$ is secretly a function of $t$, then the notation $\frac{d}{dt}f(x,t)$ is called the total derivative and is an abbreviation for. Let \(dx\) and \(dy\) represent changes in \(x\) and. Let \(z=f(x,y)\) be continuous.
Is this legal in total differential? ResearchGate
Total differentials can be generalized. For a function f = f(x, y, z) whose partial derivatives exists, the total. If $x$ is secretly a function of $t$, then the notation $\frac{d}{dt}f(x,t)$ is called the total derivative and is an abbreviation for. Let \(z=f(x,y)\) be continuous on an open set \(s\). F(x + ∆x, y + ∆y) = f(x, y) +.
Total Differentials Can Be Generalized.
Let \(z=f(x,y)\) be continuous on an open set \(s\). If $x$ is secretly a function of $t$, then the notation $\frac{d}{dt}f(x,t)$ is called the total derivative and is an abbreviation for. F(x + ∆x, y + ∆y) = f(x, y) + ∆z. For a function f = f(x, y, z) whose partial derivatives exists, the total.