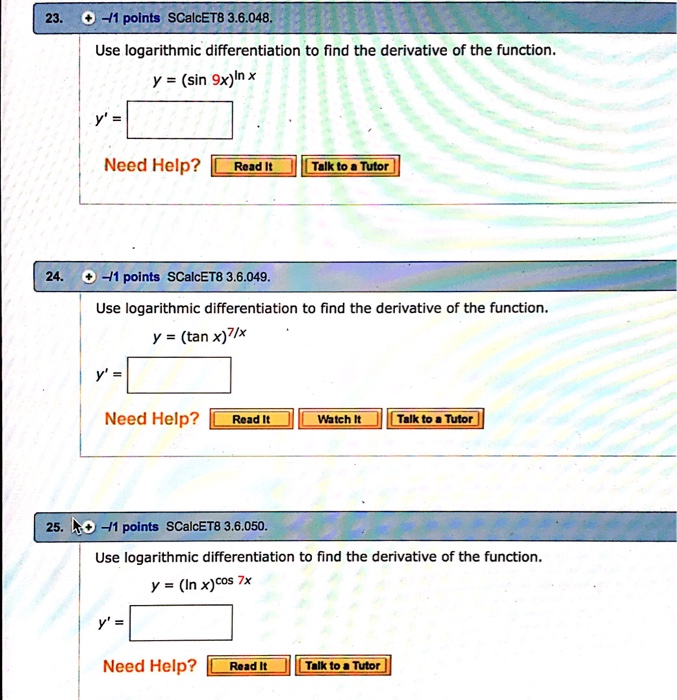
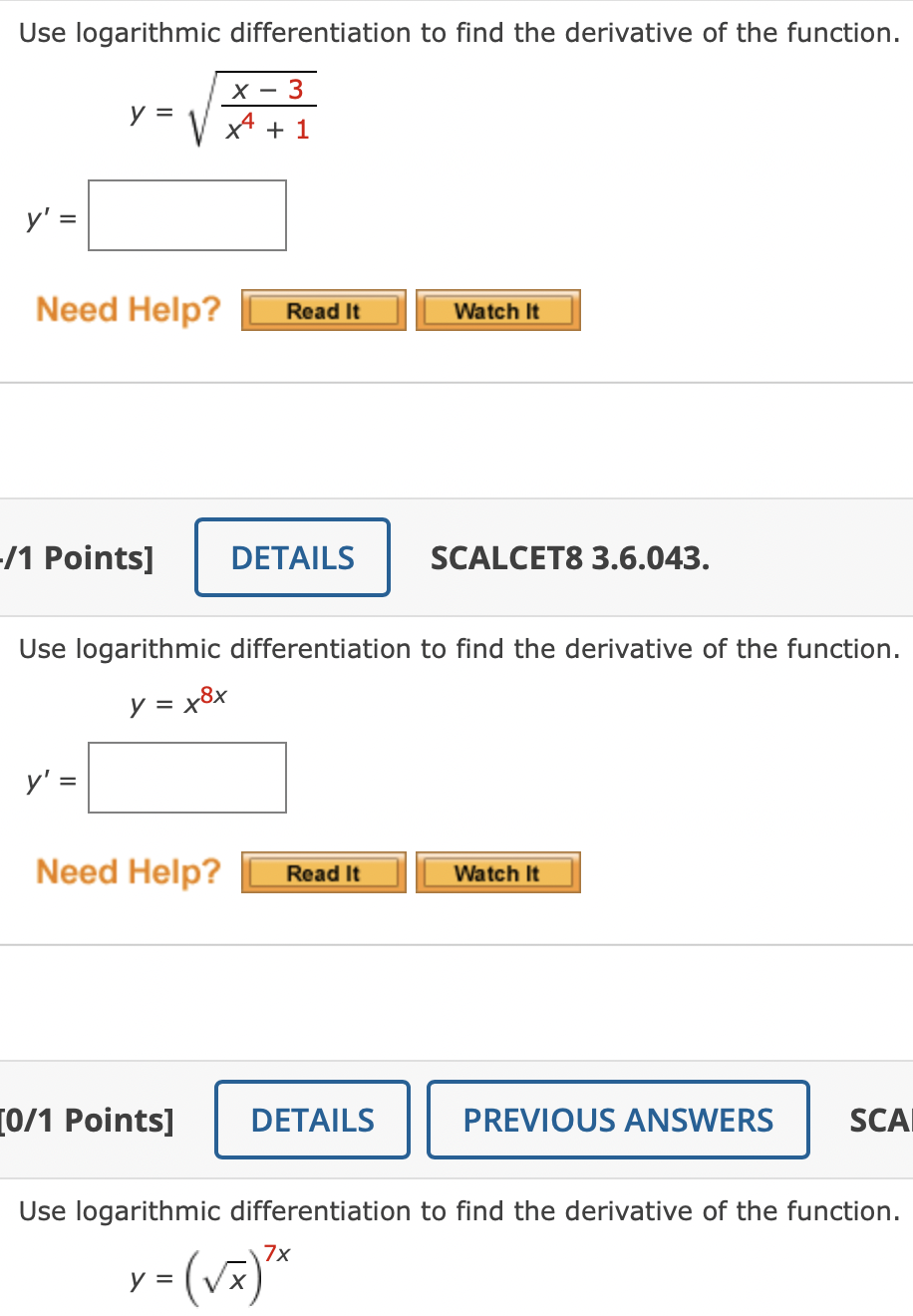
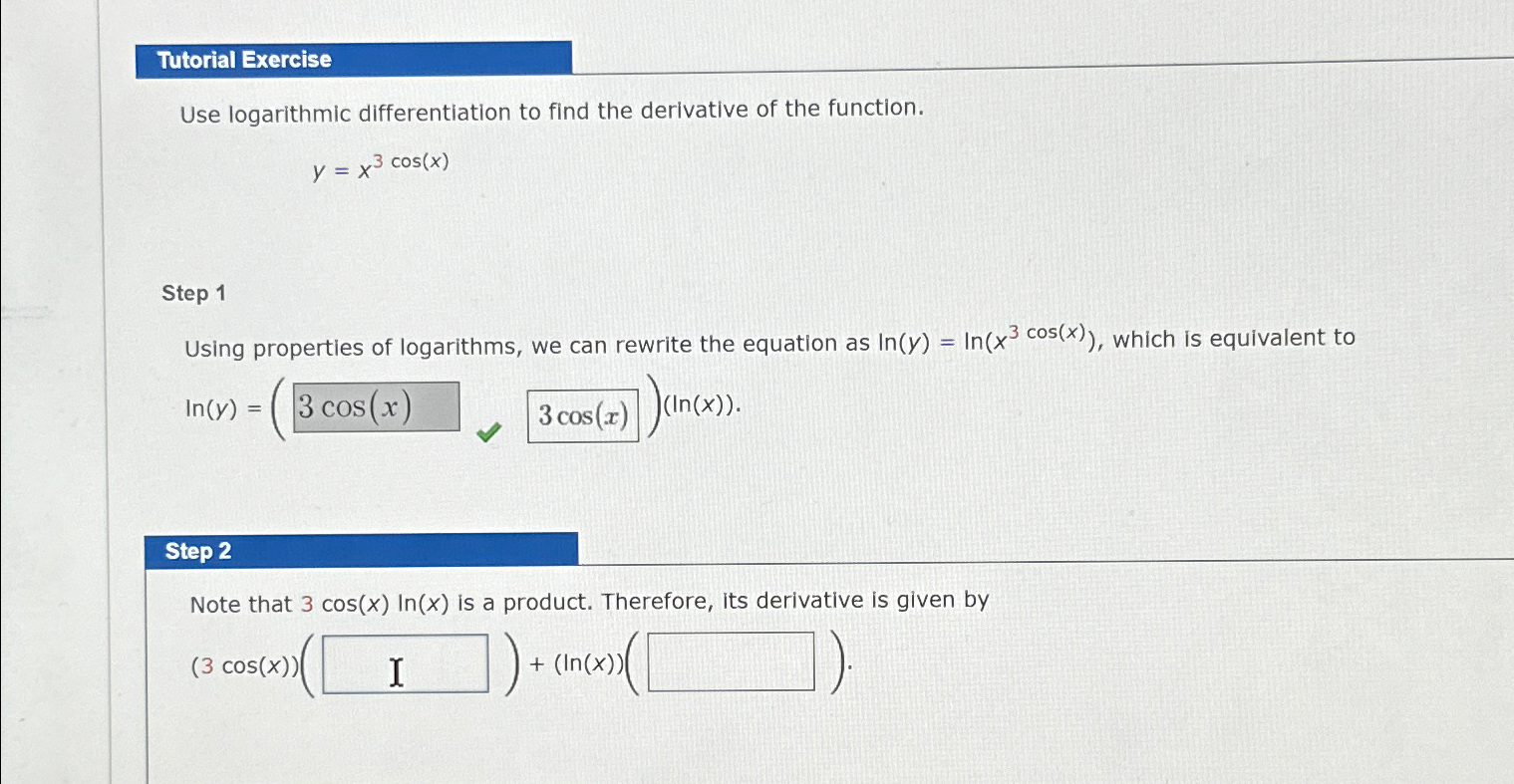
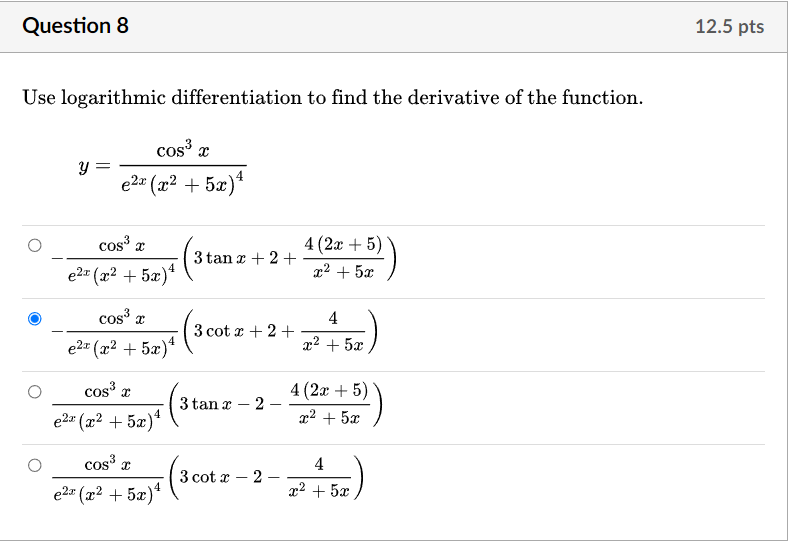
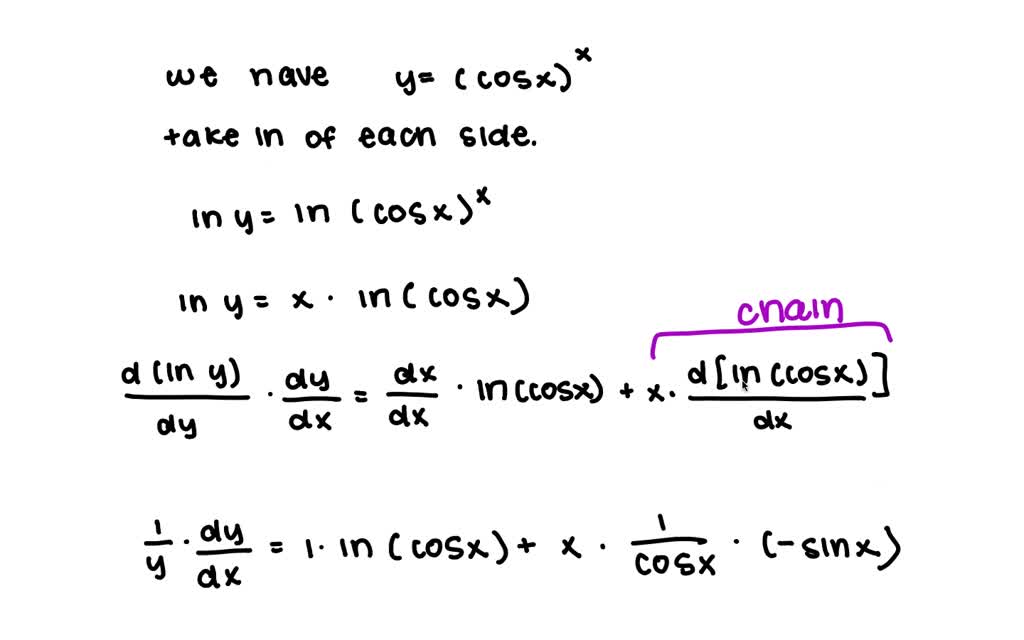Use Logarithmic Differentiation To Find The Derivative Of The Function - Expand ln((sin(x))cos (x)) by moving cos(x) outside the logarithm. Take the natural log of both sides. Taking the derivatives of some complicated functions can be simplified by using logarithms. Let y = f(x), take the natural logarithm of both sides ln(y) = ln(f(x)). Use log properties to simplify the equations. Differentiate the expression using the chain rule, keeping in mind that y y is a function of x x. Logarithmic differentiation is used to find the differentiation of some complicated functions, using logarithm. Learn its formulas and method. Logarithmic differentiation allows us to differentiate functions of the form \(y=g(x)^{f(x)}\) or very complex functions by. Just follow the five steps below:
Logarithmic differentiation is used to find the differentiation of some complicated functions, using logarithm. Learn its formulas and method. Let y = f(x), take the natural logarithm of both sides ln(y) = ln(f(x)). Use log properties to simplify the equations. Take the natural log of both sides. Differentiate the expression using the chain rule, keeping in mind that y y is a function of x x. Isolate y' y ′ and substitute the. Just follow the five steps below: Taking the derivatives of some complicated functions can be simplified by using logarithms. Expand ln((sin(x))cos (x)) by moving cos(x) outside the logarithm.
Just follow the five steps below: Let y = f(x), take the natural logarithm of both sides ln(y) = ln(f(x)). Use log properties to simplify the equations. Take the natural log of both sides. Taking the derivatives of some complicated functions can be simplified by using logarithms. Logarithmic differentiation allows us to differentiate functions of the form \(y=g(x)^{f(x)}\) or very complex functions by. Differentiate the expression using the chain rule, keeping in mind that y y is a function of x x. Logarithmic differentiation is used to find the differentiation of some complicated functions, using logarithm. Expand ln((sin(x))cos (x)) by moving cos(x) outside the logarithm. Isolate y' y ′ and substitute the.
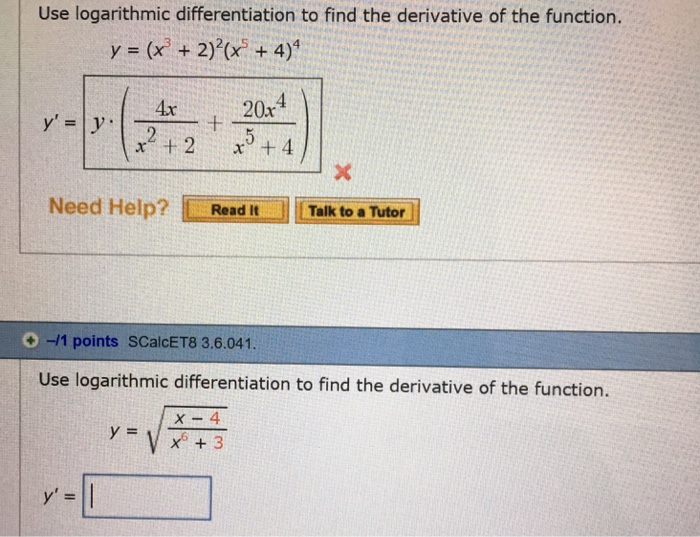
Solved Use logarithmic differentiation to find the
Logarithmic differentiation allows us to differentiate functions of the form \(y=g(x)^{f(x)}\) or very complex functions by. Learn its formulas and method. Let y = f(x), take the natural logarithm of both sides ln(y) = ln(f(x)). Expand ln((sin(x))cos (x)) by moving cos(x) outside the logarithm. Logarithmic differentiation is used to find the differentiation of some complicated functions, using logarithm.
Solved Use logarithmic differentiation to find the
Use log properties to simplify the equations. Taking the derivatives of some complicated functions can be simplified by using logarithms. Logarithmic differentiation allows us to differentiate functions of the form \(y=g(x)^{f(x)}\) or very complex functions by. Take the natural log of both sides. Just follow the five steps below:
Solved Use logarithmic differentiation to find the
Differentiate the expression using the chain rule, keeping in mind that y y is a function of x x. Take the natural log of both sides. Logarithmic differentiation is used to find the differentiation of some complicated functions, using logarithm. Use log properties to simplify the equations. Just follow the five steps below:
Solved Tutorial ExerciseUse logarithmic differentiation to
Let y = f(x), take the natural logarithm of both sides ln(y) = ln(f(x)). Differentiate the expression using the chain rule, keeping in mind that y y is a function of x x. Expand ln((sin(x))cos (x)) by moving cos(x) outside the logarithm. Logarithmic differentiation allows us to differentiate functions of the form \(y=g(x)^{f(x)}\) or very complex functions by. Learn its.
Solved Ex. 3 Use logarithmic differentiation to find the
Use log properties to simplify the equations. Logarithmic differentiation allows us to differentiate functions of the form \(y=g(x)^{f(x)}\) or very complex functions by. Logarithmic differentiation is used to find the differentiation of some complicated functions, using logarithm. Taking the derivatives of some complicated functions can be simplified by using logarithms. Let y = f(x), take the natural logarithm of both.
Solved 18Use logarithmic differentiation to find the
Logarithmic differentiation allows us to differentiate functions of the form \(y=g(x)^{f(x)}\) or very complex functions by. Take the natural log of both sides. Use log properties to simplify the equations. Let y = f(x), take the natural logarithm of both sides ln(y) = ln(f(x)). Differentiate the expression using the chain rule, keeping in mind that y y is a function.
Solved Use logarithmic differentiation to find the
Isolate y' y ′ and substitute the. Logarithmic differentiation allows us to differentiate functions of the form \(y=g(x)^{f(x)}\) or very complex functions by. Expand ln((sin(x))cos (x)) by moving cos(x) outside the logarithm. Logarithmic differentiation is used to find the differentiation of some complicated functions, using logarithm. Just follow the five steps below:
Solved use logarithmic differentiation to find the
Taking the derivatives of some complicated functions can be simplified by using logarithms. Differentiate the expression using the chain rule, keeping in mind that y y is a function of x x. Isolate y' y ′ and substitute the. Logarithmic differentiation is used to find the differentiation of some complicated functions, using logarithm. Logarithmic differentiation allows us to differentiate functions.
Solved Use logarithmic differentiation to find the
Just follow the five steps below: Use log properties to simplify the equations. Let y = f(x), take the natural logarithm of both sides ln(y) = ln(f(x)). Logarithmic differentiation allows us to differentiate functions of the form \(y=g(x)^{f(x)}\) or very complex functions by. Learn its formulas and method.
SOLVED Use logarithmic differentiation to find the derivative of the
Use log properties to simplify the equations. Logarithmic differentiation allows us to differentiate functions of the form \(y=g(x)^{f(x)}\) or very complex functions by. Just follow the five steps below: Learn its formulas and method. Logarithmic differentiation is used to find the differentiation of some complicated functions, using logarithm.
Differentiate The Expression Using The Chain Rule, Keeping In Mind That Y Y Is A Function Of X X.
Just follow the five steps below: Logarithmic differentiation is used to find the differentiation of some complicated functions, using logarithm. Let y = f(x), take the natural logarithm of both sides ln(y) = ln(f(x)). Take the natural log of both sides.
Learn Its Formulas And Method.
Expand ln((sin(x))cos (x)) by moving cos(x) outside the logarithm. Use log properties to simplify the equations. Isolate y' y ′ and substitute the. Logarithmic differentiation allows us to differentiate functions of the form \(y=g(x)^{f(x)}\) or very complex functions by.