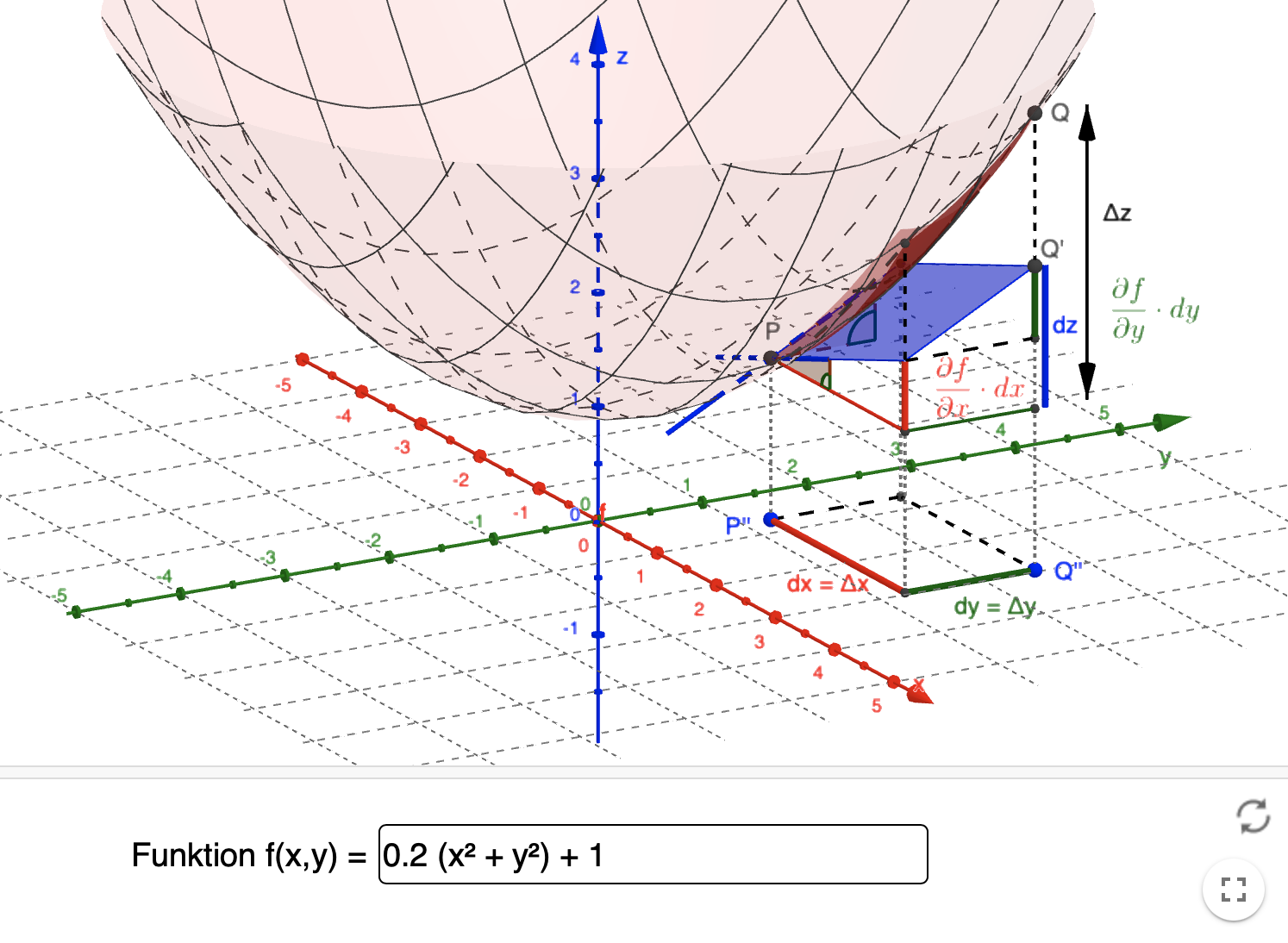
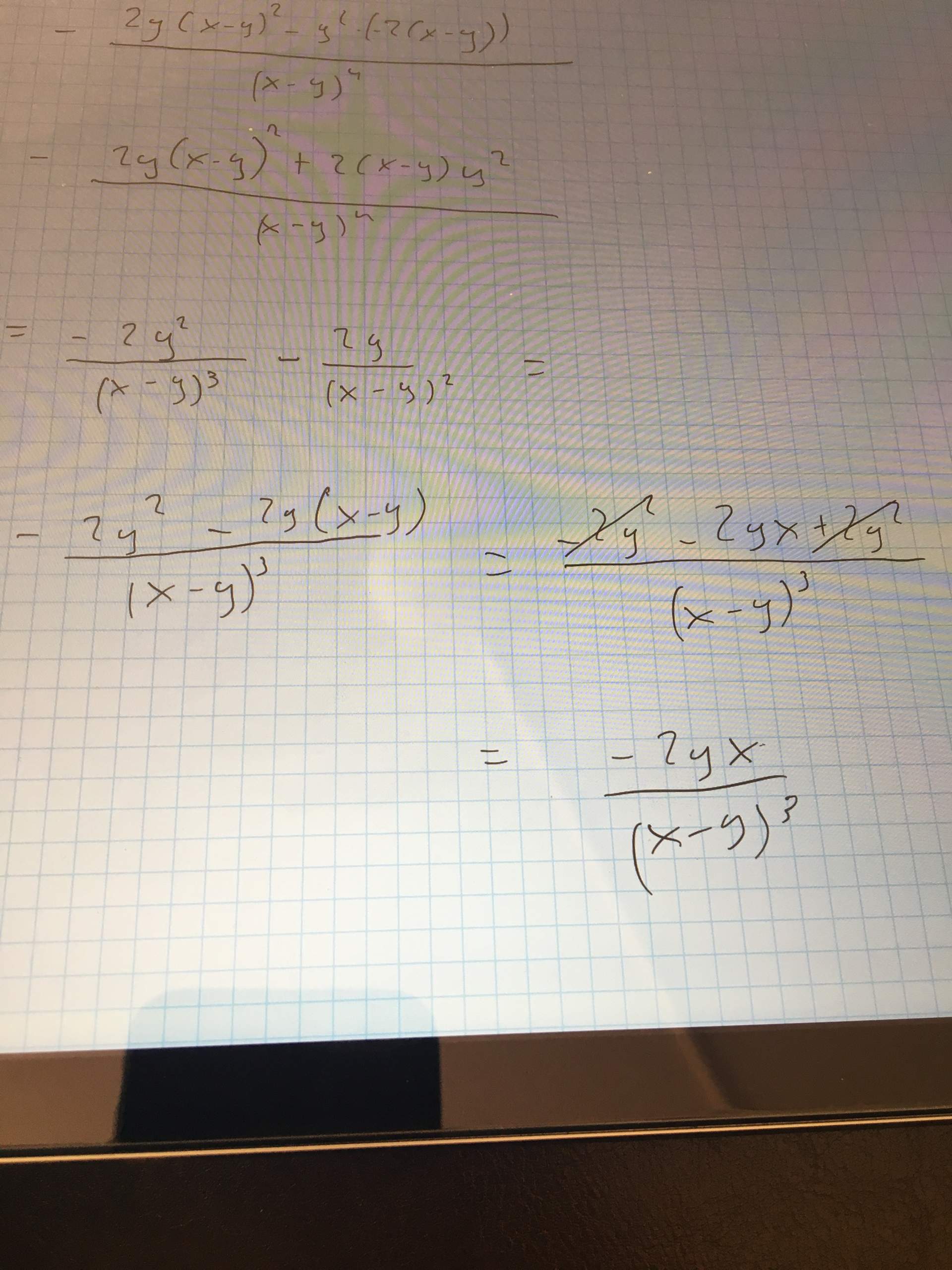Totales Differential - Let \(dx\) and \(dy\) represent changes in \(x\) and. Total differentials can be generalized. For a function f = f(x, y, z) whose partial derivatives exists, the total. F(x + ∆x, y + ∆y) = f(x, y) + ∆z. Let \(z=f(x,y)\) be continuous on an open set \(s\). The former part of δ x is called the (total) differential or the exact differential of the function f in the point (x, y, z) and it is denoted.
Let \(z=f(x,y)\) be continuous on an open set \(s\). Total differentials can be generalized. Let \(dx\) and \(dy\) represent changes in \(x\) and. F(x + ∆x, y + ∆y) = f(x, y) + ∆z. For a function f = f(x, y, z) whose partial derivatives exists, the total. The former part of δ x is called the (total) differential or the exact differential of the function f in the point (x, y, z) and it is denoted.
Let \(dx\) and \(dy\) represent changes in \(x\) and. The former part of δ x is called the (total) differential or the exact differential of the function f in the point (x, y, z) and it is denoted. F(x + ∆x, y + ∆y) = f(x, y) + ∆z. Let \(z=f(x,y)\) be continuous on an open set \(s\). For a function f = f(x, y, z) whose partial derivatives exists, the total. Total differentials can be generalized.
partial derivative Total differential definition help Mathematics
Total differentials can be generalized. The former part of δ x is called the (total) differential or the exact differential of the function f in the point (x, y, z) and it is denoted. F(x + ∆x, y + ∆y) = f(x, y) + ∆z. For a function f = f(x, y, z) whose partial derivatives exists, the total..
Totales Differential Mathelounge
For a function f = f(x, y, z) whose partial derivatives exists, the total. Total differentials can be generalized. Let \(z=f(x,y)\) be continuous on an open set \(s\). The former part of δ x is called the (total) differential or the exact differential of the function f in the point (x, y, z) and it is denoted. F(x +.
Totales Differential
The former part of δ x is called the (total) differential or the exact differential of the function f in the point (x, y, z) and it is denoted. F(x + ∆x, y + ∆y) = f(x, y) + ∆z. Let \(z=f(x,y)\) be continuous on an open set \(s\). Total differentials can be generalized. For a function f =.
Totales Differential Mathelounge
The former part of δ x is called the (total) differential or the exact differential of the function f in the point (x, y, z) and it is denoted. Let \(dx\) and \(dy\) represent changes in \(x\) and. F(x + ∆x, y + ∆y) = f(x, y) + ∆z. Total differentials can be generalized. For a function f =.
Totales Differential Aufgaben
F(x + ∆x, y + ∆y) = f(x, y) + ∆z. Total differentials can be generalized. The former part of δ x is called the (total) differential or the exact differential of the function f in the point (x, y, z) and it is denoted. Let \(dx\) and \(dy\) represent changes in \(x\) and. Let \(z=f(x,y)\) be continuous on.
calculus Visualizing the total differential Mathematics Stack Exchange
The former part of δ x is called the (total) differential or the exact differential of the function f in the point (x, y, z) and it is denoted. Let \(z=f(x,y)\) be continuous on an open set \(s\). F(x + ∆x, y + ∆y) = f(x, y) + ∆z. Total differentials can be generalized. For a function f =.
Totales Differential MathFlix
Let \(z=f(x,y)\) be continuous on an open set \(s\). The former part of δ x is called the (total) differential or the exact differential of the function f in the point (x, y, z) and it is denoted. For a function f = f(x, y, z) whose partial derivatives exists, the total. Total differentials can be generalized. F(x +.
Totales Differential berechnen und prüfen? (Schule, Mathe, Mathematik)
F(x + ∆x, y + ∆y) = f(x, y) + ∆z. Total differentials can be generalized. For a function f = f(x, y, z) whose partial derivatives exists, the total. Let \(dx\) and \(dy\) represent changes in \(x\) and. The former part of δ x is called the (total) differential or the exact differential of the function f in.
Total differential as estimation error, partial differentials as
Total differentials can be generalized. Let \(z=f(x,y)\) be continuous on an open set \(s\). F(x + ∆x, y + ∆y) = f(x, y) + ∆z. For a function f = f(x, y, z) whose partial derivatives exists, the total. Let \(dx\) and \(dy\) represent changes in \(x\) and.
Lösungen von Totalen Differential Aufgaben Tipps & Tricks zur
F(x + ∆x, y + ∆y) = f(x, y) + ∆z. Let \(dx\) and \(dy\) represent changes in \(x\) and. For a function f = f(x, y, z) whose partial derivatives exists, the total. The former part of δ x is called the (total) differential or the exact differential of the function f in the point (x, y, z).
The Former Part Of Δ X Is Called The (Total) Differential Or The Exact Differential Of The Function F In The Point (X, Y, Z) And It Is Denoted.
Let \(dx\) and \(dy\) represent changes in \(x\) and. Total differentials can be generalized. F(x + ∆x, y + ∆y) = f(x, y) + ∆z. For a function f = f(x, y, z) whose partial derivatives exists, the total.