Solving Differential Equations Using Laplace Transform - In this section we will examine how to use laplace transforms to solve ivp’s. The laplace transform is an integral transform that is widely used to solve linear differential equations with constant. In this section we employ the laplace transform to solve constant coefficient ordinary differential equations. Learn to solve differential equations using laplace transforms. Simplify complex problems with this powerful technique. We will also give brief overview on using laplace transforms to solve nonconstant coefficient differential equations. Applying the laplace transform to the ivp y00+ ay0+ by = f(t) with initial conditions y(0) =. In particular we shall consider initial. The examples in this section are restricted to. The laplace transform method from sections 5.2 and 5.3:
Learn to solve differential equations using laplace transforms. The laplace transform is an integral transform that is widely used to solve linear differential equations with constant. In this section we employ the laplace transform to solve constant coefficient ordinary differential equations. In this section we will examine how to use laplace transforms to solve ivp’s. Simplify complex problems with this powerful technique. The examples in this section are restricted to. In particular we shall consider initial. The laplace transform method from sections 5.2 and 5.3: We will also give brief overview on using laplace transforms to solve nonconstant coefficient differential equations. Applying the laplace transform to the ivp y00+ ay0+ by = f(t) with initial conditions y(0) =.
Applying the laplace transform to the ivp y00+ ay0+ by = f(t) with initial conditions y(0) =. The laplace transform method from sections 5.2 and 5.3: The laplace transform is an integral transform that is widely used to solve linear differential equations with constant. In particular we shall consider initial. In this section we will examine how to use laplace transforms to solve ivp’s. Simplify complex problems with this powerful technique. The examples in this section are restricted to. Learn to solve differential equations using laplace transforms. In this section we employ the laplace transform to solve constant coefficient ordinary differential equations. We will also give brief overview on using laplace transforms to solve nonconstant coefficient differential equations.
SOLUTION Solving simultaneous linear differential equations by using
In this section we employ the laplace transform to solve constant coefficient ordinary differential equations. Simplify complex problems with this powerful technique. The laplace transform method from sections 5.2 and 5.3: Applying the laplace transform to the ivp y00+ ay0+ by = f(t) with initial conditions y(0) =. In this section we will examine how to use laplace transforms to.
[Solved] Solve the following differential equations using Laplace
We will also give brief overview on using laplace transforms to solve nonconstant coefficient differential equations. The laplace transform is an integral transform that is widely used to solve linear differential equations with constant. Learn to solve differential equations using laplace transforms. The laplace transform method from sections 5.2 and 5.3: Applying the laplace transform to the ivp y00+ ay0+.
[Solved] Solve the following differential equations using Laplace
We will also give brief overview on using laplace transforms to solve nonconstant coefficient differential equations. The examples in this section are restricted to. In this section we employ the laplace transform to solve constant coefficient ordinary differential equations. Simplify complex problems with this powerful technique. The laplace transform is an integral transform that is widely used to solve linear.
PDF Télécharger solving differential equations using laplace transform
The examples in this section are restricted to. We will also give brief overview on using laplace transforms to solve nonconstant coefficient differential equations. The laplace transform is an integral transform that is widely used to solve linear differential equations with constant. Learn to solve differential equations using laplace transforms. In this section we employ the laplace transform to solve.
Solving Differential Equations Using Laplace Transform Solutions dummies
In this section we will examine how to use laplace transforms to solve ivp’s. Simplify complex problems with this powerful technique. The laplace transform method from sections 5.2 and 5.3: In particular we shall consider initial. The laplace transform is an integral transform that is widely used to solve linear differential equations with constant.
SOLUTION Solving Differential Equations using Laplace Transforms
Simplify complex problems with this powerful technique. We will also give brief overview on using laplace transforms to solve nonconstant coefficient differential equations. The examples in this section are restricted to. Applying the laplace transform to the ivp y00+ ay0+ by = f(t) with initial conditions y(0) =. In particular we shall consider initial.
[Solved] Solve the following differential equations using Laplace
Applying the laplace transform to the ivp y00+ ay0+ by = f(t) with initial conditions y(0) =. In this section we will examine how to use laplace transforms to solve ivp’s. In this section we employ the laplace transform to solve constant coefficient ordinary differential equations. The examples in this section are restricted to. Learn to solve differential equations using.
SOLUTION Solving simultaneous linear differential equations by using
In this section we will examine how to use laplace transforms to solve ivp’s. In particular we shall consider initial. The laplace transform method from sections 5.2 and 5.3: We will also give brief overview on using laplace transforms to solve nonconstant coefficient differential equations. Learn to solve differential equations using laplace transforms.
Daily Chaos Laplace Transform Solving Differential Equation
In this section we will examine how to use laplace transforms to solve ivp’s. In particular we shall consider initial. In this section we employ the laplace transform to solve constant coefficient ordinary differential equations. Learn to solve differential equations using laplace transforms. The laplace transform is an integral transform that is widely used to solve linear differential equations with.
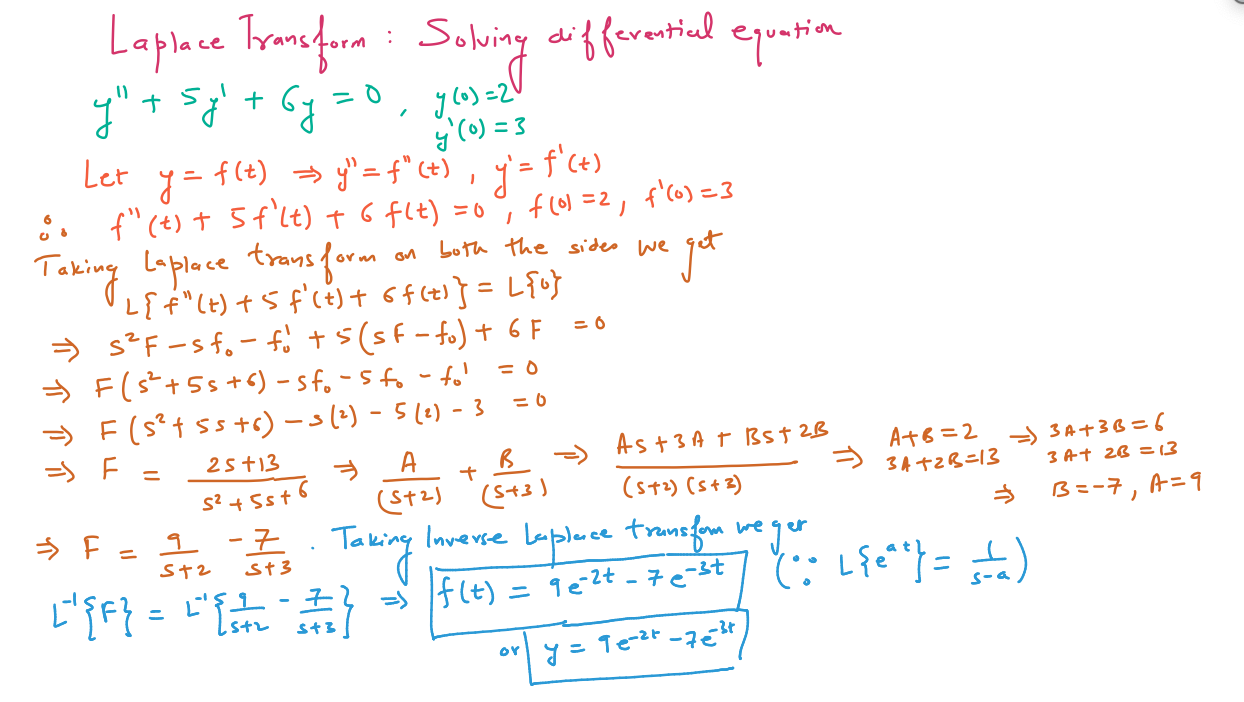
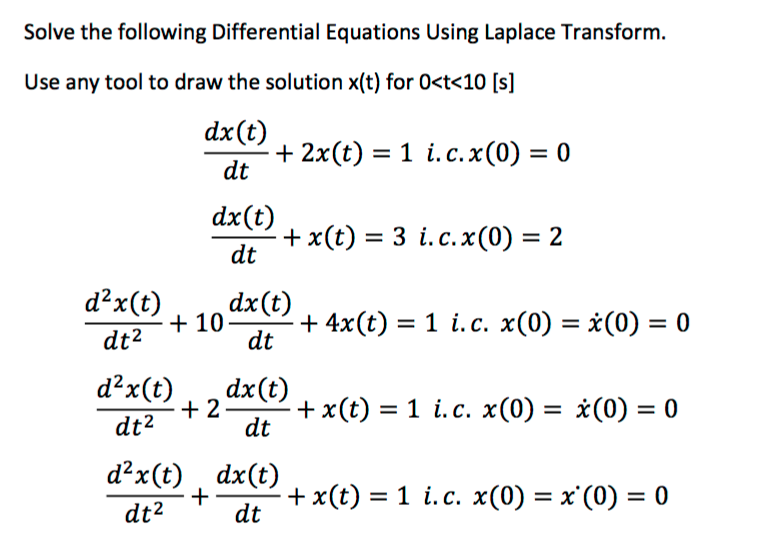
Solved Solve the following Differential Equations Using
The examples in this section are restricted to. Applying the laplace transform to the ivp y00+ ay0+ by = f(t) with initial conditions y(0) =. The laplace transform method from sections 5.2 and 5.3: Simplify complex problems with this powerful technique. The laplace transform is an integral transform that is widely used to solve linear differential equations with constant.
Simplify Complex Problems With This Powerful Technique.
The laplace transform method from sections 5.2 and 5.3: The laplace transform is an integral transform that is widely used to solve linear differential equations with constant. In this section we will examine how to use laplace transforms to solve ivp’s. Applying the laplace transform to the ivp y00+ ay0+ by = f(t) with initial conditions y(0) =.
The Examples In This Section Are Restricted To.
In this section we employ the laplace transform to solve constant coefficient ordinary differential equations. In particular we shall consider initial. Learn to solve differential equations using laplace transforms. We will also give brief overview on using laplace transforms to solve nonconstant coefficient differential equations.