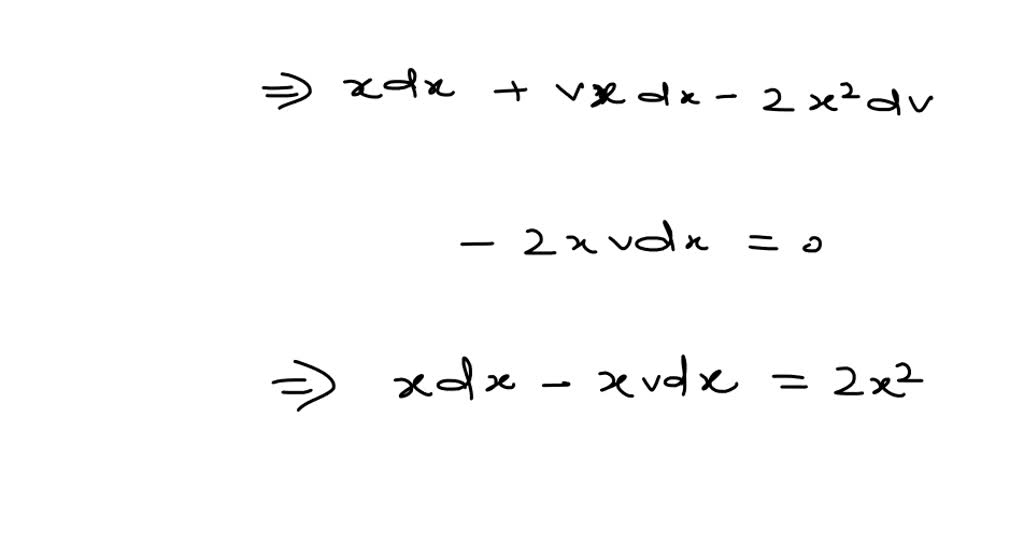Solving A Homogeneous Differential Equation - In this section we will extend the ideas behind solving 2nd order, linear, homogeneous differential equations to higher. The general form of a homogeneous differential equation is f(x, y).dy + g(x, y).dx = 0. Learn what a homogeneous differential equation is and how to solve it using the substitution method. A homogeneous differential equation can often be solved by making the substitution $v(x)=\dfrac{y}{x}$, where $v=v(x)$ is a. Using y = vx and dy dx = v + x dv dx we can solve the differential equation. Let us learn more about the homogeneous differential. See the definition, steps and solved examples. An example will show how it is all done: A differential equation of the form dy/dx = f (x, y)/ g (x, y) is called homogeneous differential equation if f (x, y) and g(x, y) are homogeneous.
Let us learn more about the homogeneous differential. The general form of a homogeneous differential equation is f(x, y).dy + g(x, y).dx = 0. An example will show how it is all done: A differential equation of the form dy/dx = f (x, y)/ g (x, y) is called homogeneous differential equation if f (x, y) and g(x, y) are homogeneous. Using y = vx and dy dx = v + x dv dx we can solve the differential equation. In this section we will extend the ideas behind solving 2nd order, linear, homogeneous differential equations to higher. A homogeneous differential equation can often be solved by making the substitution $v(x)=\dfrac{y}{x}$, where $v=v(x)$ is a. Learn what a homogeneous differential equation is and how to solve it using the substitution method. See the definition, steps and solved examples.
In this section we will extend the ideas behind solving 2nd order, linear, homogeneous differential equations to higher. Using y = vx and dy dx = v + x dv dx we can solve the differential equation. A differential equation of the form dy/dx = f (x, y)/ g (x, y) is called homogeneous differential equation if f (x, y) and g(x, y) are homogeneous. See the definition, steps and solved examples. The general form of a homogeneous differential equation is f(x, y).dy + g(x, y).dx = 0. Learn what a homogeneous differential equation is and how to solve it using the substitution method. Let us learn more about the homogeneous differential. An example will show how it is all done: A homogeneous differential equation can often be solved by making the substitution $v(x)=\dfrac{y}{x}$, where $v=v(x)$ is a.
College Park Tutors Blog Differential Equations Solving a second
Using y = vx and dy dx = v + x dv dx we can solve the differential equation. A homogeneous differential equation can often be solved by making the substitution $v(x)=\dfrac{y}{x}$, where $v=v(x)$ is a. In this section we will extend the ideas behind solving 2nd order, linear, homogeneous differential equations to higher. The general form of a homogeneous.
Ex 9.5, 17 Which is a homogeneous differential equation
In this section we will extend the ideas behind solving 2nd order, linear, homogeneous differential equations to higher. The general form of a homogeneous differential equation is f(x, y).dy + g(x, y).dx = 0. A homogeneous differential equation can often be solved by making the substitution $v(x)=\dfrac{y}{x}$, where $v=v(x)$ is a. A differential equation of the form dy/dx = f.
MODULE 04 Equations of Order One Homogeneous Differential Equations
Using y = vx and dy dx = v + x dv dx we can solve the differential equation. Let us learn more about the homogeneous differential. An example will show how it is all done: See the definition, steps and solved examples. In this section we will extend the ideas behind solving 2nd order, linear, homogeneous differential equations to.
⏩SOLVEDSolving a Homogeneous Differential Equation In Exercises
An example will show how it is all done: Learn what a homogeneous differential equation is and how to solve it using the substitution method. In this section we will extend the ideas behind solving 2nd order, linear, homogeneous differential equations to higher. Using y = vx and dy dx = v + x dv dx we can solve the.
College Park Tutors Blog Differential Equations Solving a second
Learn what a homogeneous differential equation is and how to solve it using the substitution method. The general form of a homogeneous differential equation is f(x, y).dy + g(x, y).dx = 0. A homogeneous differential equation can often be solved by making the substitution $v(x)=\dfrac{y}{x}$, where $v=v(x)$ is a. See the definition, steps and solved examples. An example will show.
Homogeneous Differential Equations HandWritten Notes in JPG Format
The general form of a homogeneous differential equation is f(x, y).dy + g(x, y).dx = 0. Let us learn more about the homogeneous differential. Using y = vx and dy dx = v + x dv dx we can solve the differential equation. A homogeneous differential equation can often be solved by making the substitution $v(x)=\dfrac{y}{x}$, where $v=v(x)$ is a..
College Park Tutors Blog Differential Equations Solving a second
In this section we will extend the ideas behind solving 2nd order, linear, homogeneous differential equations to higher. Learn what a homogeneous differential equation is and how to solve it using the substitution method. See the definition, steps and solved examples. Using y = vx and dy dx = v + x dv dx we can solve the differential equation..
College Park Tutors Blog Differential Equations Solving a second
Using y = vx and dy dx = v + x dv dx we can solve the differential equation. See the definition, steps and solved examples. The general form of a homogeneous differential equation is f(x, y).dy + g(x, y).dx = 0. In this section we will extend the ideas behind solving 2nd order, linear, homogeneous differential equations to higher..
Homogeneous Differential Equation2 PDF Waves Applied And
An example will show how it is all done: A differential equation of the form dy/dx = f (x, y)/ g (x, y) is called homogeneous differential equation if f (x, y) and g(x, y) are homogeneous. Let us learn more about the homogeneous differential. A homogeneous differential equation can often be solved by making the substitution $v(x)=\dfrac{y}{x}$, where $v=v(x)$.
Solving Homogeneous Differential Equations Maths Science
A differential equation of the form dy/dx = f (x, y)/ g (x, y) is called homogeneous differential equation if f (x, y) and g(x, y) are homogeneous. The general form of a homogeneous differential equation is f(x, y).dy + g(x, y).dx = 0. An example will show how it is all done: A homogeneous differential equation can often be.
See The Definition, Steps And Solved Examples.
A differential equation of the form dy/dx = f (x, y)/ g (x, y) is called homogeneous differential equation if f (x, y) and g(x, y) are homogeneous. The general form of a homogeneous differential equation is f(x, y).dy + g(x, y).dx = 0. An example will show how it is all done: Using y = vx and dy dx = v + x dv dx we can solve the differential equation.
A Homogeneous Differential Equation Can Often Be Solved By Making The Substitution $V(X)=\Dfrac{Y}{X}$, Where $V=V(X)$ Is A.
Learn what a homogeneous differential equation is and how to solve it using the substitution method. Let us learn more about the homogeneous differential. In this section we will extend the ideas behind solving 2nd order, linear, homogeneous differential equations to higher.