Second Order Homogeneous Linear Differential Equation - Se equations is achieved in stages. Y'' + p(x)y' + q(x)y = f (x) y ′ ′ + p (x) y ′ + q (x) y. A linear homogeneous second order ode with constant coefficients is an ordinary differential equation in the form: The first stage is to find what is cal. We call the function \(f\). There are two types of second order linear differential equations: A second order differential equation is said to be linear if it can be written as \[\label{eq:5.1.1} y''+p(x)y'+q(x)y=f(x).
Se equations is achieved in stages. There are two types of second order linear differential equations: A linear homogeneous second order ode with constant coefficients is an ordinary differential equation in the form: The first stage is to find what is cal. A second order differential equation is said to be linear if it can be written as \[\label{eq:5.1.1} y''+p(x)y'+q(x)y=f(x). Y'' + p(x)y' + q(x)y = f (x) y ′ ′ + p (x) y ′ + q (x) y. We call the function \(f\).
A second order differential equation is said to be linear if it can be written as \[\label{eq:5.1.1} y''+p(x)y'+q(x)y=f(x). Y'' + p(x)y' + q(x)y = f (x) y ′ ′ + p (x) y ′ + q (x) y. The first stage is to find what is cal. We call the function \(f\). There are two types of second order linear differential equations: Se equations is achieved in stages. A linear homogeneous second order ode with constant coefficients is an ordinary differential equation in the form:
SOLUTION Second order homogeneous linear differential equation Studypool
There are two types of second order linear differential equations: The first stage is to find what is cal. A linear homogeneous second order ode with constant coefficients is an ordinary differential equation in the form: We call the function \(f\). Y'' + p(x)y' + q(x)y = f (x) y ′ ′ + p (x) y ′ + q (x).
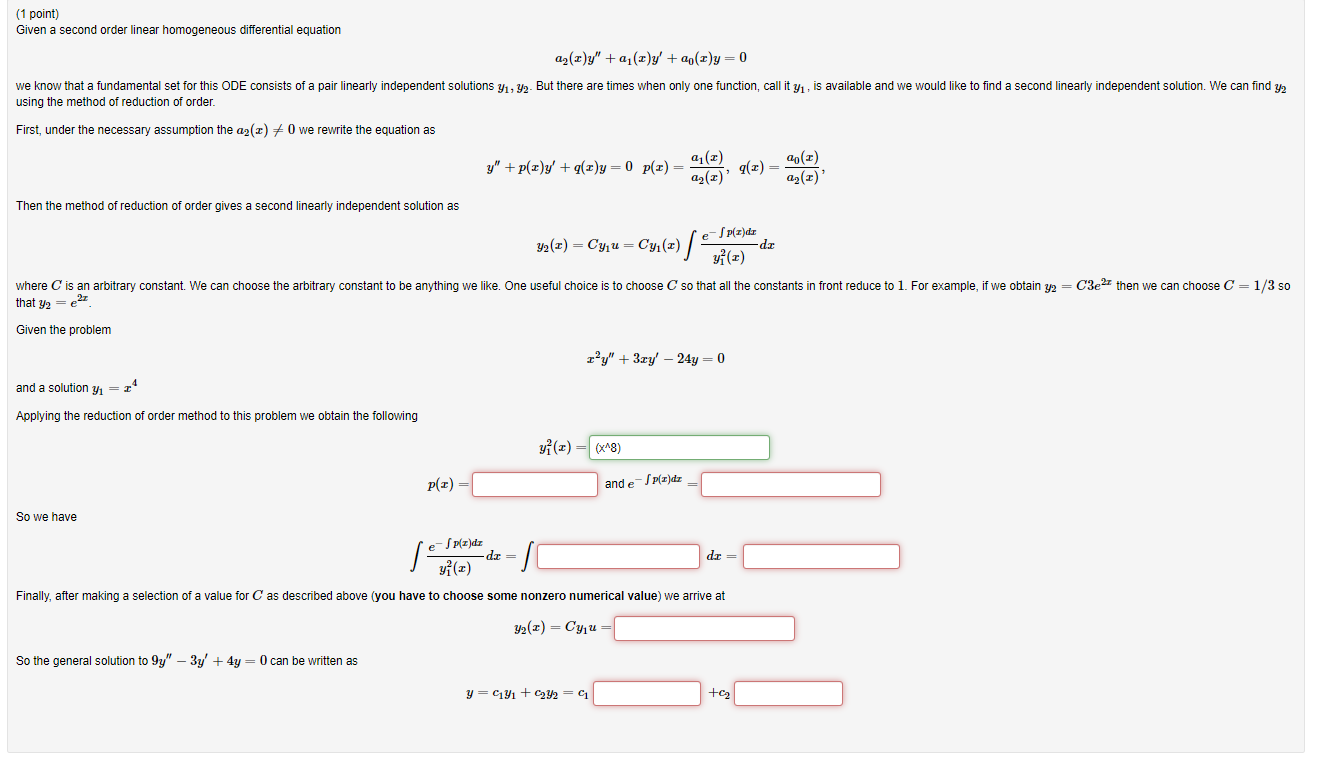
Solved Given a second order linear homogeneous differential
The first stage is to find what is cal. Se equations is achieved in stages. A linear homogeneous second order ode with constant coefficients is an ordinary differential equation in the form: Y'' + p(x)y' + q(x)y = f (x) y ′ ′ + p (x) y ′ + q (x) y. We call the function \(f\).
Solved 1 point) Given a second order linear homogeneous
The first stage is to find what is cal. There are two types of second order linear differential equations: Se equations is achieved in stages. Y'' + p(x)y' + q(x)y = f (x) y ′ ′ + p (x) y ′ + q (x) y. A linear homogeneous second order ode with constant coefficients is an ordinary differential equation in.
Solved Given a second order linear homogeneous differential
The first stage is to find what is cal. A linear homogeneous second order ode with constant coefficients is an ordinary differential equation in the form: A second order differential equation is said to be linear if it can be written as \[\label{eq:5.1.1} y''+p(x)y'+q(x)y=f(x). We call the function \(f\). There are two types of second order linear differential equations:
Solved Other questions 1. Given a homogeneous linear second
A second order differential equation is said to be linear if it can be written as \[\label{eq:5.1.1} y''+p(x)y'+q(x)y=f(x). Y'' + p(x)y' + q(x)y = f (x) y ′ ′ + p (x) y ′ + q (x) y. We call the function \(f\). Se equations is achieved in stages. The first stage is to find what is cal.
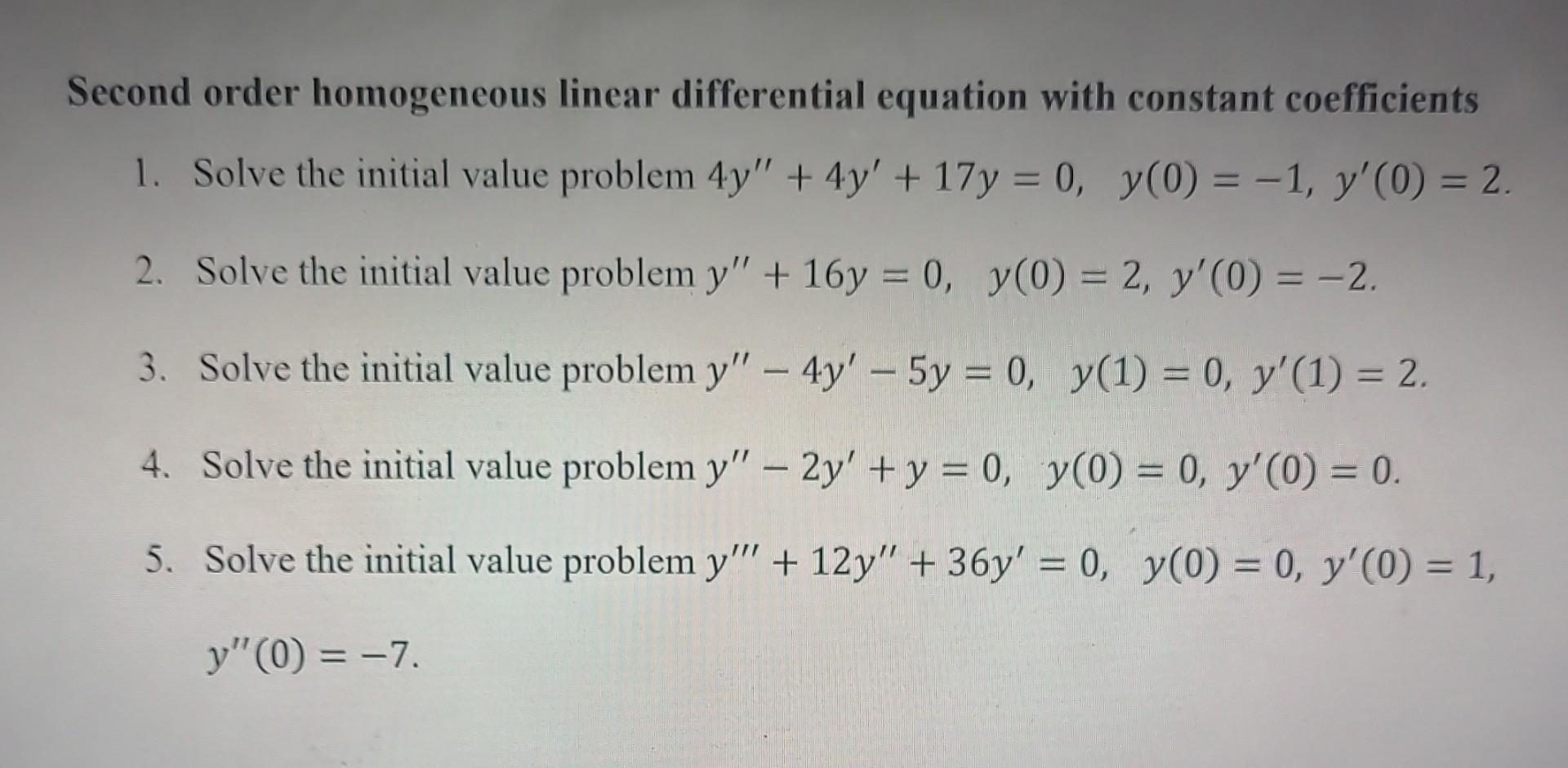
Solved Second order homogeneous linear differential equation
There are two types of second order linear differential equations: A linear homogeneous second order ode with constant coefficients is an ordinary differential equation in the form: Y'' + p(x)y' + q(x)y = f (x) y ′ ′ + p (x) y ′ + q (x) y. The first stage is to find what is cal. A second order differential.
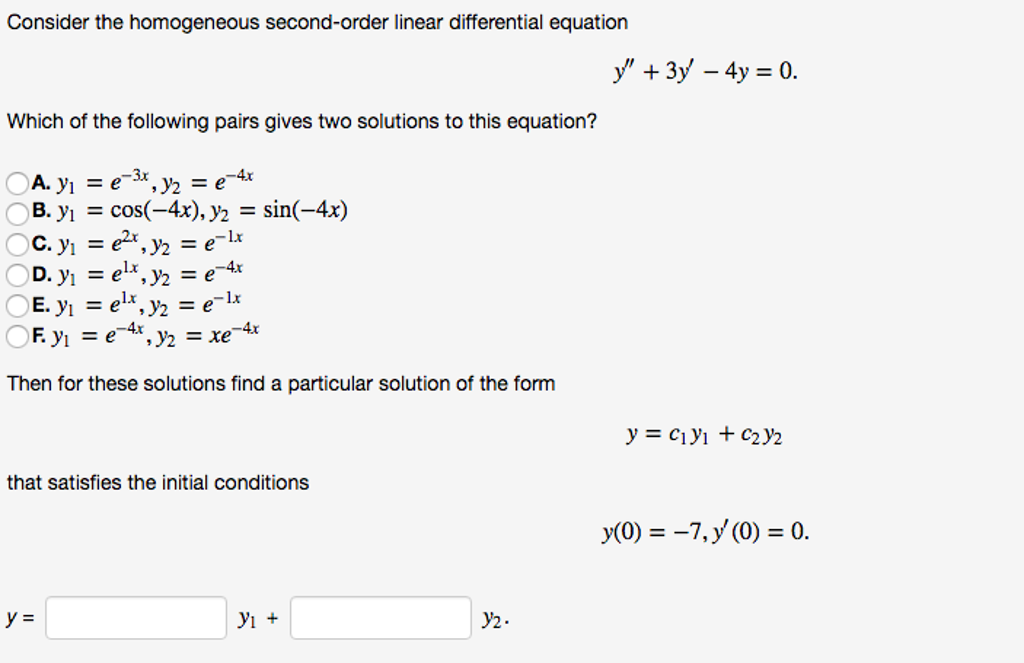
Solved Consider the homogeneous secondorder linear
We call the function \(f\). Y'' + p(x)y' + q(x)y = f (x) y ′ ′ + p (x) y ′ + q (x) y. The first stage is to find what is cal. Se equations is achieved in stages. There are two types of second order linear differential equations:
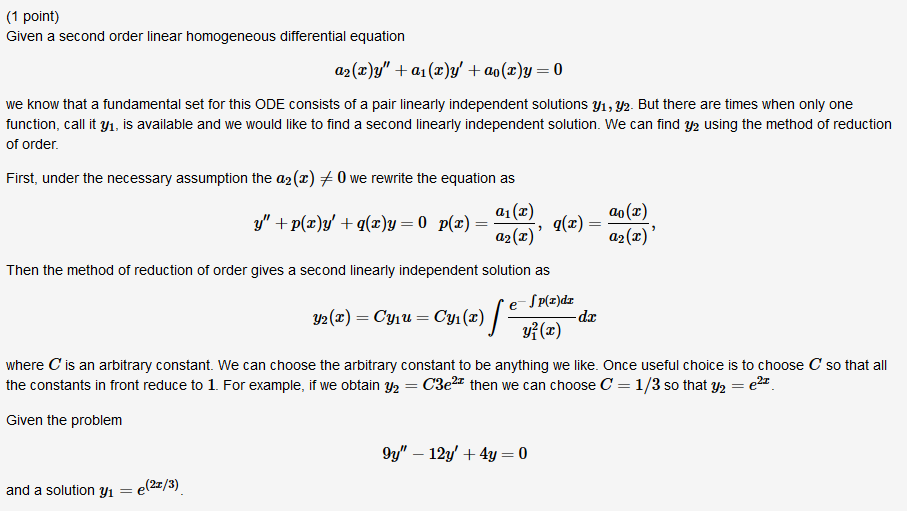
Solved Given a second order linear homogeneous differential
The first stage is to find what is cal. A linear homogeneous second order ode with constant coefficients is an ordinary differential equation in the form: Se equations is achieved in stages. We call the function \(f\). Y'' + p(x)y' + q(x)y = f (x) y ′ ′ + p (x) y ′ + q (x) y.
Solved 2. Consider the following second order linear
Se equations is achieved in stages. A second order differential equation is said to be linear if it can be written as \[\label{eq:5.1.1} y''+p(x)y'+q(x)y=f(x). Y'' + p(x)y' + q(x)y = f (x) y ′ ′ + p (x) y ′ + q (x) y. We call the function \(f\). A linear homogeneous second order ode with constant coefficients is an.
Solved Given a second order linear homogeneous differential
The first stage is to find what is cal. Y'' + p(x)y' + q(x)y = f (x) y ′ ′ + p (x) y ′ + q (x) y. We call the function \(f\). A second order differential equation is said to be linear if it can be written as \[\label{eq:5.1.1} y''+p(x)y'+q(x)y=f(x). A linear homogeneous second order ode with constant.
There Are Two Types Of Second Order Linear Differential Equations:
A second order differential equation is said to be linear if it can be written as \[\label{eq:5.1.1} y''+p(x)y'+q(x)y=f(x). The first stage is to find what is cal. We call the function \(f\). A linear homogeneous second order ode with constant coefficients is an ordinary differential equation in the form:
Se Equations Is Achieved In Stages.
Y'' + p(x)y' + q(x)y = f (x) y ′ ′ + p (x) y ′ + q (x) y.