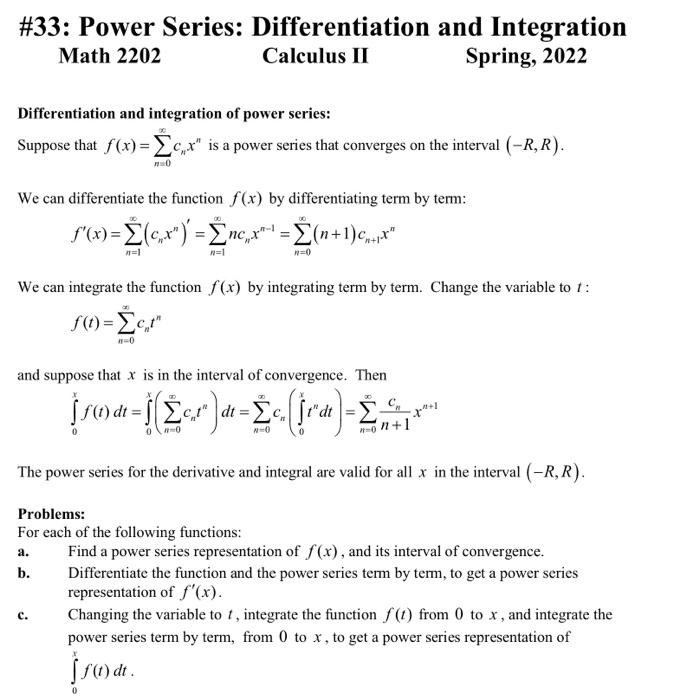
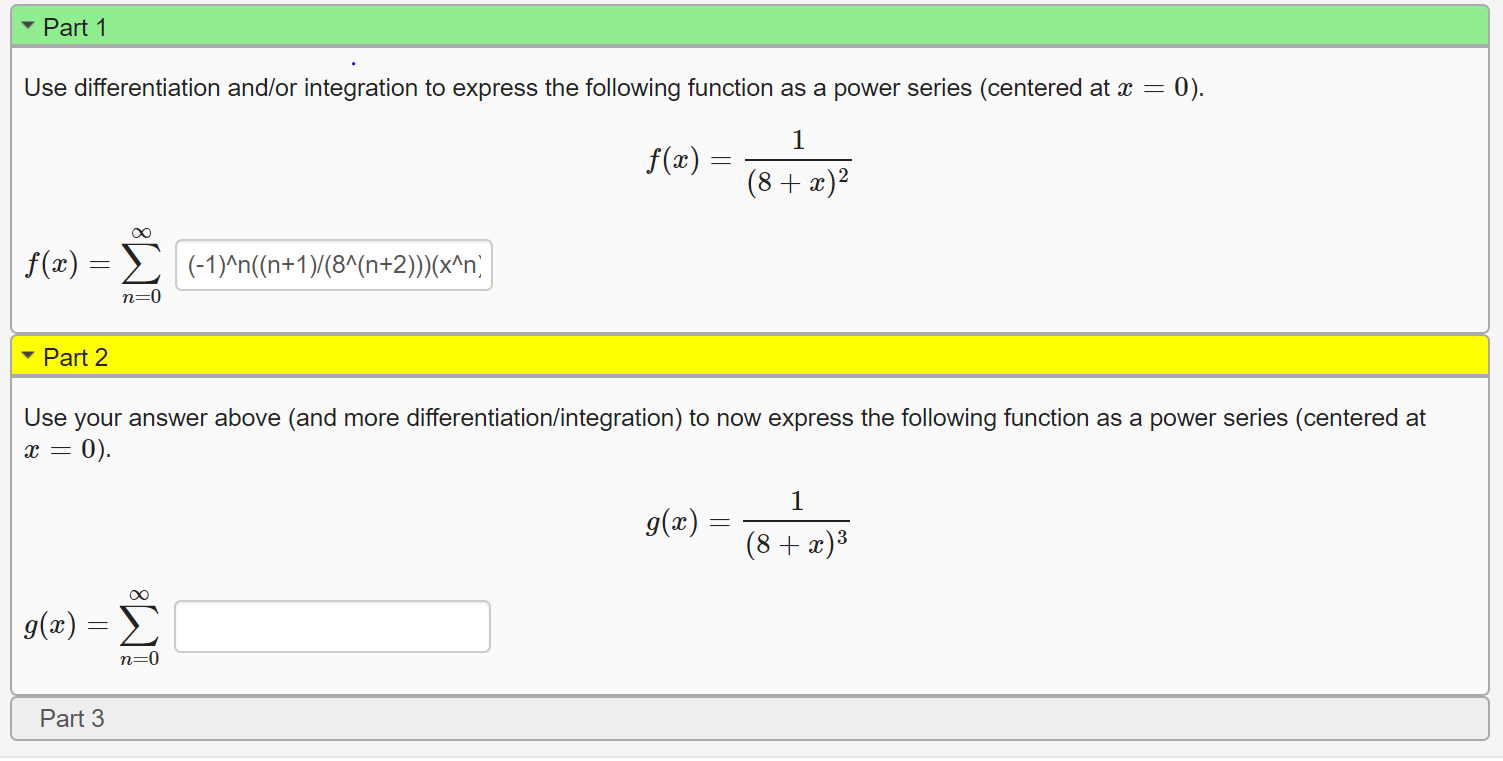
Power Series Differentiation And Integration - Let's do calculus on this function. The derivative of the power series exists and is. To use the geometric series formula, the function must be able to be put into a specific form, which is often impossible. We show how to do this in the next two examples. When a power series converges, it defines a function. Power series diff and integ geometric series radius of convergence variations on the geometric series (i) closed forms for many.
Power series diff and integ geometric series radius of convergence variations on the geometric series (i) closed forms for many. Let's do calculus on this function. We show how to do this in the next two examples. To use the geometric series formula, the function must be able to be put into a specific form, which is often impossible. The derivative of the power series exists and is. When a power series converges, it defines a function.
When a power series converges, it defines a function. The derivative of the power series exists and is. To use the geometric series formula, the function must be able to be put into a specific form, which is often impossible. Let's do calculus on this function. Power series diff and integ geometric series radius of convergence variations on the geometric series (i) closed forms for many. We show how to do this in the next two examples.
Power series differentiation to find the function's representation
The derivative of the power series exists and is. We show how to do this in the next two examples. Power series diff and integ geometric series radius of convergence variations on the geometric series (i) closed forms for many. Let's do calculus on this function. To use the geometric series formula, the function must be able to be put.
Solved 33 Power Series Differentiation and Integration
We show how to do this in the next two examples. To use the geometric series formula, the function must be able to be put into a specific form, which is often impossible. When a power series converges, it defines a function. The derivative of the power series exists and is. Power series diff and integ geometric series radius of.
13.Differentiation and Integration of Power Series.pdf Power Series
When a power series converges, it defines a function. To use the geometric series formula, the function must be able to be put into a specific form, which is often impossible. Let's do calculus on this function. Power series diff and integ geometric series radius of convergence variations on the geometric series (i) closed forms for many. The derivative of.
Differentiation and Integration of Power Series DocsLib
Power series diff and integ geometric series radius of convergence variations on the geometric series (i) closed forms for many. We show how to do this in the next two examples. When a power series converges, it defines a function. The derivative of the power series exists and is. Let's do calculus on this function.
Solved Part 1 Use differentiation and/or integration to
To use the geometric series formula, the function must be able to be put into a specific form, which is often impossible. Let's do calculus on this function. The derivative of the power series exists and is. We show how to do this in the next two examples. Power series diff and integ geometric series radius of convergence variations on.
Power series differentiation to find the function's representation
The derivative of the power series exists and is. To use the geometric series formula, the function must be able to be put into a specific form, which is often impossible. Power series diff and integ geometric series radius of convergence variations on the geometric series (i) closed forms for many. Let's do calculus on this function. When a power.
Power Series PDF
Let's do calculus on this function. When a power series converges, it defines a function. To use the geometric series formula, the function must be able to be put into a specific form, which is often impossible. We show how to do this in the next two examples. Power series diff and integ geometric series radius of convergence variations on.
Differentiation and Integration Mean Green Math
When a power series converges, it defines a function. To use the geometric series formula, the function must be able to be put into a specific form, which is often impossible. We show how to do this in the next two examples. Power series diff and integ geometric series radius of convergence variations on the geometric series (i) closed forms.
Power Series PDF
When a power series converges, it defines a function. Power series diff and integ geometric series radius of convergence variations on the geometric series (i) closed forms for many. Let's do calculus on this function. To use the geometric series formula, the function must be able to be put into a specific form, which is often impossible. We show how.
Solved Part 1 Use differentiation and/or integration to
We show how to do this in the next two examples. When a power series converges, it defines a function. To use the geometric series formula, the function must be able to be put into a specific form, which is often impossible. Power series diff and integ geometric series radius of convergence variations on the geometric series (i) closed forms.
The Derivative Of The Power Series Exists And Is.
When a power series converges, it defines a function. Power series diff and integ geometric series radius of convergence variations on the geometric series (i) closed forms for many. We show how to do this in the next two examples. Let's do calculus on this function.