Parabolic Differential Equation - (6.1) ut = ∆u + f is a prototypical example of a parabolic pde. If b2 4ac = 0, then the pde is parabolic (heat). Tumor growth models describe the diffusion of cancer cells through the tissue. This pde has to be. If b2 4ac > 0, then the pde is hyperbolic (wave).
If b2 4ac = 0, then the pde is parabolic (heat). This pde has to be. Tumor growth models describe the diffusion of cancer cells through the tissue. (6.1) ut = ∆u + f is a prototypical example of a parabolic pde. If b2 4ac > 0, then the pde is hyperbolic (wave).
If b2 4ac = 0, then the pde is parabolic (heat). Tumor growth models describe the diffusion of cancer cells through the tissue. If b2 4ac > 0, then the pde is hyperbolic (wave). This pde has to be. (6.1) ut = ∆u + f is a prototypical example of a parabolic pde.
SOLUTION Chapter 7 parabolic differential equations Studypool
If b2 4ac > 0, then the pde is hyperbolic (wave). Tumor growth models describe the diffusion of cancer cells through the tissue. (6.1) ut = ∆u + f is a prototypical example of a parabolic pde. This pde has to be. If b2 4ac = 0, then the pde is parabolic (heat).
(PDF) Structure of a Parabolic Partial Differential Equation on Graphs
Tumor growth models describe the diffusion of cancer cells through the tissue. If b2 4ac > 0, then the pde is hyperbolic (wave). (6.1) ut = ∆u + f is a prototypical example of a parabolic pde. This pde has to be. If b2 4ac = 0, then the pde is parabolic (heat).
PPT Parabolic Partial Differential Equations PowerPoint Presentation
(6.1) ut = ∆u + f is a prototypical example of a parabolic pde. If b2 4ac = 0, then the pde is parabolic (heat). If b2 4ac > 0, then the pde is hyperbolic (wave). Tumor growth models describe the diffusion of cancer cells through the tissue. This pde has to be.
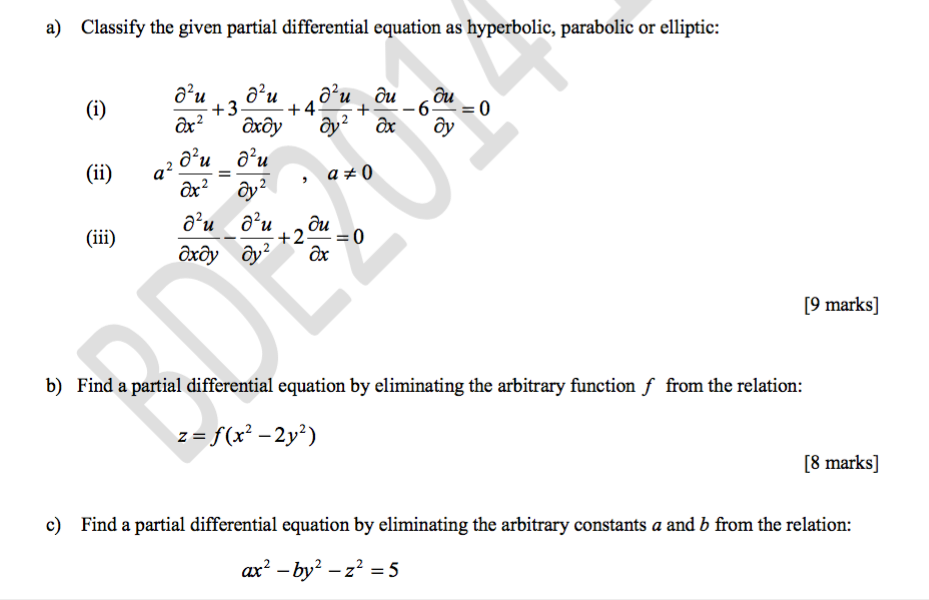
Solved a) Classify the given partial differential equation
If b2 4ac = 0, then the pde is parabolic (heat). (6.1) ut = ∆u + f is a prototypical example of a parabolic pde. If b2 4ac > 0, then the pde is hyperbolic (wave). Tumor growth models describe the diffusion of cancer cells through the tissue. This pde has to be.
Localized orthogonal for a multiscale parabolic
(6.1) ut = ∆u + f is a prototypical example of a parabolic pde. If b2 4ac = 0, then the pde is parabolic (heat). If b2 4ac > 0, then the pde is hyperbolic (wave). Tumor growth models describe the diffusion of cancer cells through the tissue. This pde has to be.
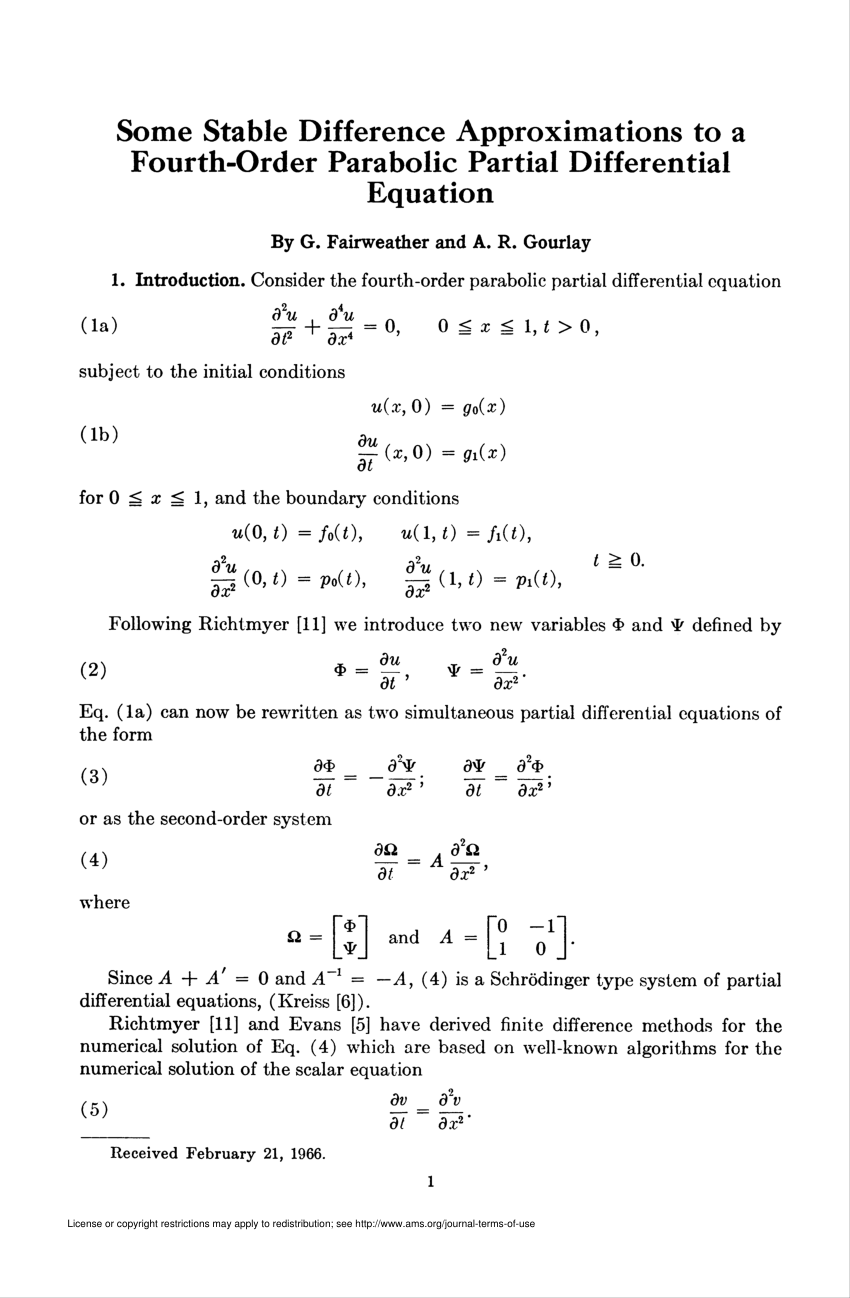
(PDF) Some Stable Difference Approximations to a FourthOrder Parabolic
If b2 4ac = 0, then the pde is parabolic (heat). If b2 4ac > 0, then the pde is hyperbolic (wave). (6.1) ut = ∆u + f is a prototypical example of a parabolic pde. Tumor growth models describe the diffusion of cancer cells through the tissue. This pde has to be.
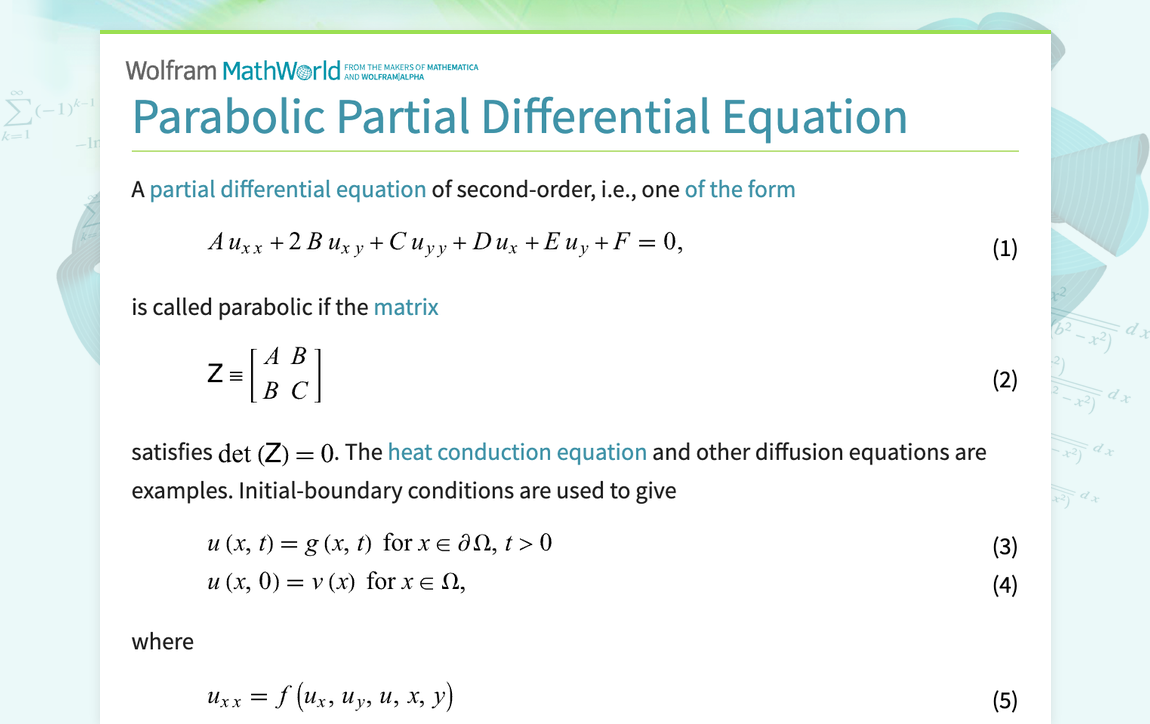
Parabolic Partial Differential Equation from Wolfram MathWorld
Tumor growth models describe the diffusion of cancer cells through the tissue. If b2 4ac > 0, then the pde is hyperbolic (wave). This pde has to be. (6.1) ut = ∆u + f is a prototypical example of a parabolic pde. If b2 4ac = 0, then the pde is parabolic (heat).
(PDF) Normal Forms For Parabolic Partial Differential Equations
This pde has to be. If b2 4ac > 0, then the pde is hyperbolic (wave). (6.1) ut = ∆u + f is a prototypical example of a parabolic pde. Tumor growth models describe the diffusion of cancer cells through the tissue. If b2 4ac = 0, then the pde is parabolic (heat).
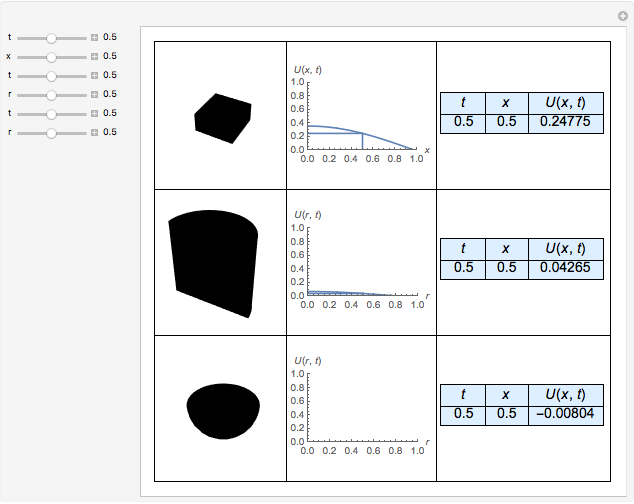
A Parabolic Partial Differential Equation in Three Different Geometries
If b2 4ac = 0, then the pde is parabolic (heat). This pde has to be. (6.1) ut = ∆u + f is a prototypical example of a parabolic pde. Tumor growth models describe the diffusion of cancer cells through the tissue. If b2 4ac > 0, then the pde is hyperbolic (wave).
(PDF) Parameteruniformly convergent numerical scheme for singularly
Tumor growth models describe the diffusion of cancer cells through the tissue. This pde has to be. (6.1) ut = ∆u + f is a prototypical example of a parabolic pde. If b2 4ac > 0, then the pde is hyperbolic (wave). If b2 4ac = 0, then the pde is parabolic (heat).
(6.1) Ut = ∆U + F Is A Prototypical Example Of A Parabolic Pde.
If b2 4ac = 0, then the pde is parabolic (heat). Tumor growth models describe the diffusion of cancer cells through the tissue. If b2 4ac > 0, then the pde is hyperbolic (wave). This pde has to be.