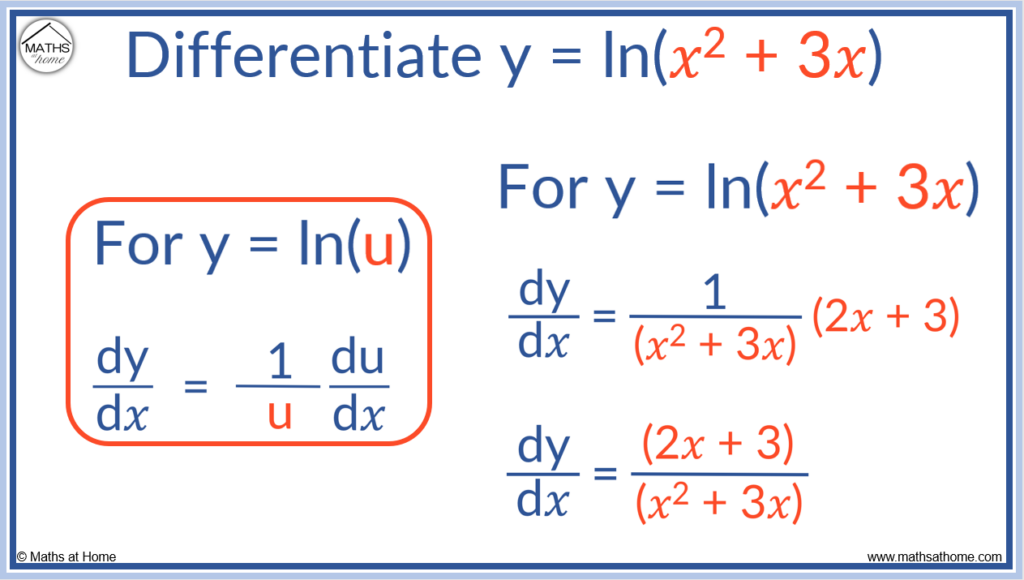
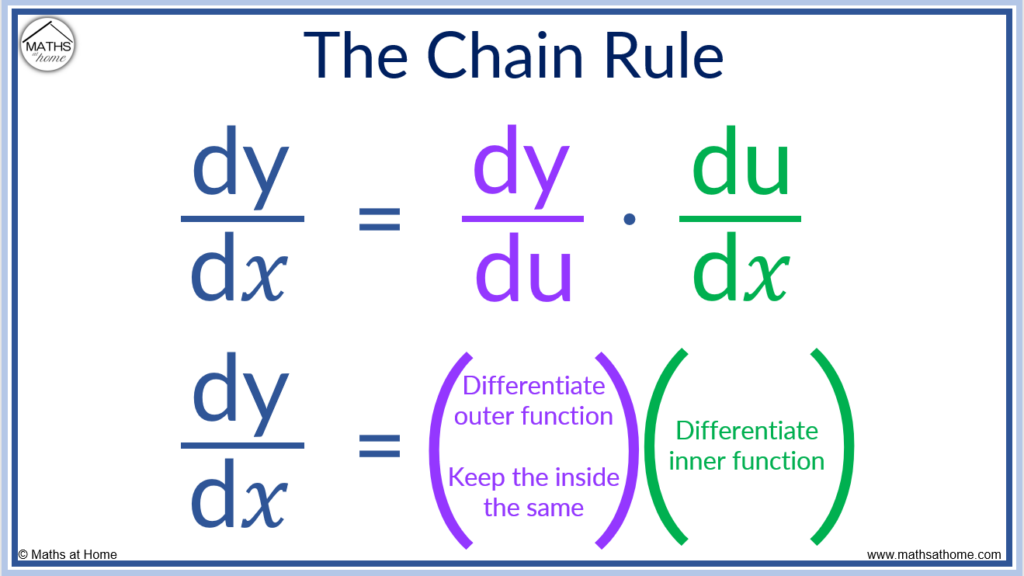
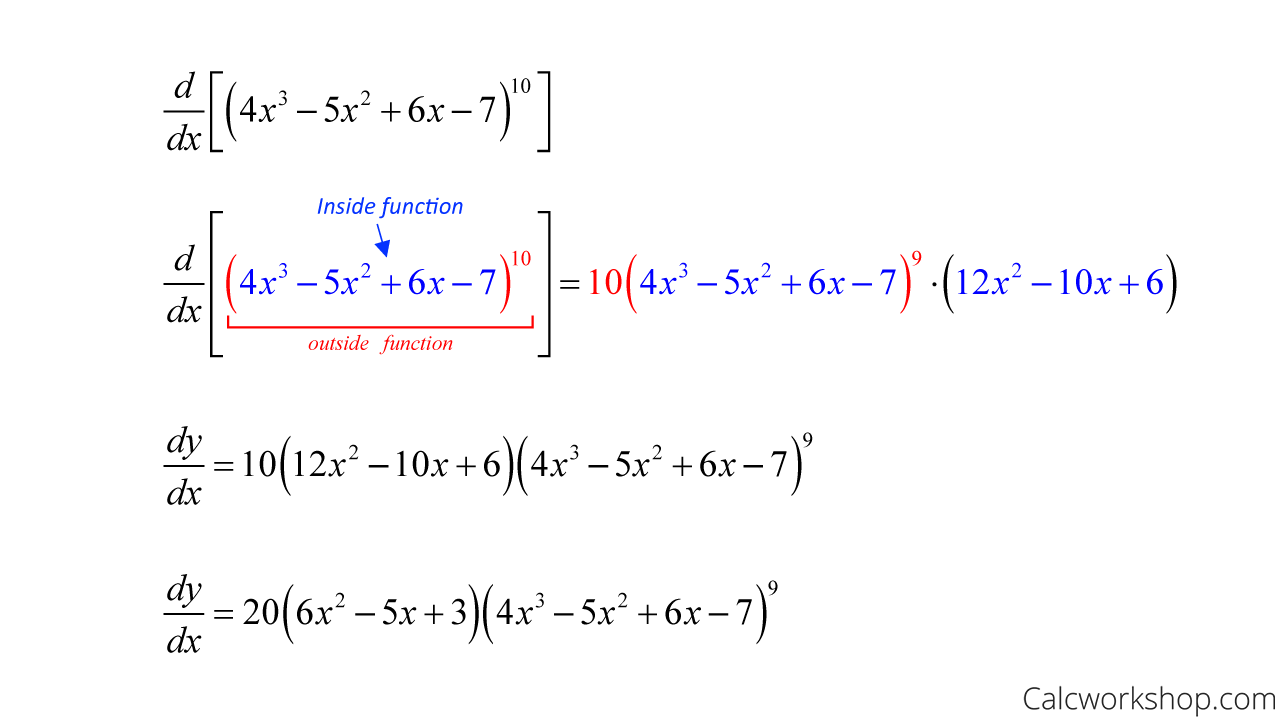Matrix Differentiation Chain Rule - The matrices df(y) 2 m(n;p) and dr(x) 2m(p;m) combine to the matrix product dfdrat a point. Use the chain rule to find relations between different partial derivatives of a function. The matrices df(y) 2 m(n;p) and dr(x) 2m(p;m) combine to the matrix product dfdrat a point. The purpose of this document is to help you learn to take derivatives of vectors, matrices, and. Rk × k → rn × n as a(b) = c ′ bc. My problem is computing $\frac{\partial h}{\partial w_1}$. Denote also g(a) = [gij(a)], a = [aij], c = [cij].
The matrices df(y) 2 m(n;p) and dr(x) 2m(p;m) combine to the matrix product dfdrat a point. My problem is computing $\frac{\partial h}{\partial w_1}$. Denote also g(a) = [gij(a)], a = [aij], c = [cij]. The purpose of this document is to help you learn to take derivatives of vectors, matrices, and. Use the chain rule to find relations between different partial derivatives of a function. The matrices df(y) 2 m(n;p) and dr(x) 2m(p;m) combine to the matrix product dfdrat a point. Rk × k → rn × n as a(b) = c ′ bc.
Use the chain rule to find relations between different partial derivatives of a function. Rk × k → rn × n as a(b) = c ′ bc. The purpose of this document is to help you learn to take derivatives of vectors, matrices, and. My problem is computing $\frac{\partial h}{\partial w_1}$. The matrices df(y) 2 m(n;p) and dr(x) 2m(p;m) combine to the matrix product dfdrat a point. The matrices df(y) 2 m(n;p) and dr(x) 2m(p;m) combine to the matrix product dfdrat a point. Denote also g(a) = [gij(a)], a = [aij], c = [cij].
calculus Automatic Differentiation Chain Rule Question
The matrices df(y) 2 m(n;p) and dr(x) 2m(p;m) combine to the matrix product dfdrat a point. Use the chain rule to find relations between different partial derivatives of a function. My problem is computing $\frac{\partial h}{\partial w_1}$. The purpose of this document is to help you learn to take derivatives of vectors, matrices, and. Denote also g(a) = [gij(a)], a.
Chain Rule Differentiation Benytr
The matrices df(y) 2 m(n;p) and dr(x) 2m(p;m) combine to the matrix product dfdrat a point. My problem is computing $\frac{\partial h}{\partial w_1}$. The matrices df(y) 2 m(n;p) and dr(x) 2m(p;m) combine to the matrix product dfdrat a point. Rk × k → rn × n as a(b) = c ′ bc. The purpose of this document is to help.
14.5 The Chain Rule PDF Derivative Function (Mathematics)
The purpose of this document is to help you learn to take derivatives of vectors, matrices, and. Denote also g(a) = [gij(a)], a = [aij], c = [cij]. Rk × k → rn × n as a(b) = c ′ bc. My problem is computing $\frac{\partial h}{\partial w_1}$. The matrices df(y) 2 m(n;p) and dr(x) 2m(p;m) combine to the matrix.
The Chain Rule Made Easy Examples and Solutions
The matrices df(y) 2 m(n;p) and dr(x) 2m(p;m) combine to the matrix product dfdrat a point. The matrices df(y) 2 m(n;p) and dr(x) 2m(p;m) combine to the matrix product dfdrat a point. Rk × k → rn × n as a(b) = c ′ bc. My problem is computing $\frac{\partial h}{\partial w_1}$. Use the chain rule to find relations between.
The Chain Rule Made Easy Examples and Solutions
Denote also g(a) = [gij(a)], a = [aij], c = [cij]. Rk × k → rn × n as a(b) = c ′ bc. Use the chain rule to find relations between different partial derivatives of a function. The matrices df(y) 2 m(n;p) and dr(x) 2m(p;m) combine to the matrix product dfdrat a point. My problem is computing $\frac{\partial h}{\partial.
The Chain Rule Made Easy Examples and Solutions
My problem is computing $\frac{\partial h}{\partial w_1}$. Rk × k → rn × n as a(b) = c ′ bc. Denote also g(a) = [gij(a)], a = [aij], c = [cij]. The matrices df(y) 2 m(n;p) and dr(x) 2m(p;m) combine to the matrix product dfdrat a point. The purpose of this document is to help you learn to take derivatives.
Formula of Differentiation by chain rule With solved example
The matrices df(y) 2 m(n;p) and dr(x) 2m(p;m) combine to the matrix product dfdrat a point. My problem is computing $\frac{\partial h}{\partial w_1}$. The purpose of this document is to help you learn to take derivatives of vectors, matrices, and. Use the chain rule to find relations between different partial derivatives of a function. The matrices df(y) 2 m(n;p) and.
Chain Rule Applications of Chain Rule PDF Subtraction Syntax (Logic)
Denote also g(a) = [gij(a)], a = [aij], c = [cij]. My problem is computing $\frac{\partial h}{\partial w_1}$. Use the chain rule to find relations between different partial derivatives of a function. The purpose of this document is to help you learn to take derivatives of vectors, matrices, and. The matrices df(y) 2 m(n;p) and dr(x) 2m(p;m) combine to the.
M53 Lec2.2 The Chain Rule and Differentiability PDF
Rk × k → rn × n as a(b) = c ′ bc. Denote also g(a) = [gij(a)], a = [aij], c = [cij]. The purpose of this document is to help you learn to take derivatives of vectors, matrices, and. The matrices df(y) 2 m(n;p) and dr(x) 2m(p;m) combine to the matrix product dfdrat a point. Use the chain.
Lecture 2 Continue Intro Diff and Chain Rule PDF
The matrices df(y) 2 m(n;p) and dr(x) 2m(p;m) combine to the matrix product dfdrat a point. My problem is computing $\frac{\partial h}{\partial w_1}$. Denote also g(a) = [gij(a)], a = [aij], c = [cij]. Use the chain rule to find relations between different partial derivatives of a function. The purpose of this document is to help you learn to take.
Rk × K → Rn × N As A(B) = C ′ Bc.
Use the chain rule to find relations between different partial derivatives of a function. The matrices df(y) 2 m(n;p) and dr(x) 2m(p;m) combine to the matrix product dfdrat a point. Denote also g(a) = [gij(a)], a = [aij], c = [cij]. My problem is computing $\frac{\partial h}{\partial w_1}$.
The Purpose Of This Document Is To Help You Learn To Take Derivatives Of Vectors, Matrices, And.
The matrices df(y) 2 m(n;p) and dr(x) 2m(p;m) combine to the matrix product dfdrat a point.