Let F Be A Twice Differentiable Function - The correct answer is f(x)+∫0x (x−t)f'(t)dt=e2x+e−2xcos2x+2ax differentiating. Let f be a function that is twice differentiable for all real numbers. The table above gives values. Let g(x) = log(f(x)), where f(x) is twice differentiable function on (0, ∞) such that. Let $f$ be twice differentiable function on $(0,1)$. Let f be a function twice differentiable and with derivatives continuous on an interval $[a,b]$.
Let f be a function twice differentiable and with derivatives continuous on an interval $[a,b]$. Let $f$ be twice differentiable function on $(0,1)$. The table above gives values. The correct answer is f(x)+∫0x (x−t)f'(t)dt=e2x+e−2xcos2x+2ax differentiating. Let g(x) = log(f(x)), where f(x) is twice differentiable function on (0, ∞) such that. Let f be a function that is twice differentiable for all real numbers.
Let f be a function twice differentiable and with derivatives continuous on an interval $[a,b]$. Let g(x) = log(f(x)), where f(x) is twice differentiable function on (0, ∞) such that. Let $f$ be twice differentiable function on $(0,1)$. The table above gives values. The correct answer is f(x)+∫0x (x−t)f'(t)dt=e2x+e−2xcos2x+2ax differentiating. Let f be a function that is twice differentiable for all real numbers.
[Solved] Let f be a twicedifferentiable function such that f '(1)= 0
The table above gives values. Let g(x) = log(f(x)), where f(x) is twice differentiable function on (0, ∞) such that. Let $f$ be twice differentiable function on $(0,1)$. Let f be a function twice differentiable and with derivatives continuous on an interval $[a,b]$. Let f be a function that is twice differentiable for all real numbers.
Solved Let f be a twicedifferentiable function such that
Let g(x) = log(f(x)), where f(x) is twice differentiable function on (0, ∞) such that. The table above gives values. Let f be a function that is twice differentiable for all real numbers. Let $f$ be twice differentiable function on $(0,1)$. Let f be a function twice differentiable and with derivatives continuous on an interval $[a,b]$.
Solved Let f be a twicedifferentiable function. Values of
The table above gives values. Let g(x) = log(f(x)), where f(x) is twice differentiable function on (0, ∞) such that. Let f be a function that is twice differentiable for all real numbers. Let f be a function twice differentiable and with derivatives continuous on an interval $[a,b]$. The correct answer is f(x)+∫0x (x−t)f'(t)dt=e2x+e−2xcos2x+2ax differentiating.
Solved Let f be a twicedifferentiable function defined by
The table above gives values. Let g(x) = log(f(x)), where f(x) is twice differentiable function on (0, ∞) such that. Let f be a function twice differentiable and with derivatives continuous on an interval $[a,b]$. Let $f$ be twice differentiable function on $(0,1)$. Let f be a function that is twice differentiable for all real numbers.
SOLVEDLet f be a twice differentiable function such that f^''(x)=f(x
Let $f$ be twice differentiable function on $(0,1)$. Let f be a function that is twice differentiable for all real numbers. The correct answer is f(x)+∫0x (x−t)f'(t)dt=e2x+e−2xcos2x+2ax differentiating. Let g(x) = log(f(x)), where f(x) is twice differentiable function on (0, ∞) such that. The table above gives values.
Solved Let y = f (x) be a twicedifferentiable function such
Let g(x) = log(f(x)), where f(x) is twice differentiable function on (0, ∞) such that. The correct answer is f(x)+∫0x (x−t)f'(t)dt=e2x+e−2xcos2x+2ax differentiating. Let f be a function that is twice differentiable for all real numbers. Let f be a function twice differentiable and with derivatives continuous on an interval $[a,b]$. Let $f$ be twice differentiable function on $(0,1)$.
Solved Let y=f(x) be a twicedifferentiable function such
Let f be a function that is twice differentiable for all real numbers. Let f be a function twice differentiable and with derivatives continuous on an interval $[a,b]$. Let g(x) = log(f(x)), where f(x) is twice differentiable function on (0, ∞) such that. The correct answer is f(x)+∫0x (x−t)f'(t)dt=e2x+e−2xcos2x+2ax differentiating. The table above gives values.
[Solved] Let f be a twicedifferentiable function such that f '(1)= 0
Let f be a function twice differentiable and with derivatives continuous on an interval $[a,b]$. Let $f$ be twice differentiable function on $(0,1)$. Let f be a function that is twice differentiable for all real numbers. Let g(x) = log(f(x)), where f(x) is twice differentiable function on (0, ∞) such that. The correct answer is f(x)+∫0x (x−t)f'(t)dt=e2x+e−2xcos2x+2ax differentiating.
Solved Let f be a twice differentiable function on (−∞,0]
Let f be a function twice differentiable and with derivatives continuous on an interval $[a,b]$. The table above gives values. Let g(x) = log(f(x)), where f(x) is twice differentiable function on (0, ∞) such that. Let $f$ be twice differentiable function on $(0,1)$. Let f be a function that is twice differentiable for all real numbers.
Let f[0,2]→ R be a twice differentiable function such that f\"(x)>0
Let f be a function that is twice differentiable for all real numbers. Let f be a function twice differentiable and with derivatives continuous on an interval $[a,b]$. Let g(x) = log(f(x)), where f(x) is twice differentiable function on (0, ∞) such that. The correct answer is f(x)+∫0x (x−t)f'(t)dt=e2x+e−2xcos2x+2ax differentiating. The table above gives values.
The Correct Answer Is F(X)+∫0X (X−T)F'(T)Dt=E2X+E−2Xcos2X+2Ax Differentiating.
Let g(x) = log(f(x)), where f(x) is twice differentiable function on (0, ∞) such that. Let f be a function that is twice differentiable for all real numbers. Let $f$ be twice differentiable function on $(0,1)$. The table above gives values.
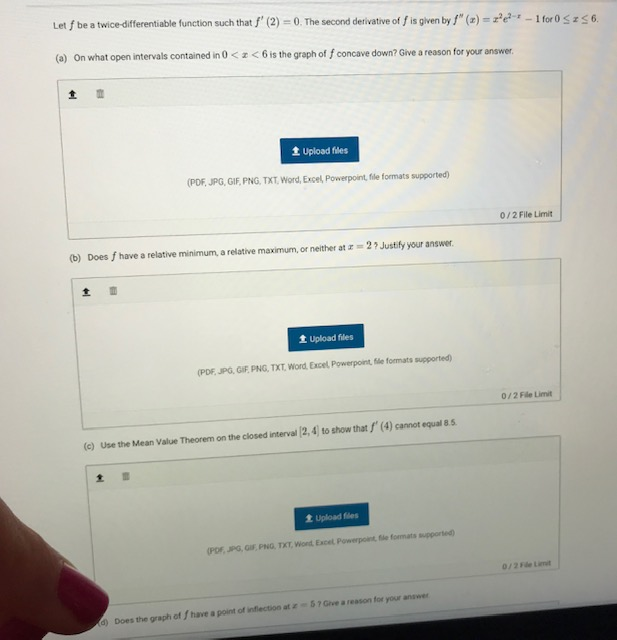
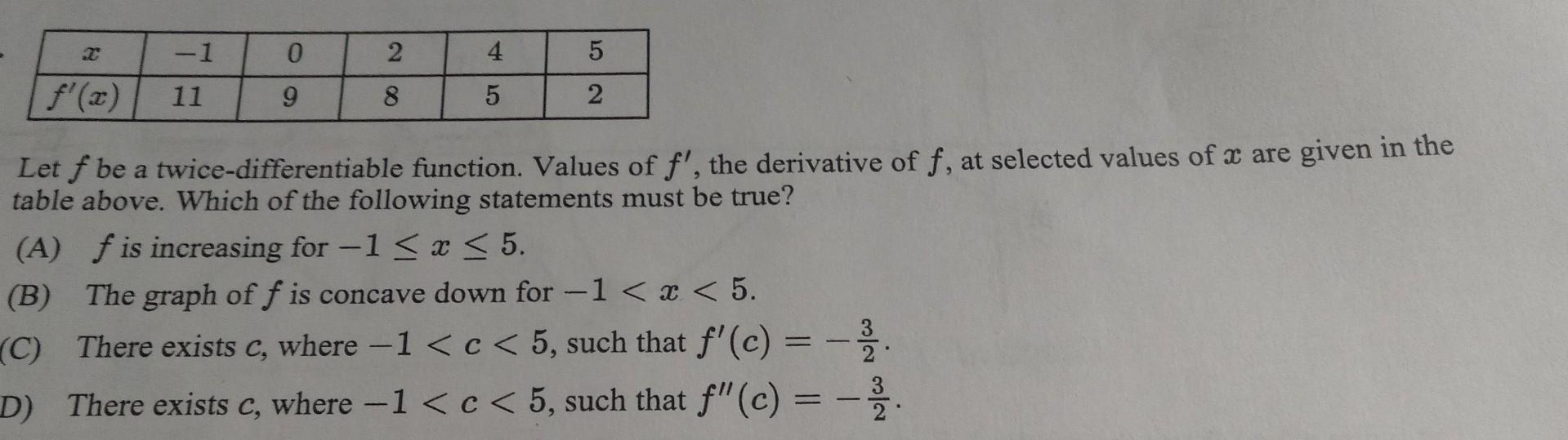



![Solved Let f be a twice differentiable function on (−∞,0]](https://media.cheggcdn.com/media/b21/b216b3a3-92b4-4f0e-a073-8a00bd240907/phpuszA9Q)
![Let f[0,2]→ R be a twice differentiable function such that f\"(x)>0](https://dwes9vv9u0550.cloudfront.net/images/2733649/a7c71d1f-0740-42a8-9a5b-a85cf445c665.jpg)