Inhomogeneous First Order Differential Equation - Solutions to linear first order ode’s 1. The essential difference between first and second order equations is that for first order equations. A first order linear equation is homogeneous if the right hand side is zero: Variation of parameters can be used to solve 2nd order odes, whereas if does not generalize. An example of a first order linear non. First order linear equations in the previous session we. In order to be able to investigate these precisely we first express them in a standard way. X ̇ + p(t)x = 0.
An example of a first order linear non. X ̇ + p(t)x = 0. Variation of parameters can be used to solve 2nd order odes, whereas if does not generalize. In order to be able to investigate these precisely we first express them in a standard way. The essential difference between first and second order equations is that for first order equations. Solutions to linear first order ode’s 1. First order linear equations in the previous session we. A first order linear equation is homogeneous if the right hand side is zero:
Variation of parameters can be used to solve 2nd order odes, whereas if does not generalize. The essential difference between first and second order equations is that for first order equations. Solutions to linear first order ode’s 1. A first order linear equation is homogeneous if the right hand side is zero: X ̇ + p(t)x = 0. In order to be able to investigate these precisely we first express them in a standard way. First order linear equations in the previous session we. An example of a first order linear non.
SOLUTION Differential equation homogeneous first order example 2
In order to be able to investigate these precisely we first express them in a standard way. The essential difference between first and second order equations is that for first order equations. An example of a first order linear non. A first order linear equation is homogeneous if the right hand side is zero: Solutions to linear first order ode’s.
(PDF) Digital Film Music Creation Model Based on Inhomogeneous First
Solutions to linear first order ode’s 1. In order to be able to investigate these precisely we first express them in a standard way. Variation of parameters can be used to solve 2nd order odes, whereas if does not generalize. An example of a first order linear non. X ̇ + p(t)x = 0.
The second order inhomogeneous differential StudyX
X ̇ + p(t)x = 0. Variation of parameters can be used to solve 2nd order odes, whereas if does not generalize. Solutions to linear first order ode’s 1. The essential difference between first and second order equations is that for first order equations. In order to be able to investigate these precisely we first express them in a standard.
Homogeneous Differential Equation First Order Lesson 4 Homogeneous
An example of a first order linear non. A first order linear equation is homogeneous if the right hand side is zero: Solutions to linear first order ode’s 1. First order linear equations in the previous session we. The essential difference between first and second order equations is that for first order equations.
Second Order Inhomogeneous Differential Equations
In order to be able to investigate these precisely we first express them in a standard way. First order linear equations in the previous session we. X ̇ + p(t)x = 0. The essential difference between first and second order equations is that for first order equations. Solutions to linear first order ode’s 1.
Inhomogeneous second order differential equation question r/askmath
Solutions to linear first order ode’s 1. The essential difference between first and second order equations is that for first order equations. X ̇ + p(t)x = 0. In order to be able to investigate these precisely we first express them in a standard way. An example of a first order linear non.
[Solved] 1. A firstorder differential equation is homogenous if it can
A first order linear equation is homogeneous if the right hand side is zero: An example of a first order linear non. Variation of parameters can be used to solve 2nd order odes, whereas if does not generalize. X ̇ + p(t)x = 0. Solutions to linear first order ode’s 1.
Solved Consider the inhomogeneous partial differential
In order to be able to investigate these precisely we first express them in a standard way. Variation of parameters can be used to solve 2nd order odes, whereas if does not generalize. X ̇ + p(t)x = 0. Solutions to linear first order ode’s 1. The essential difference between first and second order equations is that for first order.
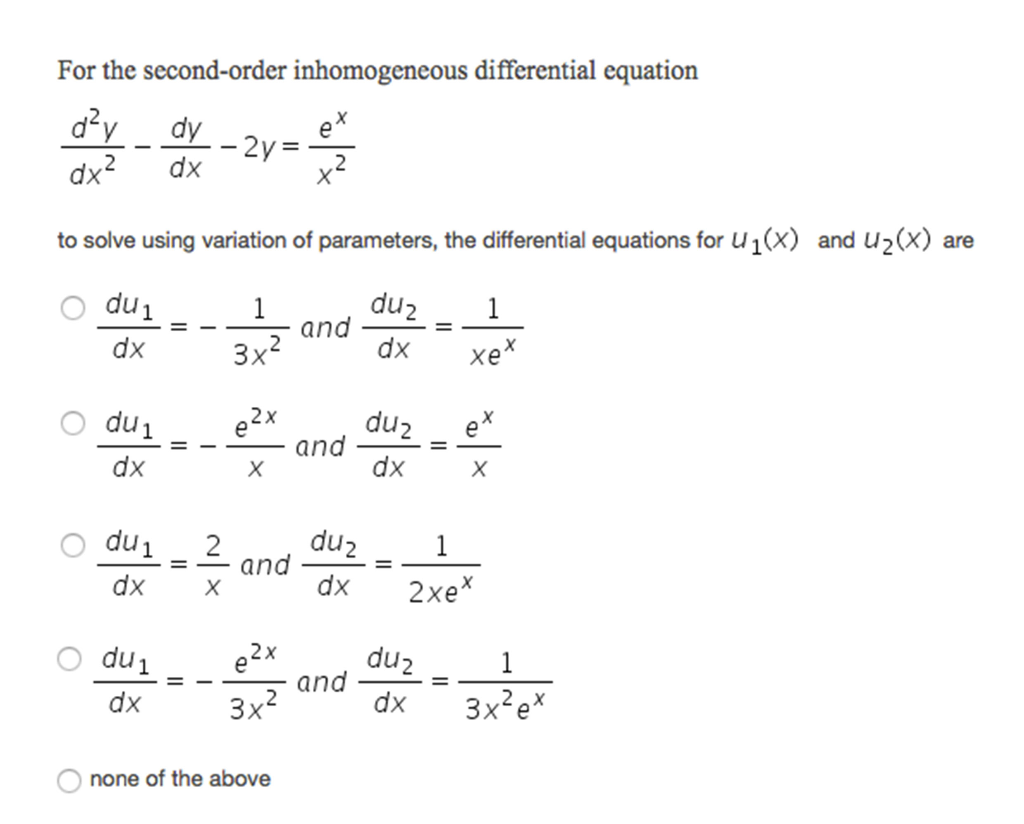
Solved For the secondorder inhomogeneous differential
A first order linear equation is homogeneous if the right hand side is zero: An example of a first order linear non. The essential difference between first and second order equations is that for first order equations. Solutions to linear first order ode’s 1. In order to be able to investigate these precisely we first express them in a standard.
Differential Equation Calculator
A first order linear equation is homogeneous if the right hand side is zero: An example of a first order linear non. X ̇ + p(t)x = 0. First order linear equations in the previous session we. The essential difference between first and second order equations is that for first order equations.
In Order To Be Able To Investigate These Precisely We First Express Them In A Standard Way.
An example of a first order linear non. A first order linear equation is homogeneous if the right hand side is zero: Solutions to linear first order ode’s 1. First order linear equations in the previous session we.
Variation Of Parameters Can Be Used To Solve 2Nd Order Odes, Whereas If Does Not Generalize.
X ̇ + p(t)x = 0. The essential difference between first and second order equations is that for first order equations.