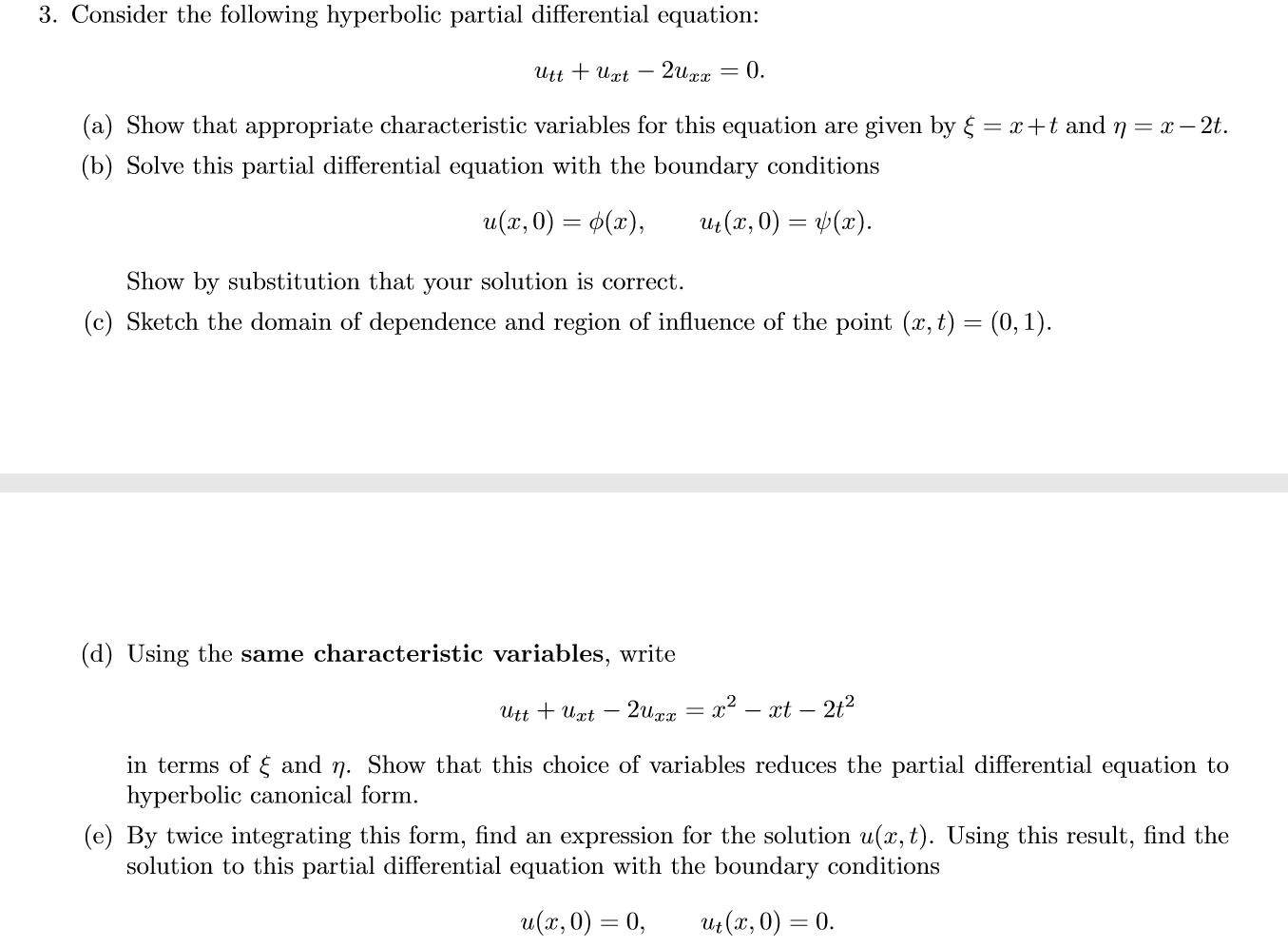
Hyperbolic Differential Equation

Hyperbolic Differential Equation - The independent variables are x 2 [a; ∂ ∂t u(x,t) + ∂ ∂x f[u(x,t)] = 0, with initial condition u(x,0) = u 0(x) and fa given function of u. The aim of this book is to present hyperbolic partial di?erential equations at an elementary level. If b2 4ac < 0, then the pde is elliptic (steady state). In fact, the required mathematical background is only a third year university. A wave is propagating in an interval from a to b. The theory of hyperbolic equations is a large subject, and its applications are many: If b2 4ac > 0, then the pde is hyperbolic (wave). Consider the convective nonlinear equation: This equation can be solved simply by the method of.

Consider the convective nonlinear equation: The aim of this book is to present hyperbolic partial di?erential equations at an elementary level. The theory of hyperbolic equations is a large subject, and its applications are many: If b2 4ac < 0, then the pde is elliptic (steady state). The independent variables are x 2 [a; A wave is propagating in an interval from a to b. If b2 4ac > 0, then the pde is hyperbolic (wave). This equation can be solved simply by the method of. ∂ ∂t u(x,t) + ∂ ∂x f[u(x,t)] = 0, with initial condition u(x,0) = u 0(x) and fa given function of u. In fact, the required mathematical background is only a third year university.

The aim of this book is to present hyperbolic partial di?erential equations at an elementary level. If b2 4ac < 0, then the pde is elliptic (steady state). ∂ ∂t u(x,t) + ∂ ∂x f[u(x,t)] = 0, with initial condition u(x,0) = u 0(x) and fa given function of u. Consider the convective nonlinear equation: The independent variables are x 2 [a; A wave is propagating in an interval from a to b. In fact, the required mathematical background is only a third year university. If b2 4ac > 0, then the pde is hyperbolic (wave). The theory of hyperbolic equations is a large subject, and its applications are many: This equation can be solved simply by the method of.

How do I solve this differential equation to get expression with

The theory of hyperbolic equations is a large subject, and its applications are many: In fact, the required mathematical background is only a third year university. The aim of this book is to present hyperbolic partial di?erential equations at an elementary level. ∂ ∂t u(x,t) + ∂ ∂x f[u(x,t)] = 0, with initial condition u(x,0) = u 0(x) and fa.

Solution of the Hyperbolic Partial Differential Equation on Graphs and

In fact, the required mathematical background is only a third year university. If b2 4ac > 0, then the pde is hyperbolic (wave). The aim of this book is to present hyperbolic partial di?erential equations at an elementary level. Consider the convective nonlinear equation: ∂ ∂t u(x,t) + ∂ ∂x f[u(x,t)] = 0, with initial condition u(x,0) = u 0(x).

Ulam stability of a hyperbolic partial differential equation

The independent variables are x 2 [a; If b2 4ac < 0, then the pde is elliptic (steady state). ∂ ∂t u(x,t) + ∂ ∂x f[u(x,t)] = 0, with initial condition u(x,0) = u 0(x) and fa given function of u. In fact, the required mathematical background is only a third year university. If b2 4ac > 0, then the.

+ 5 PROBLEM 5 HYPERBOLIC DIFFERENTIAL EQUATION The

A wave is propagating in an interval from a to b. If b2 4ac < 0, then the pde is elliptic (steady state). The independent variables are x 2 [a; In fact, the required mathematical background is only a third year university. The aim of this book is to present hyperbolic partial di?erential equations at an elementary level.

Solved 3. Consider the following hyperbolic partial

The aim of this book is to present hyperbolic partial di?erential equations at an elementary level. Consider the convective nonlinear equation: In fact, the required mathematical background is only a third year university. If b2 4ac < 0, then the pde is elliptic (steady state). The independent variables are x 2 [a;

(PDF) On Hyperbolic Differential Equation with Periodic Control Initial

∂ ∂t u(x,t) + ∂ ∂x f[u(x,t)] = 0, with initial condition u(x,0) = u 0(x) and fa given function of u. In fact, the required mathematical background is only a third year university. If b2 4ac < 0, then the pde is elliptic (steady state). The independent variables are x 2 [a; A wave is propagating in an interval.

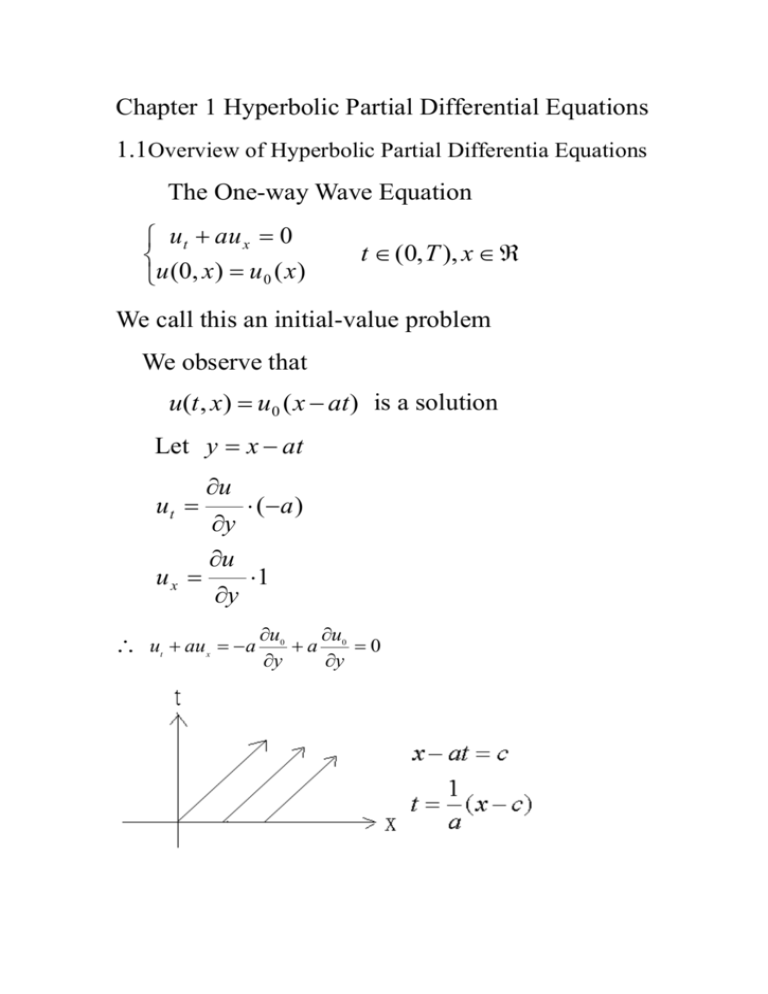
Chapter 1 Hyperbolic Partial Differential Equations

The independent variables are x 2 [a; A wave is propagating in an interval from a to b. This equation can be solved simply by the method of. ∂ ∂t u(x,t) + ∂ ∂x f[u(x,t)] = 0, with initial condition u(x,0) = u 0(x) and fa given function of u. If b2 4ac < 0, then the pde is elliptic.

Numerical Solution of Hyperbolic Differential Equation Nova Science

In fact, the required mathematical background is only a third year university. The independent variables are x 2 [a; The theory of hyperbolic equations is a large subject, and its applications are many: This equation can be solved simply by the method of. ∂ ∂t u(x,t) + ∂ ∂x f[u(x,t)] = 0, with initial condition u(x,0) = u 0(x) and.

[Calc 2] Hyperbolic differential equation learnmath

A wave is propagating in an interval from a to b. In fact, the required mathematical background is only a third year university. This equation can be solved simply by the method of. The independent variables are x 2 [a; Consider the convective nonlinear equation:

Hyperbolic Geometry

∂ ∂t u(x,t) + ∂ ∂x f[u(x,t)] = 0, with initial condition u(x,0) = u 0(x) and fa given function of u. The independent variables are x 2 [a; In fact, the required mathematical background is only a third year university. A wave is propagating in an interval from a to b. The theory of hyperbolic equations is a large.

In Fact, The Required Mathematical Background Is Only A Third Year University.

The theory of hyperbolic equations is a large subject, and its applications are many: If b2 4ac < 0, then the pde is elliptic (steady state). Consider the convective nonlinear equation: This equation can be solved simply by the method of.

The Aim Of This Book Is To Present Hyperbolic Partial Di?Erential Equations At An Elementary Level.

A wave is propagating in an interval from a to b. The independent variables are x 2 [a; If b2 4ac > 0, then the pde is hyperbolic (wave). ∂ ∂t u(x,t) + ∂ ∂x f[u(x,t)] = 0, with initial condition u(x,0) = u 0(x) and fa given function of u.