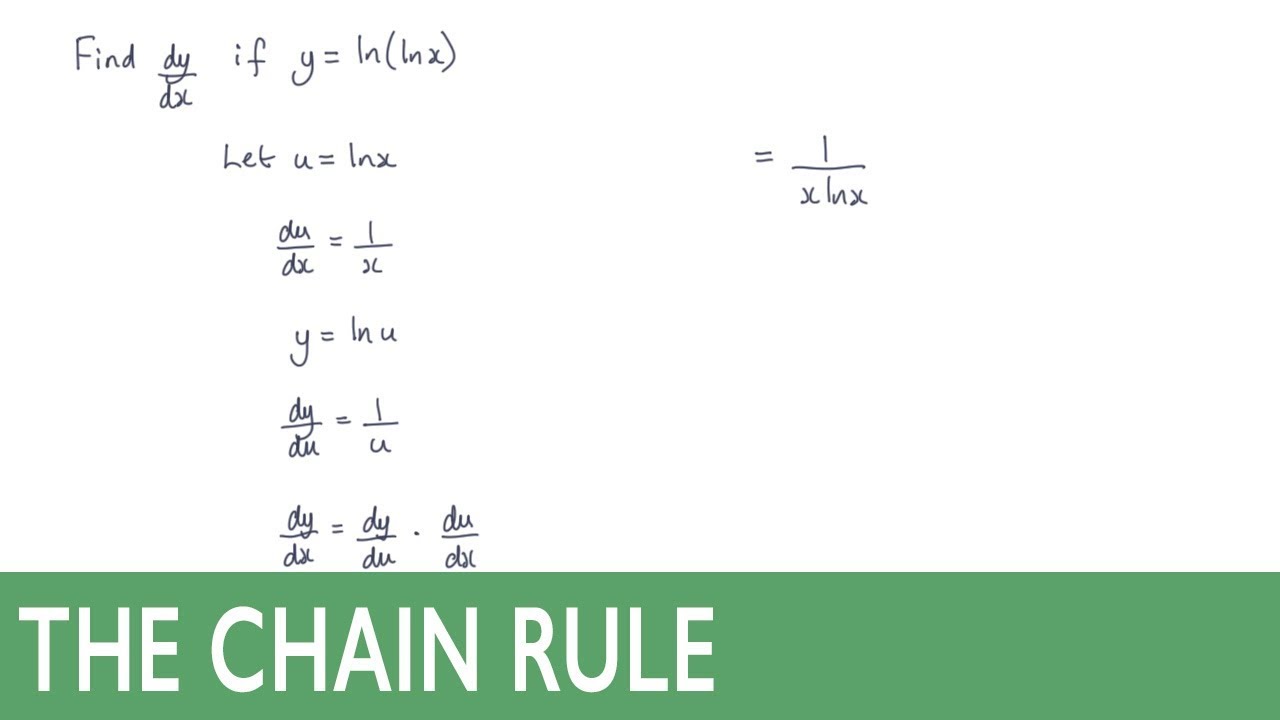
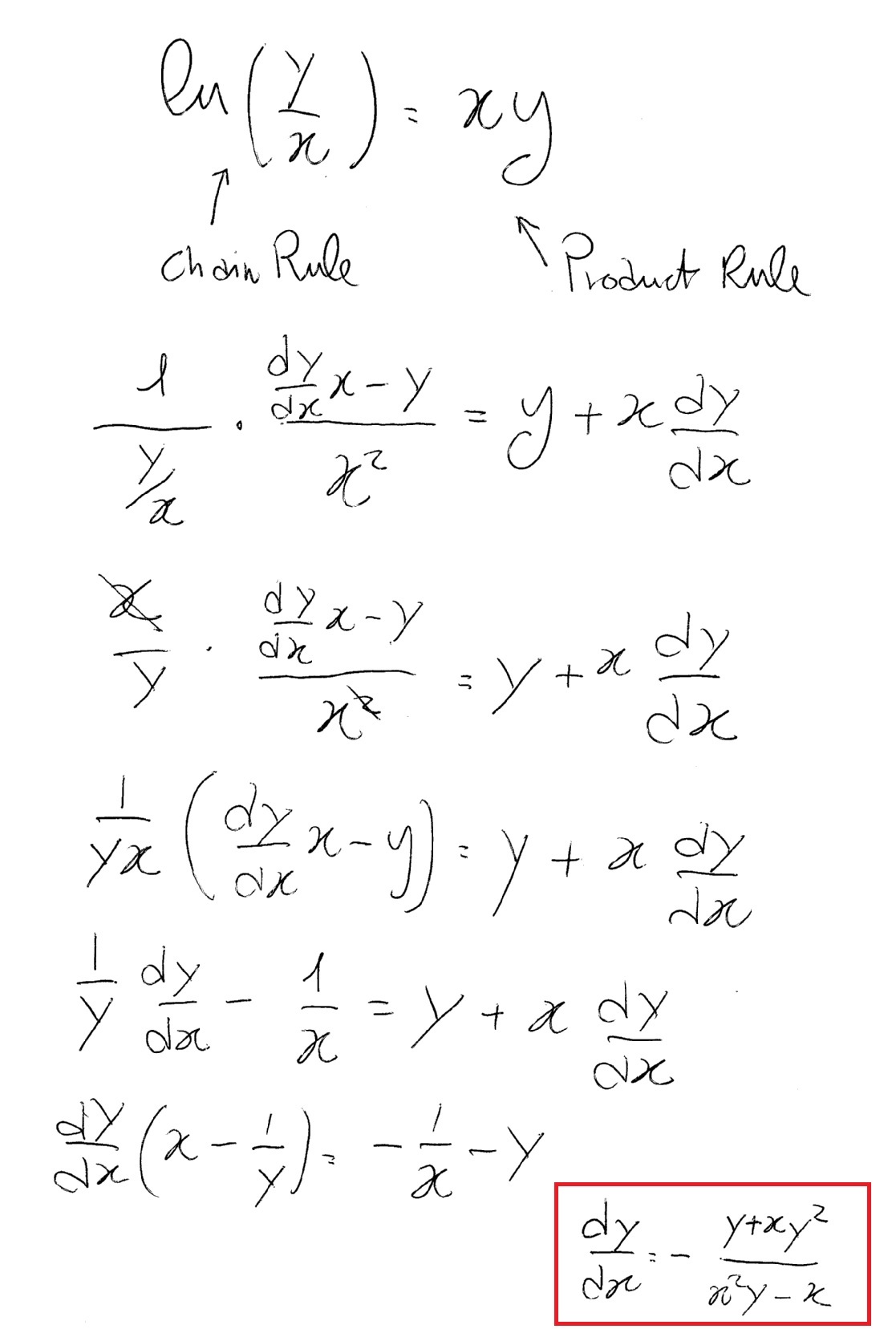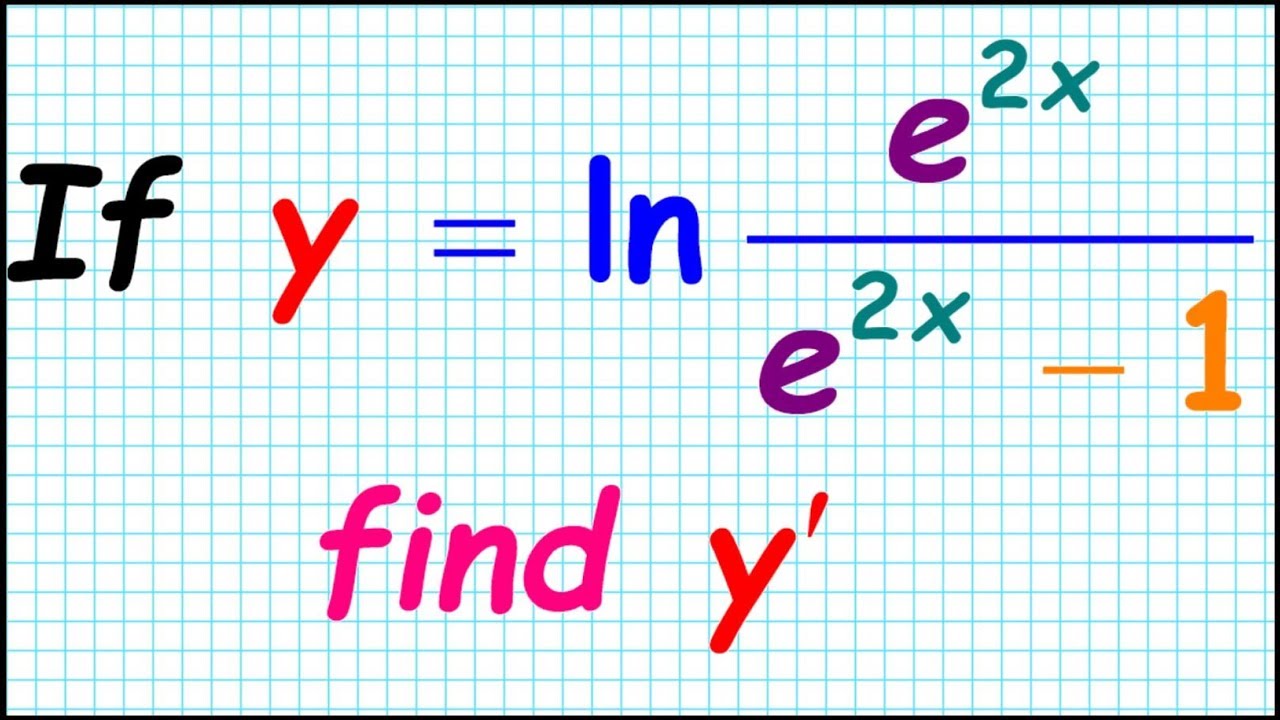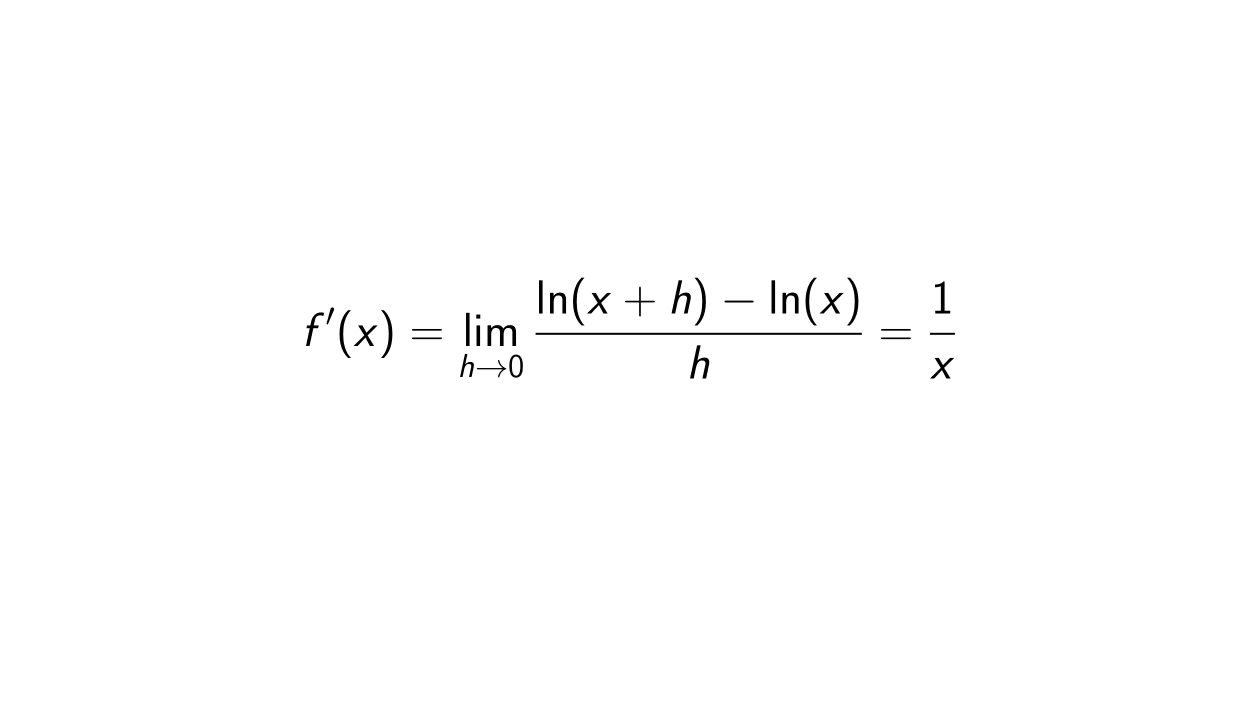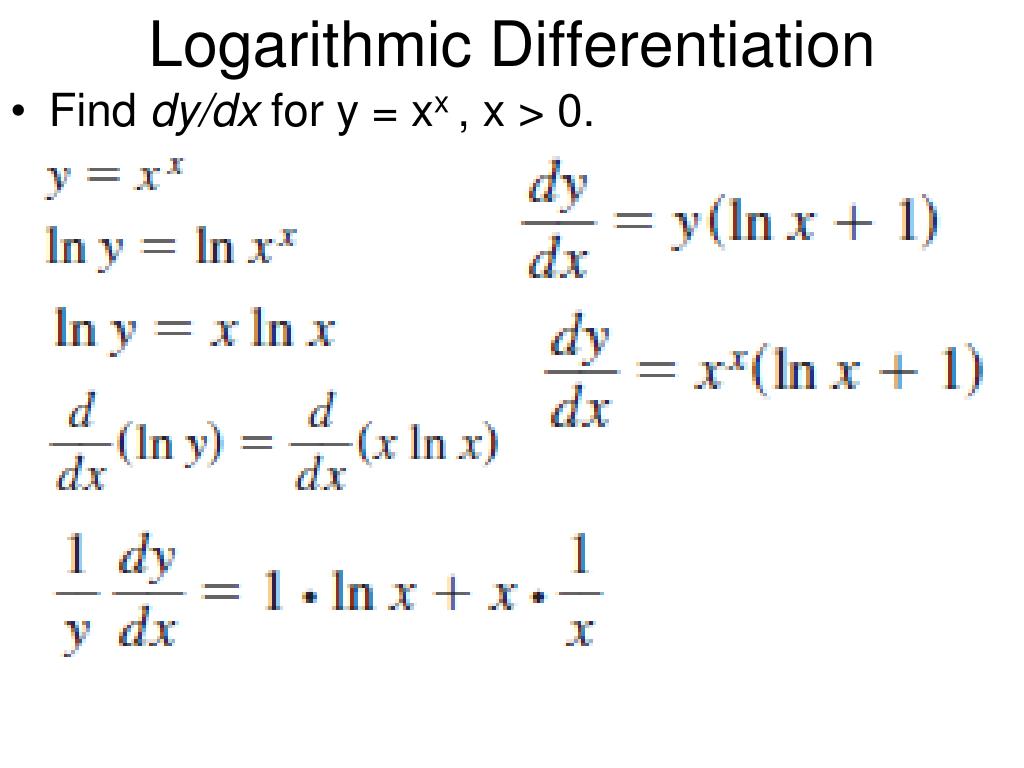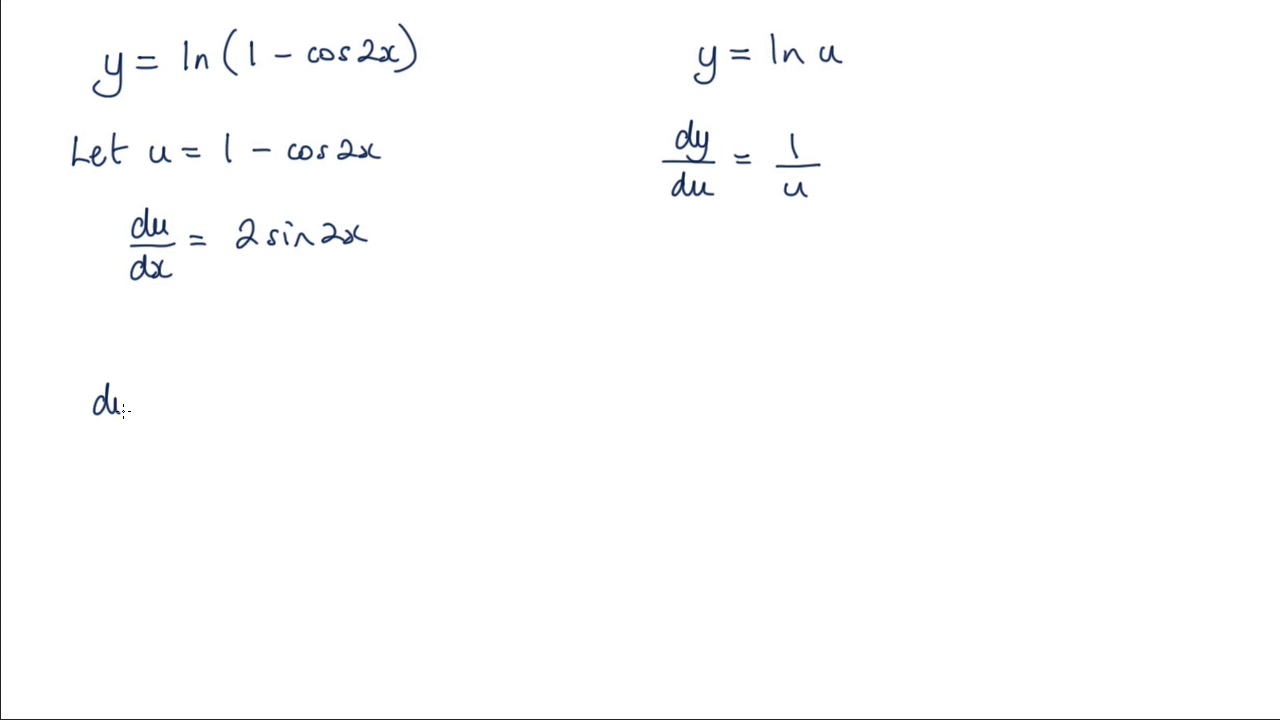How To Differentiate Ln X - We can use a formula to find the derivative of \(y=\ln x\), and the relationship \(log_bx=\frac{\ln x}{\ln b}\) allows us to extend. To find the derivative of ln x, we can use the definition of the derivative and the properties of logarithmic functions.
We can use a formula to find the derivative of \(y=\ln x\), and the relationship \(log_bx=\frac{\ln x}{\ln b}\) allows us to extend. To find the derivative of ln x, we can use the definition of the derivative and the properties of logarithmic functions.
We can use a formula to find the derivative of \(y=\ln x\), and the relationship \(log_bx=\frac{\ln x}{\ln b}\) allows us to extend. To find the derivative of ln x, we can use the definition of the derivative and the properties of logarithmic functions.
Differentiate Ln X
We can use a formula to find the derivative of \(y=\ln x\), and the relationship \(log_bx=\frac{\ln x}{\ln b}\) allows us to extend. To find the derivative of ln x, we can use the definition of the derivative and the properties of logarithmic functions.
Differentiate Ln X
We can use a formula to find the derivative of \(y=\ln x\), and the relationship \(log_bx=\frac{\ln x}{\ln b}\) allows us to extend. To find the derivative of ln x, we can use the definition of the derivative and the properties of logarithmic functions.
Differentiate Ln X
We can use a formula to find the derivative of \(y=\ln x\), and the relationship \(log_bx=\frac{\ln x}{\ln b}\) allows us to extend. To find the derivative of ln x, we can use the definition of the derivative and the properties of logarithmic functions.
Differentiate Ln X
We can use a formula to find the derivative of \(y=\ln x\), and the relationship \(log_bx=\frac{\ln x}{\ln b}\) allows us to extend. To find the derivative of ln x, we can use the definition of the derivative and the properties of logarithmic functions.
Differentiate Ln X
To find the derivative of ln x, we can use the definition of the derivative and the properties of logarithmic functions. We can use a formula to find the derivative of \(y=\ln x\), and the relationship \(log_bx=\frac{\ln x}{\ln b}\) allows us to extend.
Differentiate Ln X
We can use a formula to find the derivative of \(y=\ln x\), and the relationship \(log_bx=\frac{\ln x}{\ln b}\) allows us to extend. To find the derivative of ln x, we can use the definition of the derivative and the properties of logarithmic functions.
Differentiate Ln X
We can use a formula to find the derivative of \(y=\ln x\), and the relationship \(log_bx=\frac{\ln x}{\ln b}\) allows us to extend. To find the derivative of ln x, we can use the definition of the derivative and the properties of logarithmic functions.
Differentiate Ln X
To find the derivative of ln x, we can use the definition of the derivative and the properties of logarithmic functions. We can use a formula to find the derivative of \(y=\ln x\), and the relationship \(log_bx=\frac{\ln x}{\ln b}\) allows us to extend.
Differentiate Ln X
We can use a formula to find the derivative of \(y=\ln x\), and the relationship \(log_bx=\frac{\ln x}{\ln b}\) allows us to extend. To find the derivative of ln x, we can use the definition of the derivative and the properties of logarithmic functions.
Differentiate Ln X
To find the derivative of ln x, we can use the definition of the derivative and the properties of logarithmic functions. We can use a formula to find the derivative of \(y=\ln x\), and the relationship \(log_bx=\frac{\ln x}{\ln b}\) allows us to extend.
We Can Use A Formula To Find The Derivative Of \(Y=\Ln X\), And The Relationship \(Log_Bx=\Frac{\Ln X}{\Ln B}\) Allows Us To Extend.
To find the derivative of ln x, we can use the definition of the derivative and the properties of logarithmic functions.