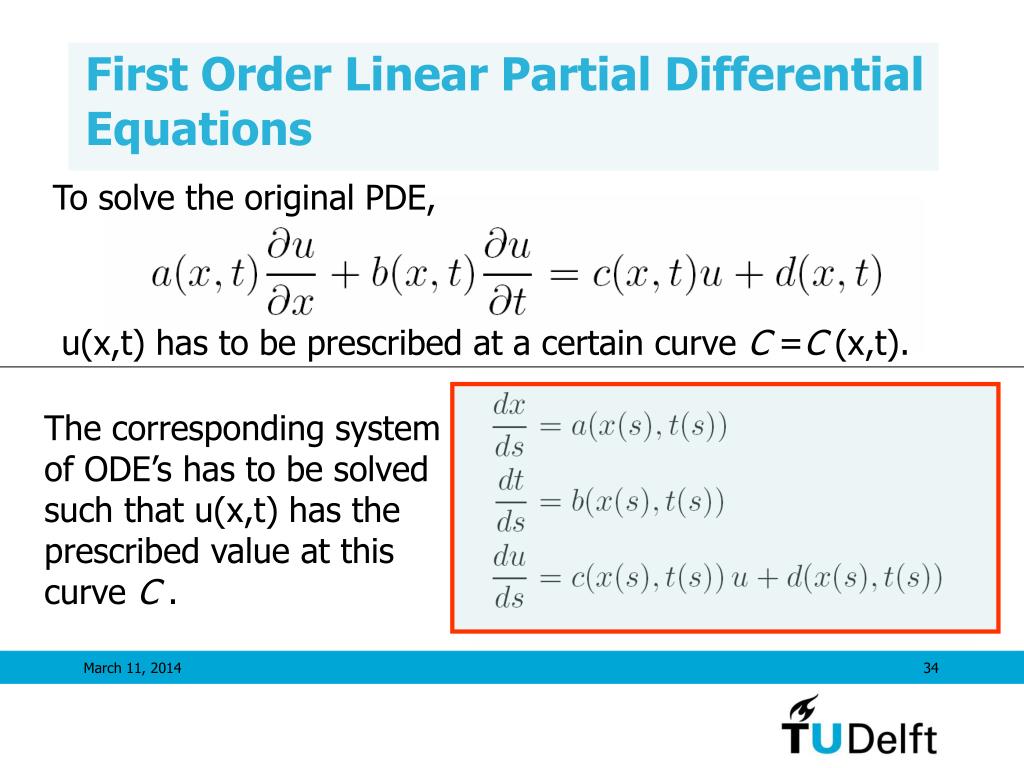
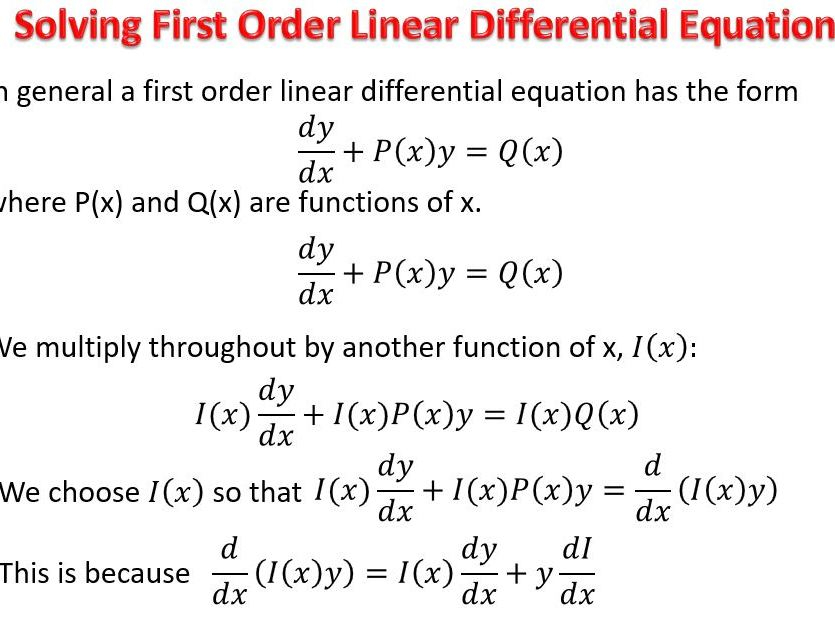
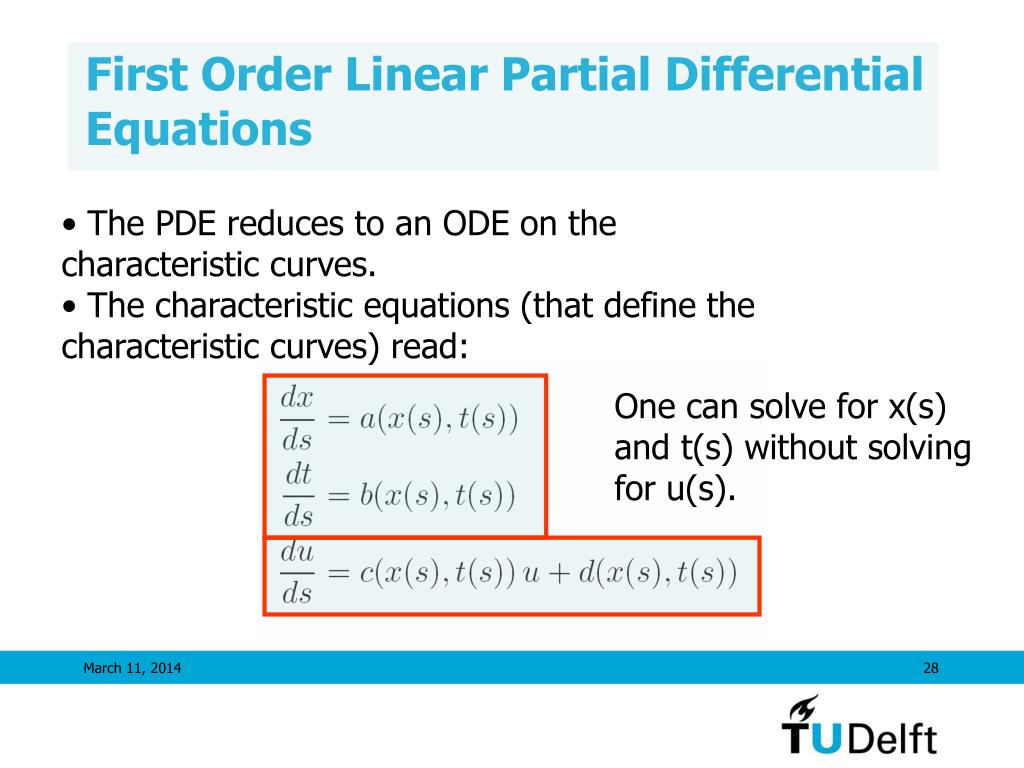
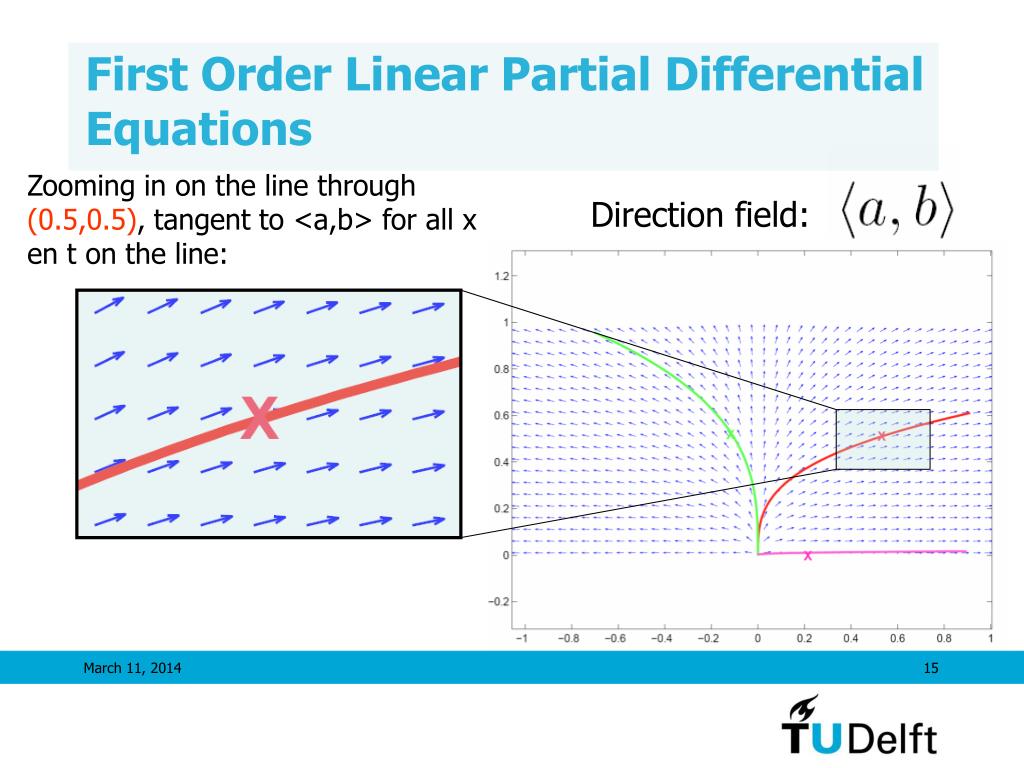
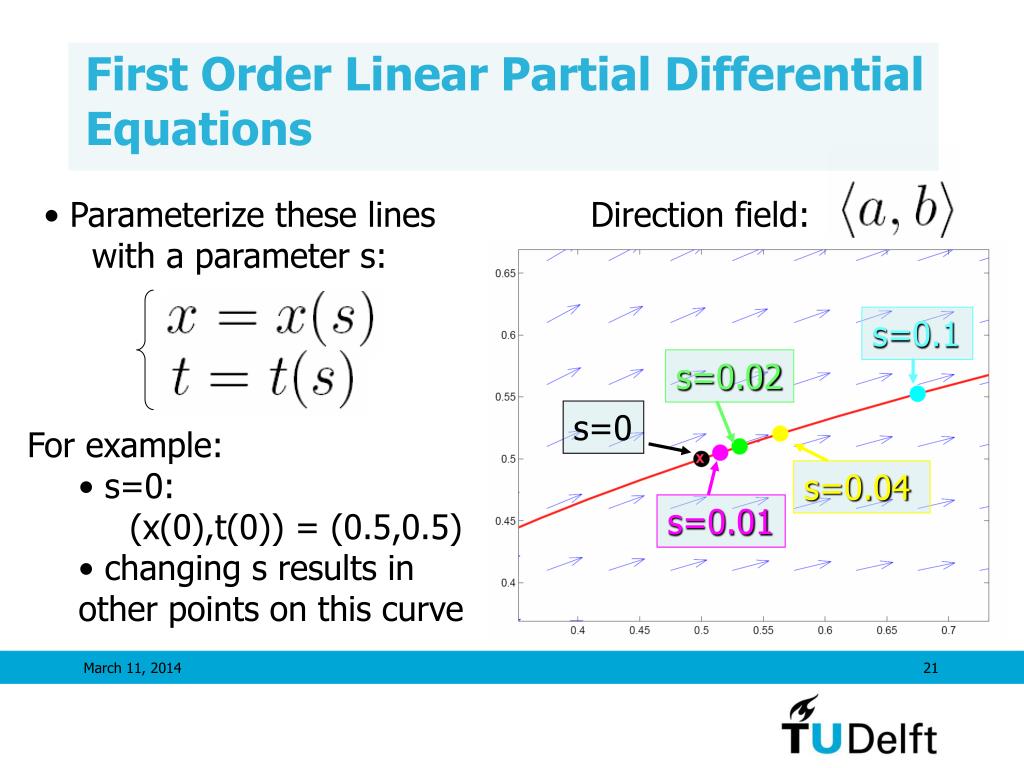
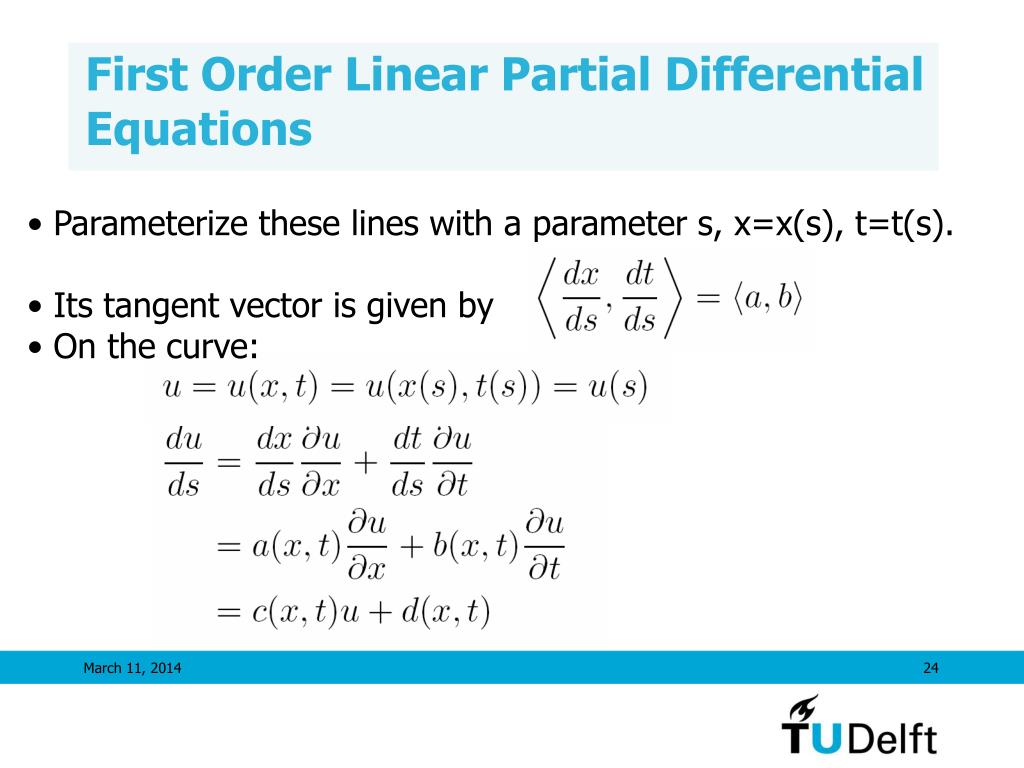
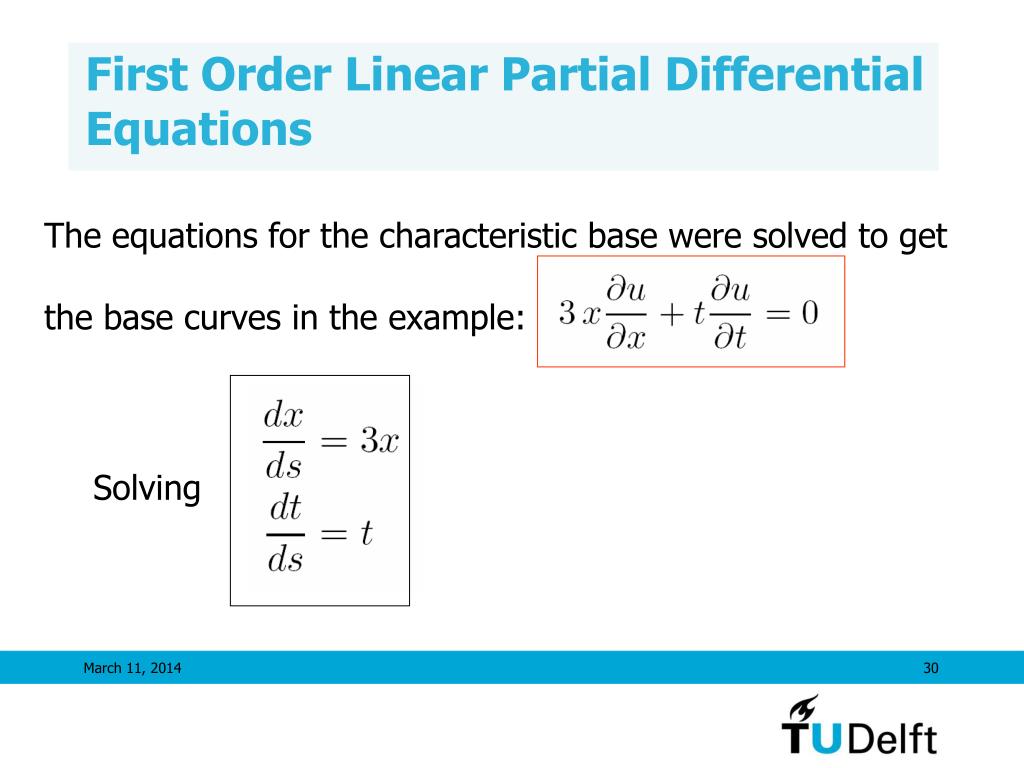
First Order Partial Differential Equations - Consider a first order pde of the form a(x,y) ∂u ∂x +b(x,y) ∂u ∂y = c(x,y,u). We have spent time solving quasilinear first order partial differential equations. We are concerned in the course with partial di erential equations with one dependent variable z and mostly two independent variables x and y. See examples of linear, semilinear, quasilinear. Before doing so, we need to define a few terms. We now turn to nonlinear first order equations of the form \[f(x,. (5) when a(x,y) and b(x,y) are constants, a linear change of variables can. We begin our study of partial differential equations with first order partial differential equations.
Before doing so, we need to define a few terms. We now turn to nonlinear first order equations of the form \[f(x,. (5) when a(x,y) and b(x,y) are constants, a linear change of variables can. We are concerned in the course with partial di erential equations with one dependent variable z and mostly two independent variables x and y. See examples of linear, semilinear, quasilinear. We have spent time solving quasilinear first order partial differential equations. We begin our study of partial differential equations with first order partial differential equations. Consider a first order pde of the form a(x,y) ∂u ∂x +b(x,y) ∂u ∂y = c(x,y,u).
We begin our study of partial differential equations with first order partial differential equations. We are concerned in the course with partial di erential equations with one dependent variable z and mostly two independent variables x and y. (5) when a(x,y) and b(x,y) are constants, a linear change of variables can. Before doing so, we need to define a few terms. We now turn to nonlinear first order equations of the form \[f(x,. We have spent time solving quasilinear first order partial differential equations. See examples of linear, semilinear, quasilinear. Consider a first order pde of the form a(x,y) ∂u ∂x +b(x,y) ∂u ∂y = c(x,y,u).
PPT First Order Partial Differential Equations PowerPoint
(5) when a(x,y) and b(x,y) are constants, a linear change of variables can. We have spent time solving quasilinear first order partial differential equations. Consider a first order pde of the form a(x,y) ∂u ∂x +b(x,y) ∂u ∂y = c(x,y,u). Before doing so, we need to define a few terms. See examples of linear, semilinear, quasilinear.
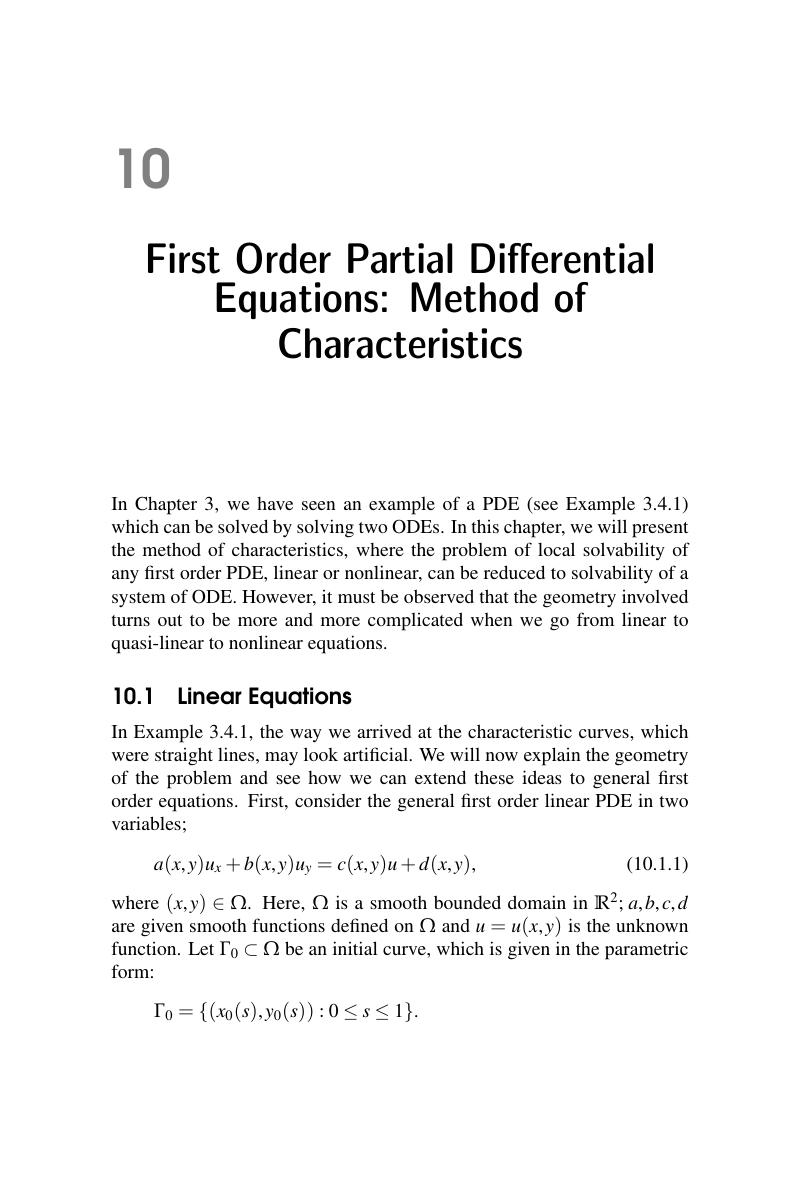
First Order Partial Differential Equations Method of Characteristics
We have spent time solving quasilinear first order partial differential equations. We now turn to nonlinear first order equations of the form \[f(x,. Consider a first order pde of the form a(x,y) ∂u ∂x +b(x,y) ∂u ∂y = c(x,y,u). (5) when a(x,y) and b(x,y) are constants, a linear change of variables can. See examples of linear, semilinear, quasilinear.
PPT First Order Partial Differential Equations PowerPoint
See examples of linear, semilinear, quasilinear. We are concerned in the course with partial di erential equations with one dependent variable z and mostly two independent variables x and y. Before doing so, we need to define a few terms. Consider a first order pde of the form a(x,y) ∂u ∂x +b(x,y) ∂u ∂y = c(x,y,u). We begin our study.
First Order Differential Equation Worksheet Equations Worksheets
Before doing so, we need to define a few terms. (5) when a(x,y) and b(x,y) are constants, a linear change of variables can. We begin our study of partial differential equations with first order partial differential equations. See examples of linear, semilinear, quasilinear. Consider a first order pde of the form a(x,y) ∂u ∂x +b(x,y) ∂u ∂y = c(x,y,u).
PPT First Order Partial Differential Equations PowerPoint
See examples of linear, semilinear, quasilinear. We begin our study of partial differential equations with first order partial differential equations. Consider a first order pde of the form a(x,y) ∂u ∂x +b(x,y) ∂u ∂y = c(x,y,u). Before doing so, we need to define a few terms. We now turn to nonlinear first order equations of the form \[f(x,.
PPT First Order Partial Differential Equations PowerPoint
Consider a first order pde of the form a(x,y) ∂u ∂x +b(x,y) ∂u ∂y = c(x,y,u). We have spent time solving quasilinear first order partial differential equations. See examples of linear, semilinear, quasilinear. We are concerned in the course with partial di erential equations with one dependent variable z and mostly two independent variables x and y. Before doing so,.
PPT First Order Partial Differential Equations PowerPoint
We are concerned in the course with partial di erential equations with one dependent variable z and mostly two independent variables x and y. We have spent time solving quasilinear first order partial differential equations. We begin our study of partial differential equations with first order partial differential equations. We now turn to nonlinear first order equations of the form.
PPT First Order Partial Differential Equations PowerPoint
We now turn to nonlinear first order equations of the form \[f(x,. We have spent time solving quasilinear first order partial differential equations. (5) when a(x,y) and b(x,y) are constants, a linear change of variables can. See examples of linear, semilinear, quasilinear. Consider a first order pde of the form a(x,y) ∂u ∂x +b(x,y) ∂u ∂y = c(x,y,u).
First Order Partial Differential Equations PDF
Consider a first order pde of the form a(x,y) ∂u ∂x +b(x,y) ∂u ∂y = c(x,y,u). We now turn to nonlinear first order equations of the form \[f(x,. We are concerned in the course with partial di erential equations with one dependent variable z and mostly two independent variables x and y. Before doing so, we need to define a.
PPT First Order Partial Differential Equations PowerPoint
We now turn to nonlinear first order equations of the form \[f(x,. Consider a first order pde of the form a(x,y) ∂u ∂x +b(x,y) ∂u ∂y = c(x,y,u). See examples of linear, semilinear, quasilinear. We have spent time solving quasilinear first order partial differential equations. We are concerned in the course with partial di erential equations with one dependent variable.
(5) When A(X,Y) And B(X,Y) Are Constants, A Linear Change Of Variables Can.
Consider a first order pde of the form a(x,y) ∂u ∂x +b(x,y) ∂u ∂y = c(x,y,u). We now turn to nonlinear first order equations of the form \[f(x,. See examples of linear, semilinear, quasilinear. We begin our study of partial differential equations with first order partial differential equations.
We Are Concerned In The Course With Partial Di Erential Equations With One Dependent Variable Z And Mostly Two Independent Variables X And Y.
We have spent time solving quasilinear first order partial differential equations. Before doing so, we need to define a few terms.