Existence Theorem Differential Equations - Notes on the existence and uniqueness theorem for first order differential equations i. The existence and uniqueness of solutions to differential equations 5 theorem 3.9. Then the differential equation (2) with initial con. I!rnis a solution to x_ = v(t;x) with. Whether we are looking for exact solutions or numerical approximations, it is useful to know conditions that imply the existence and. Let the function f(t,y) be continuous and satisfy the bound (3).
Notes on the existence and uniqueness theorem for first order differential equations i. Then the differential equation (2) with initial con. Whether we are looking for exact solutions or numerical approximations, it is useful to know conditions that imply the existence and. I!rnis a solution to x_ = v(t;x) with. Let the function f(t,y) be continuous and satisfy the bound (3). The existence and uniqueness of solutions to differential equations 5 theorem 3.9.
I!rnis a solution to x_ = v(t;x) with. Then the differential equation (2) with initial con. Whether we are looking for exact solutions or numerical approximations, it is useful to know conditions that imply the existence and. Let the function f(t,y) be continuous and satisfy the bound (3). The existence and uniqueness of solutions to differential equations 5 theorem 3.9. Notes on the existence and uniqueness theorem for first order differential equations i.
(PDF) A comparison theorem for solutions of backward stochastic
Let the function f(t,y) be continuous and satisfy the bound (3). Notes on the existence and uniqueness theorem for first order differential equations i. I!rnis a solution to x_ = v(t;x) with. The existence and uniqueness of solutions to differential equations 5 theorem 3.9. Whether we are looking for exact solutions or numerical approximations, it is useful to know conditions.
(PDF) An existence theorem for ordinary differential equations in
I!rnis a solution to x_ = v(t;x) with. The existence and uniqueness of solutions to differential equations 5 theorem 3.9. Let the function f(t,y) be continuous and satisfy the bound (3). Notes on the existence and uniqueness theorem for first order differential equations i. Then the differential equation (2) with initial con.
Lesson 7 Existence And Uniqueness Theorem (Differential Equations
Whether we are looking for exact solutions or numerical approximations, it is useful to know conditions that imply the existence and. Then the differential equation (2) with initial con. The existence and uniqueness of solutions to differential equations 5 theorem 3.9. Let the function f(t,y) be continuous and satisfy the bound (3). I!rnis a solution to x_ = v(t;x) with.
ordinary differential equations Application of Picard's existence
Whether we are looking for exact solutions or numerical approximations, it is useful to know conditions that imply the existence and. Then the differential equation (2) with initial con. The existence and uniqueness of solutions to differential equations 5 theorem 3.9. I!rnis a solution to x_ = v(t;x) with. Let the function f(t,y) be continuous and satisfy the bound (3).
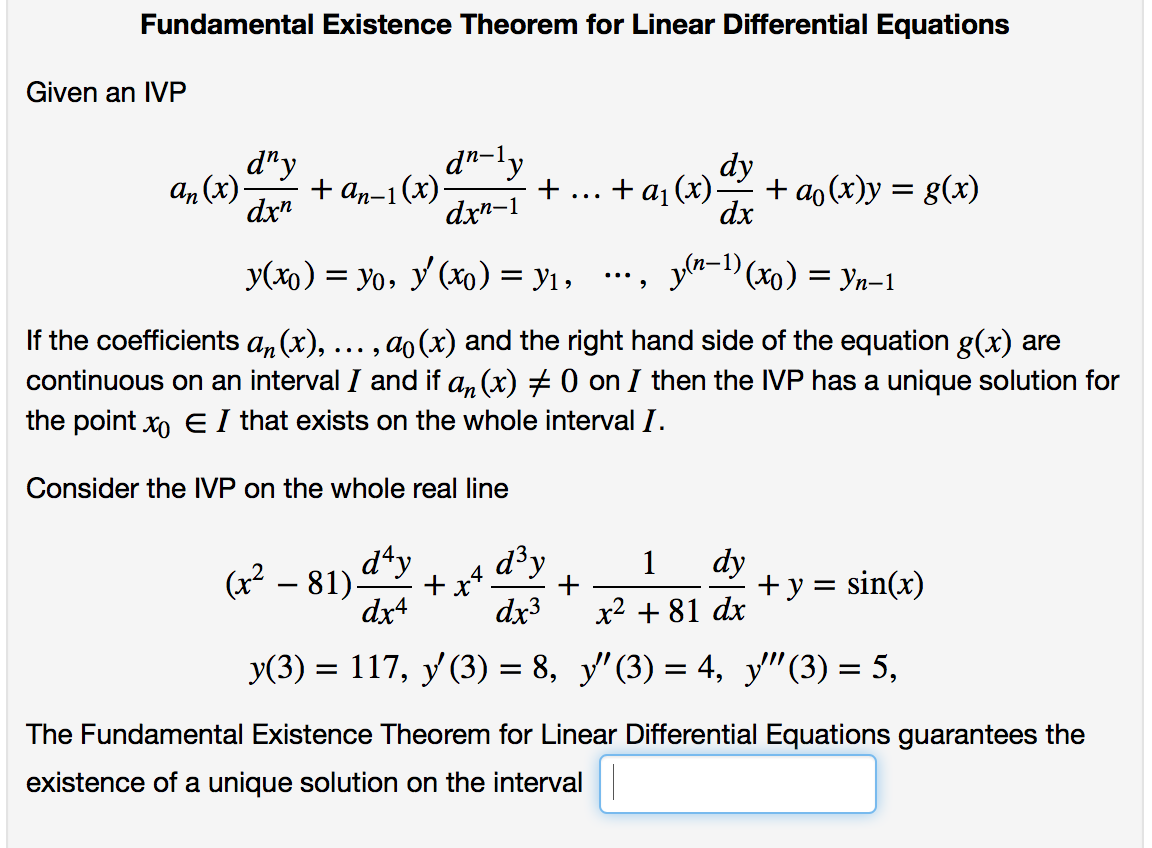
Solved The Fundamental Existence Theorem for Linear
I!rnis a solution to x_ = v(t;x) with. Notes on the existence and uniqueness theorem for first order differential equations i. The existence and uniqueness of solutions to differential equations 5 theorem 3.9. Let the function f(t,y) be continuous and satisfy the bound (3). Then the differential equation (2) with initial con.
SOLUTION Laplace transform and properties of laplace transform and
Notes on the existence and uniqueness theorem for first order differential equations i. The existence and uniqueness of solutions to differential equations 5 theorem 3.9. Let the function f(t,y) be continuous and satisfy the bound (3). I!rnis a solution to x_ = v(t;x) with. Whether we are looking for exact solutions or numerical approximations, it is useful to know conditions.
(PDF) Uniqueness and existence results for ordinary differential equations
Then the differential equation (2) with initial con. Notes on the existence and uniqueness theorem for first order differential equations i. Whether we are looking for exact solutions or numerical approximations, it is useful to know conditions that imply the existence and. The existence and uniqueness of solutions to differential equations 5 theorem 3.9. Let the function f(t,y) be continuous.
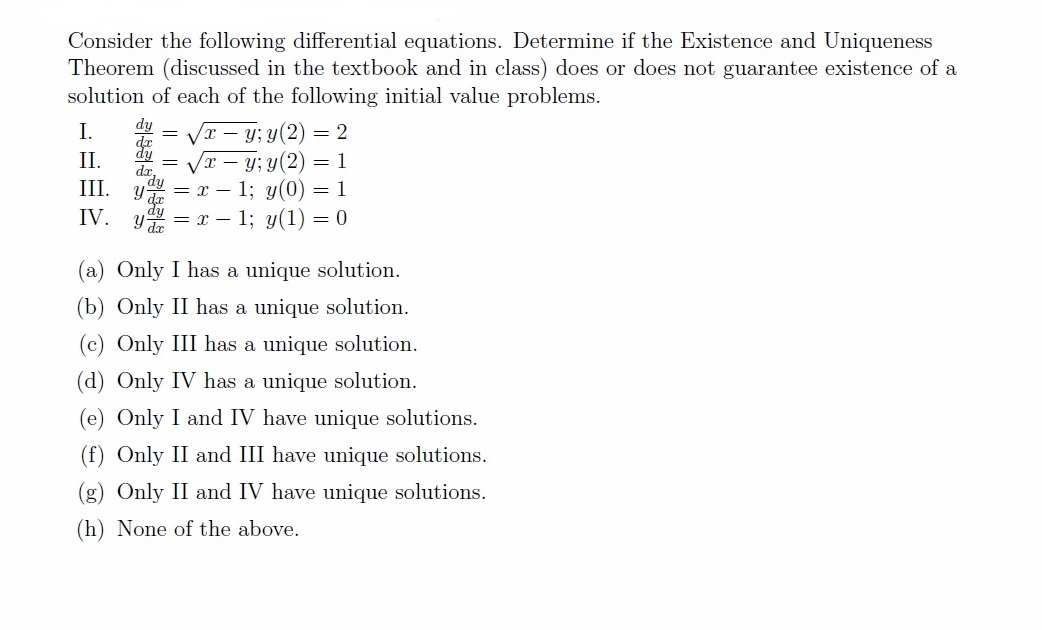
Solved Consider the following differential equations.
The existence and uniqueness of solutions to differential equations 5 theorem 3.9. Notes on the existence and uniqueness theorem for first order differential equations i. Then the differential equation (2) with initial con. I!rnis a solution to x_ = v(t;x) with. Whether we are looking for exact solutions or numerical approximations, it is useful to know conditions that imply the.
Existence theorem NOTES ON THE EXISTENCE AND UNIQUENESS THEOREM FOR
Whether we are looking for exact solutions or numerical approximations, it is useful to know conditions that imply the existence and. The existence and uniqueness of solutions to differential equations 5 theorem 3.9. I!rnis a solution to x_ = v(t;x) with. Let the function f(t,y) be continuous and satisfy the bound (3). Notes on the existence and uniqueness theorem for.
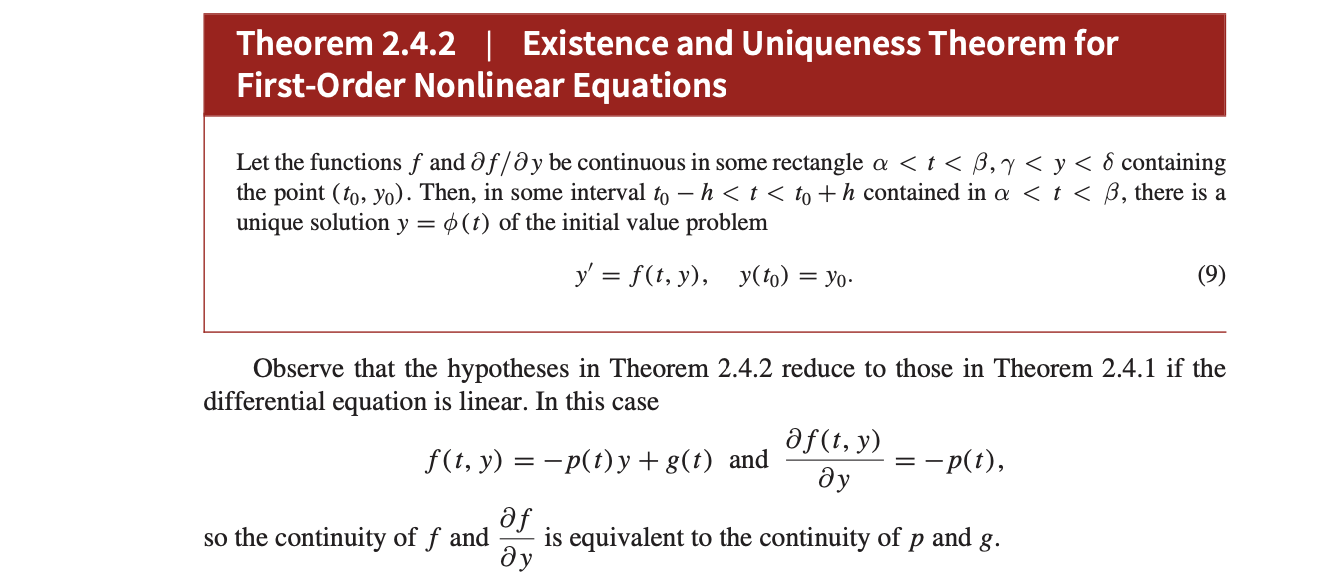
Solved Theorem 2.4.1 Existence and Uniqueness Theorem for
The existence and uniqueness of solutions to differential equations 5 theorem 3.9. Whether we are looking for exact solutions or numerical approximations, it is useful to know conditions that imply the existence and. Notes on the existence and uniqueness theorem for first order differential equations i. Then the differential equation (2) with initial con. I!rnis a solution to x_ =.
The Existence And Uniqueness Of Solutions To Differential Equations 5 Theorem 3.9.
Then the differential equation (2) with initial con. Let the function f(t,y) be continuous and satisfy the bound (3). Whether we are looking for exact solutions or numerical approximations, it is useful to know conditions that imply the existence and. Notes on the existence and uniqueness theorem for first order differential equations i.