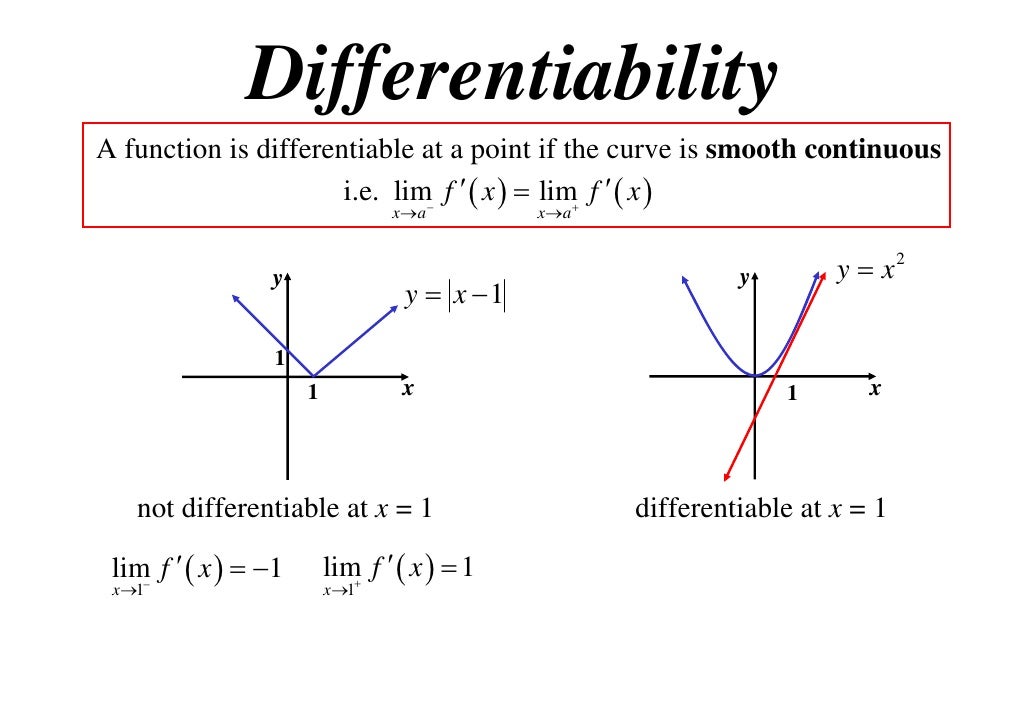
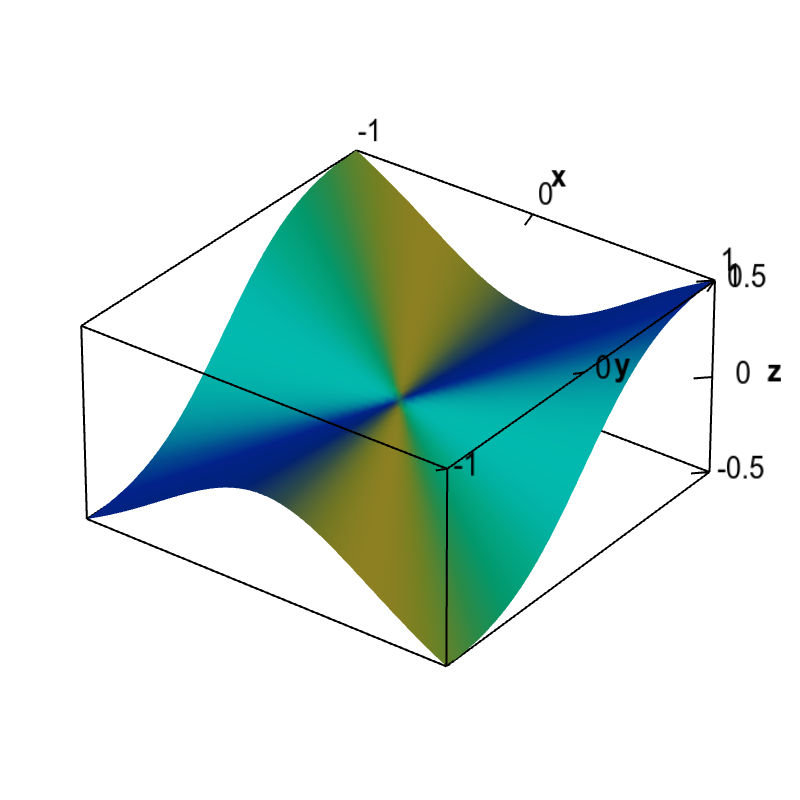Examples Of Not Differentiable - The function jumps at x x, (is not continuous) like. Here are a number of cases in which f does not have a derivative at a point. For example, if there is a. When f is not continuous at x = x 0. We can say that f is not differentiable for any value of x where a tangent cannot 'exist' or the tangent exists but is vertical (vertical line has.
When f is not continuous at x = x 0. Here are a number of cases in which f does not have a derivative at a point. We can say that f is not differentiable for any value of x where a tangent cannot 'exist' or the tangent exists but is vertical (vertical line has. The function jumps at x x, (is not continuous) like. For example, if there is a.
We can say that f is not differentiable for any value of x where a tangent cannot 'exist' or the tangent exists but is vertical (vertical line has. The function jumps at x x, (is not continuous) like. When f is not continuous at x = x 0. Here are a number of cases in which f does not have a derivative at a point. For example, if there is a.
When Is a Function Continuous but Not Differentiable Quant RL
When f is not continuous at x = x 0. The function jumps at x x, (is not continuous) like. We can say that f is not differentiable for any value of x where a tangent cannot 'exist' or the tangent exists but is vertical (vertical line has. Here are a number of cases in which f does not have.
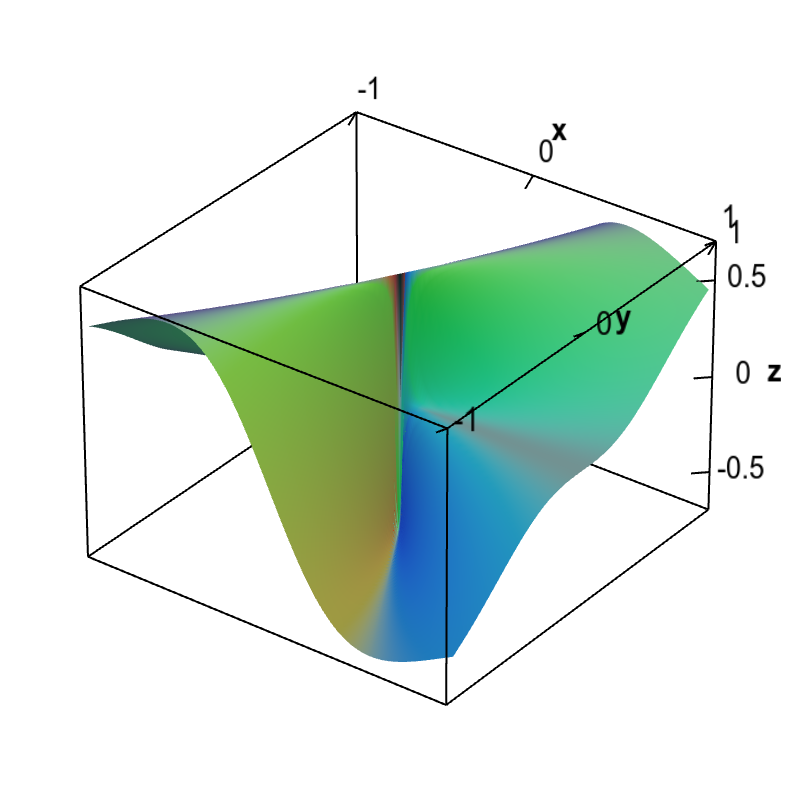
Nondifferentiable functions must have discontinuous partial
The function jumps at x x, (is not continuous) like. We can say that f is not differentiable for any value of x where a tangent cannot 'exist' or the tangent exists but is vertical (vertical line has. Here are a number of cases in which f does not have a derivative at a point. When f is not continuous.
Nondifferentiable functions must have discontinuous partial
Here are a number of cases in which f does not have a derivative at a point. When f is not continuous at x = x 0. For example, if there is a. The function jumps at x x, (is not continuous) like. We can say that f is not differentiable for any value of x where a tangent cannot.
Differentiable Function Meaning, Formulas and Examples Outlier
For example, if there is a. When f is not continuous at x = x 0. We can say that f is not differentiable for any value of x where a tangent cannot 'exist' or the tangent exists but is vertical (vertical line has. Here are a number of cases in which f does not have a derivative at a.
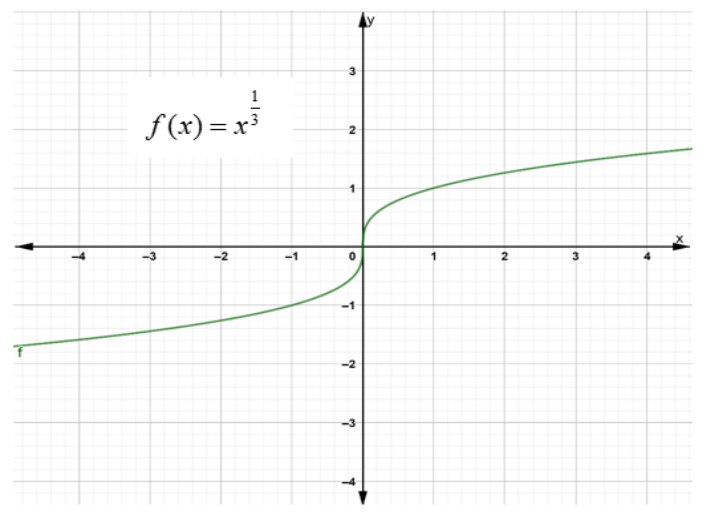
What are some examples of nondifferentiable functions?
Here are a number of cases in which f does not have a derivative at a point. When f is not continuous at x = x 0. We can say that f is not differentiable for any value of x where a tangent cannot 'exist' or the tangent exists but is vertical (vertical line has. The function jumps at x.
Can Something Be Differentiable but Not Continuous Quant RL
When f is not continuous at x = x 0. We can say that f is not differentiable for any value of x where a tangent cannot 'exist' or the tangent exists but is vertical (vertical line has. Here are a number of cases in which f does not have a derivative at a point. The function jumps at x.
Differentiable vs. Continuous Functions Understanding the Distinctions
For example, if there is a. Here are a number of cases in which f does not have a derivative at a point. The function jumps at x x, (is not continuous) like. When f is not continuous at x = x 0. We can say that f is not differentiable for any value of x where a tangent cannot.
Differentiable Cuemath
Here are a number of cases in which f does not have a derivative at a point. The function jumps at x x, (is not continuous) like. We can say that f is not differentiable for any value of x where a tangent cannot 'exist' or the tangent exists but is vertical (vertical line has. For example, if there is.
Can Something Be Differentiable but Not Continuous Quant RL
For example, if there is a. When f is not continuous at x = x 0. Here are a number of cases in which f does not have a derivative at a point. The function jumps at x x, (is not continuous) like. We can say that f is not differentiable for any value of x where a tangent cannot.
SOLVEDWrite a paragraph that explains what it means for a function to
For example, if there is a. The function jumps at x x, (is not continuous) like. Here are a number of cases in which f does not have a derivative at a point. We can say that f is not differentiable for any value of x where a tangent cannot 'exist' or the tangent exists but is vertical (vertical line.
We Can Say That F Is Not Differentiable For Any Value Of X Where A Tangent Cannot 'Exist' Or The Tangent Exists But Is Vertical (Vertical Line Has.
The function jumps at x x, (is not continuous) like. When f is not continuous at x = x 0. For example, if there is a. Here are a number of cases in which f does not have a derivative at a point.