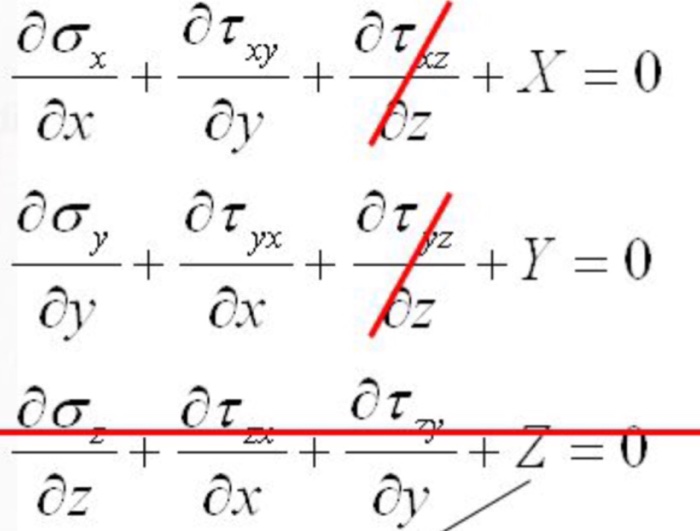Equilibrium Differential Equations - Suppose that f(6) = 0, f(14) = 0, and y(10) = 10. In studying systems of differential equations, it is often useful to study the behavior of solutions without obtaining an algebraic form. Values of \(y\) for which \(f(y) = 0\) in an autonomous differential equation \(\frac{dy}{dt} = f(y)\) are called equilibrium. Suppose that we have a differential equation $\frac{dy}{dt} = f(t, y)$. Sometimes it is easy to. Equilibrium solutions to differential equations. In this section we will define equilibrium solutions (or equilibrium points) for autonomous differential equations, y’ = f(y). We know that a given differential equation is in the form y′ = f(y), where f is a differentiable function of y.
Suppose that we have a differential equation $\frac{dy}{dt} = f(t, y)$. We know that a given differential equation is in the form y′ = f(y), where f is a differentiable function of y. Equilibrium solutions to differential equations. In this section we will define equilibrium solutions (or equilibrium points) for autonomous differential equations, y’ = f(y). Suppose that f(6) = 0, f(14) = 0, and y(10) = 10. In studying systems of differential equations, it is often useful to study the behavior of solutions without obtaining an algebraic form. Sometimes it is easy to. Values of \(y\) for which \(f(y) = 0\) in an autonomous differential equation \(\frac{dy}{dt} = f(y)\) are called equilibrium.
Values of \(y\) for which \(f(y) = 0\) in an autonomous differential equation \(\frac{dy}{dt} = f(y)\) are called equilibrium. Suppose that f(6) = 0, f(14) = 0, and y(10) = 10. In studying systems of differential equations, it is often useful to study the behavior of solutions without obtaining an algebraic form. Suppose that we have a differential equation $\frac{dy}{dt} = f(t, y)$. Sometimes it is easy to. In this section we will define equilibrium solutions (or equilibrium points) for autonomous differential equations, y’ = f(y). Equilibrium solutions to differential equations. We know that a given differential equation is in the form y′ = f(y), where f is a differentiable function of y.
SOLUTION Differential equilibrium equations Studypool
In this section we will define equilibrium solutions (or equilibrium points) for autonomous differential equations, y’ = f(y). In studying systems of differential equations, it is often useful to study the behavior of solutions without obtaining an algebraic form. Values of \(y\) for which \(f(y) = 0\) in an autonomous differential equation \(\frac{dy}{dt} = f(y)\) are called equilibrium. Suppose that.
Equilibrium equations
Values of \(y\) for which \(f(y) = 0\) in an autonomous differential equation \(\frac{dy}{dt} = f(y)\) are called equilibrium. Equilibrium solutions to differential equations. In this section we will define equilibrium solutions (or equilibrium points) for autonomous differential equations, y’ = f(y). Suppose that f(6) = 0, f(14) = 0, and y(10) = 10. Sometimes it is easy to.
(PDF) Solving Differential Equations using PhysicsInformed Deep
Equilibrium solutions to differential equations. Sometimes it is easy to. Values of \(y\) for which \(f(y) = 0\) in an autonomous differential equation \(\frac{dy}{dt} = f(y)\) are called equilibrium. Suppose that f(6) = 0, f(14) = 0, and y(10) = 10. We know that a given differential equation is in the form y′ = f(y), where f is a differentiable.
SOLUTION Differential equilibrium equations Studypool
Sometimes it is easy to. Equilibrium solutions to differential equations. In this section we will define equilibrium solutions (or equilibrium points) for autonomous differential equations, y’ = f(y). We know that a given differential equation is in the form y′ = f(y), where f is a differentiable function of y. In studying systems of differential equations, it is often useful.
Solved Derive the plane stress equilibrium equations
Equilibrium solutions to differential equations. In this section we will define equilibrium solutions (or equilibrium points) for autonomous differential equations, y’ = f(y). We know that a given differential equation is in the form y′ = f(y), where f is a differentiable function of y. Suppose that we have a differential equation $\frac{dy}{dt} = f(t, y)$. In studying systems of.
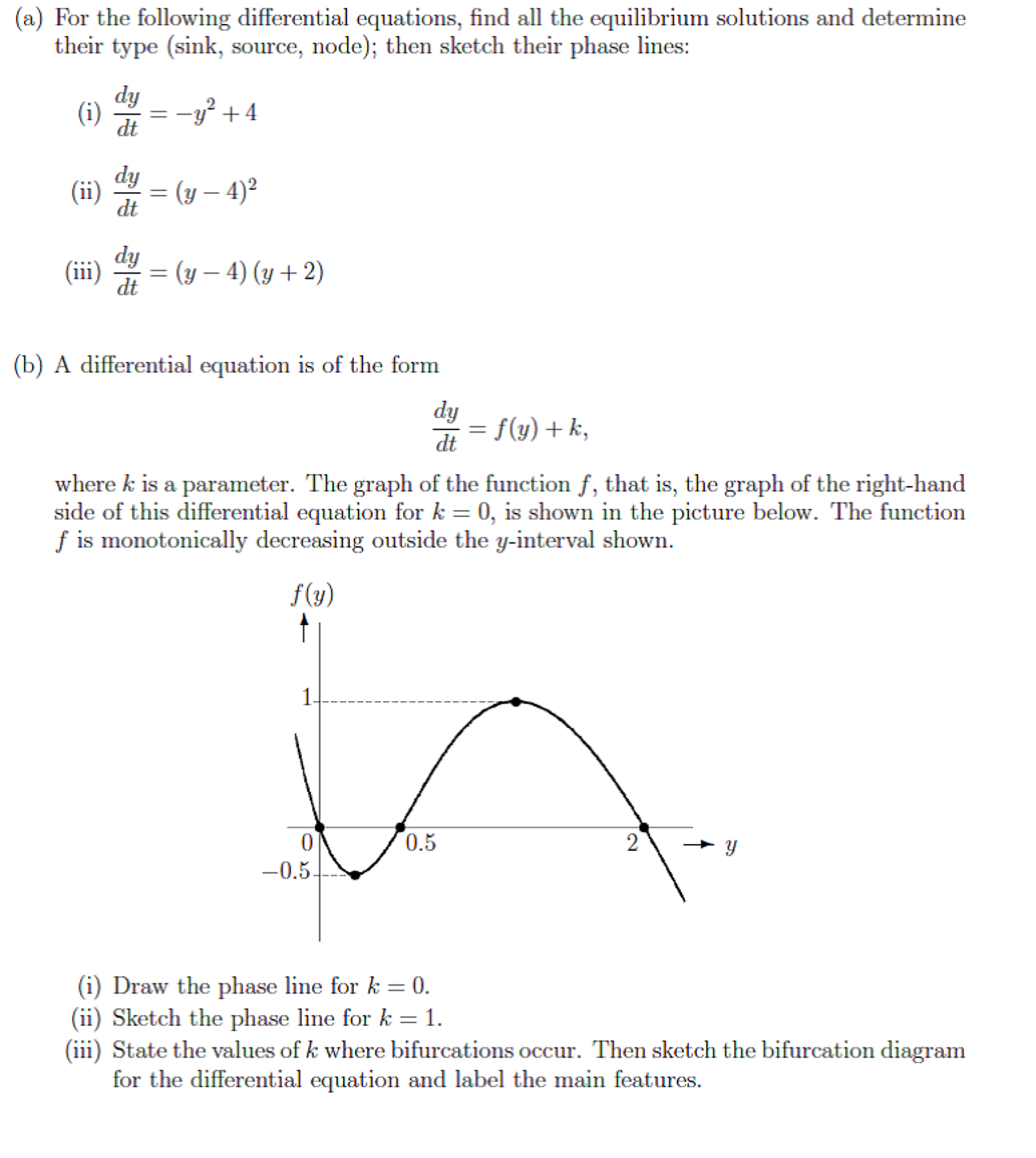
Solved (a) For the following differential equations, find
We know that a given differential equation is in the form y′ = f(y), where f is a differentiable function of y. Values of \(y\) for which \(f(y) = 0\) in an autonomous differential equation \(\frac{dy}{dt} = f(y)\) are called equilibrium. Suppose that f(6) = 0, f(14) = 0, and y(10) = 10. In this section we will define equilibrium.
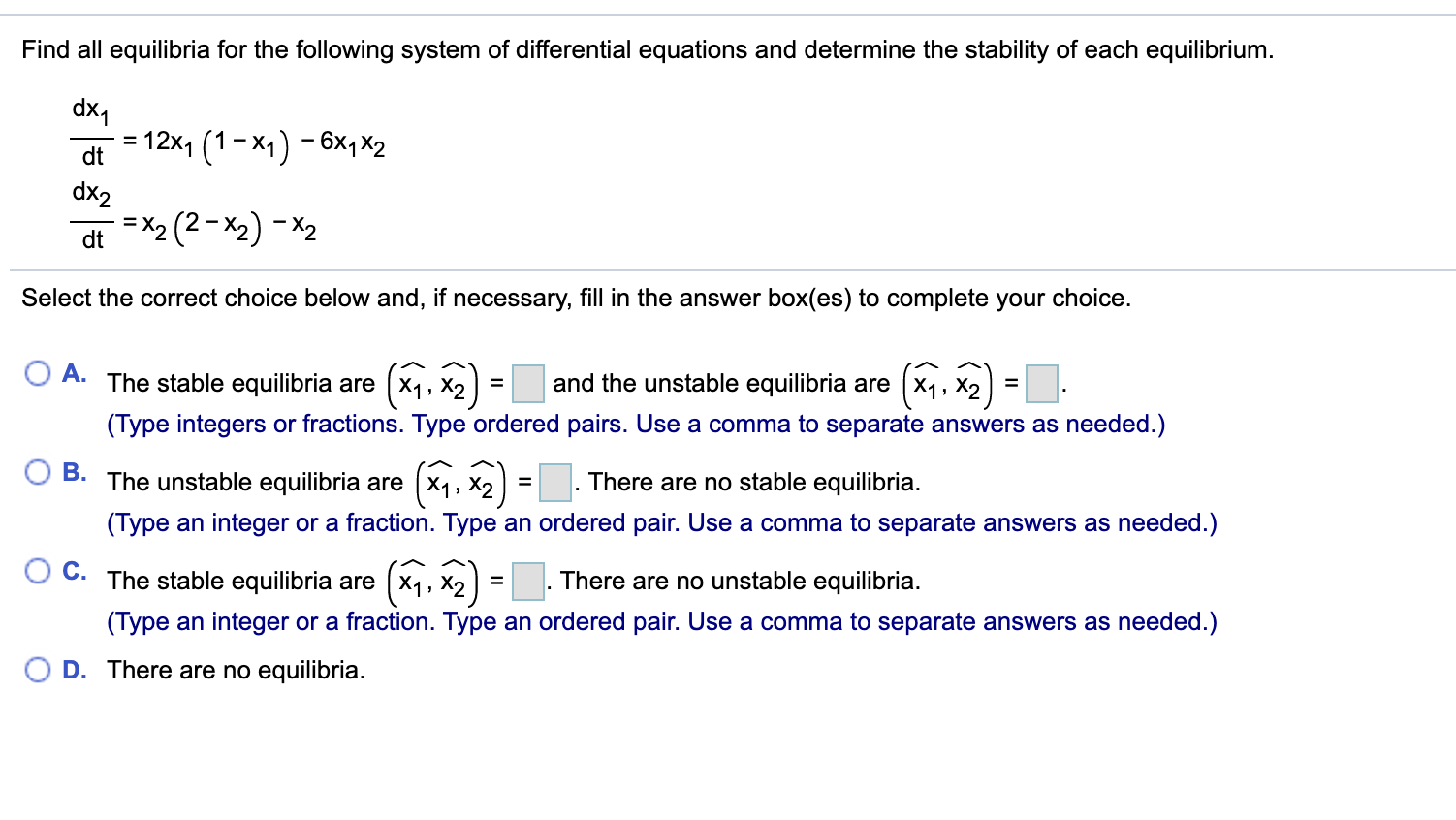
Solved Find all equilibria for the following system of
We know that a given differential equation is in the form y′ = f(y), where f is a differentiable function of y. Equilibrium solutions to differential equations. In studying systems of differential equations, it is often useful to study the behavior of solutions without obtaining an algebraic form. Suppose that we have a differential equation $\frac{dy}{dt} = f(t, y)$. Values.
Equilibrium solutions of differential equations Mathematics Stack
Suppose that f(6) = 0, f(14) = 0, and y(10) = 10. Suppose that we have a differential equation $\frac{dy}{dt} = f(t, y)$. In this section we will define equilibrium solutions (or equilibrium points) for autonomous differential equations, y’ = f(y). Sometimes it is easy to. Values of \(y\) for which \(f(y) = 0\) in an autonomous differential equation \(\frac{dy}{dt}.
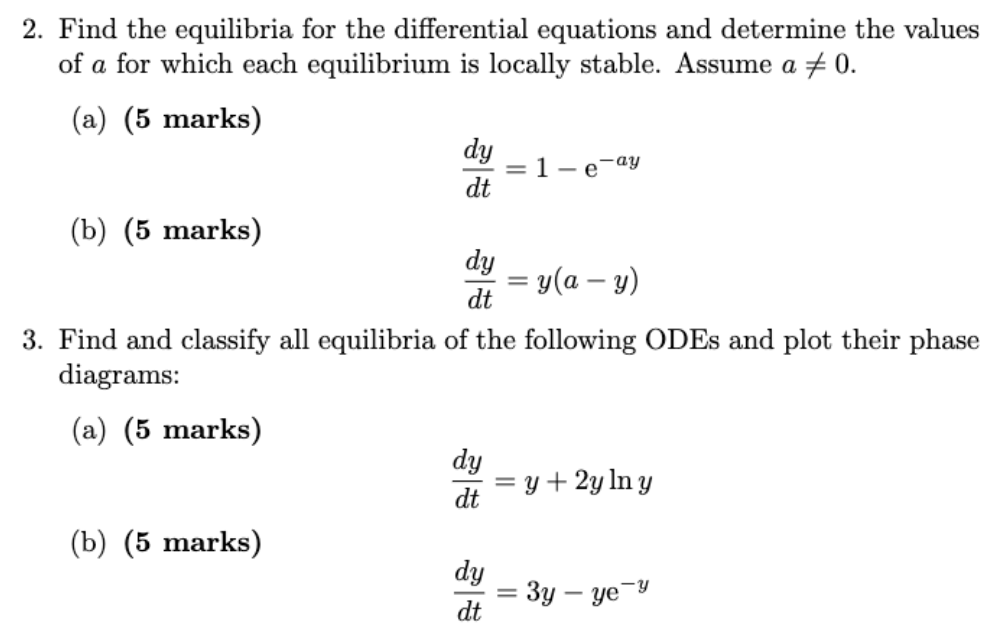
Solved 2. Find the equilibria for the differential equations
Sometimes it is easy to. In this section we will define equilibrium solutions (or equilibrium points) for autonomous differential equations, y’ = f(y). We know that a given differential equation is in the form y′ = f(y), where f is a differentiable function of y. Equilibrium solutions to differential equations. In studying systems of differential equations, it is often useful.
What are the differential equations? Types of Differential Equations
In studying systems of differential equations, it is often useful to study the behavior of solutions without obtaining an algebraic form. In this section we will define equilibrium solutions (or equilibrium points) for autonomous differential equations, y’ = f(y). Suppose that we have a differential equation $\frac{dy}{dt} = f(t, y)$. Suppose that f(6) = 0, f(14) = 0, and y(10).
Sometimes It Is Easy To.
In this section we will define equilibrium solutions (or equilibrium points) for autonomous differential equations, y’ = f(y). Values of \(y\) for which \(f(y) = 0\) in an autonomous differential equation \(\frac{dy}{dt} = f(y)\) are called equilibrium. Suppose that we have a differential equation $\frac{dy}{dt} = f(t, y)$. We know that a given differential equation is in the form y′ = f(y), where f is a differentiable function of y.
Suppose That F(6) = 0, F(14) = 0, And Y(10) = 10.
In studying systems of differential equations, it is often useful to study the behavior of solutions without obtaining an algebraic form. Equilibrium solutions to differential equations.