Differentiation Shortcuts

Differentiation Shortcuts - Basic derivative rules in this section, we are going to start developing some shortcuts for computing. 1) derivative of a constant: In this section, we are going to start developing some shortcuts for computing derivatives. [c]’ = 0 or dc/dx = 0 in leibniz form. We will do so by looking at some broad categories of. 2) derivative of a linear function: Differentiate each piece separately, according to the shortcuts above, to get a piecewise formula for the derivative. Using the definition of derivative in calculus is tedious, so differentiation shortcuts are developed. [mx + b]’ = m =.

Basic derivative rules in this section, we are going to start developing some shortcuts for computing. [mx + b]’ = m =. In this section, we are going to start developing some shortcuts for computing derivatives. 1) derivative of a constant: [c]’ = 0 or dc/dx = 0 in leibniz form. Using the definition of derivative in calculus is tedious, so differentiation shortcuts are developed. 2) derivative of a linear function: We will do so by looking at some broad categories of. Differentiate each piece separately, according to the shortcuts above, to get a piecewise formula for the derivative.

We will do so by looking at some broad categories of. Using the definition of derivative in calculus is tedious, so differentiation shortcuts are developed. [c]’ = 0 or dc/dx = 0 in leibniz form. In this section, we are going to start developing some shortcuts for computing derivatives. 1) derivative of a constant: [mx + b]’ = m =. Differentiate each piece separately, according to the shortcuts above, to get a piecewise formula for the derivative. 2) derivative of a linear function: Basic derivative rules in this section, we are going to start developing some shortcuts for computing.

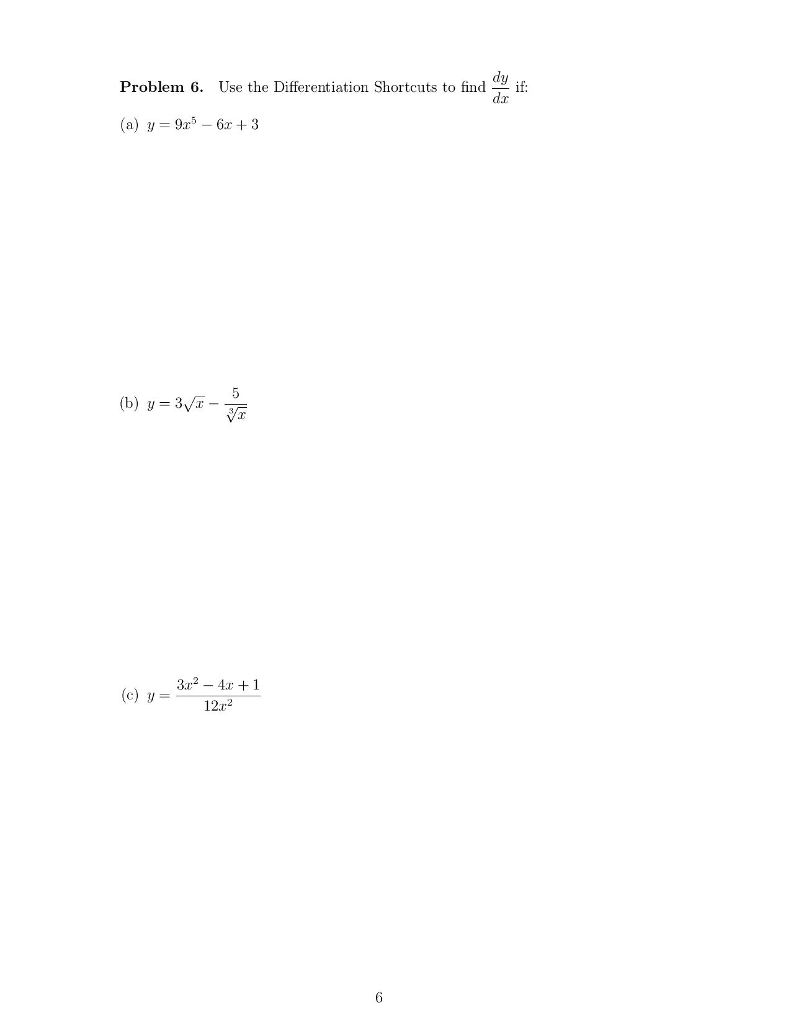
Solved Use the Differentiation Shortcuts to find dy/dx if

2) derivative of a linear function: Differentiate each piece separately, according to the shortcuts above, to get a piecewise formula for the derivative. [mx + b]’ = m =. In this section, we are going to start developing some shortcuts for computing derivatives. Basic derivative rules in this section, we are going to start developing some shortcuts for computing.

Differentiation Icon Style 21655633 Vector Art at Vecteezy

We will do so by looking at some broad categories of. In this section, we are going to start developing some shortcuts for computing derivatives. [mx + b]’ = m =. Using the definition of derivative in calculus is tedious, so differentiation shortcuts are developed. 2) derivative of a linear function:

How to Do Implicit Differentiation 7 Steps (with Pictures)

[c]’ = 0 or dc/dx = 0 in leibniz form. Differentiate each piece separately, according to the shortcuts above, to get a piecewise formula for the derivative. We will do so by looking at some broad categories of. In this section, we are going to start developing some shortcuts for computing derivatives. Basic derivative rules in this section, we are.

Differentiation Generic Flat icon

Basic derivative rules in this section, we are going to start developing some shortcuts for computing. [mx + b]’ = m =. Differentiate each piece separately, according to the shortcuts above, to get a piecewise formula for the derivative. 2) derivative of a linear function: Using the definition of derivative in calculus is tedious, so differentiation shortcuts are developed.

Differentiation Generic Flat icon

[c]’ = 0 or dc/dx = 0 in leibniz form. [mx + b]’ = m =. We will do so by looking at some broad categories of. In this section, we are going to start developing some shortcuts for computing derivatives. Using the definition of derivative in calculus is tedious, so differentiation shortcuts are developed.

$Solved \4. [15 pts] Use differentiation shortcuts to find a$

Solved \4. [15 pts] Use differentiation shortcuts to find a

We will do so by looking at some broad categories of. 2) derivative of a linear function: [mx + b]’ = m =. [c]’ = 0 or dc/dx = 0 in leibniz form. Basic derivative rules in this section, we are going to start developing some shortcuts for computing.

Differentiation An Important Marketing Strategy Technique Career Parts

2) derivative of a linear function: 1) derivative of a constant: Basic derivative rules in this section, we are going to start developing some shortcuts for computing. [c]’ = 0 or dc/dx = 0 in leibniz form. Differentiate each piece separately, according to the shortcuts above, to get a piecewise formula for the derivative.

Differentiation Free seo and web icons

2) derivative of a linear function: [c]’ = 0 or dc/dx = 0 in leibniz form. 1) derivative of a constant: Differentiate each piece separately, according to the shortcuts above, to get a piecewise formula for the derivative. We will do so by looking at some broad categories of.

Differentiation Button Cartoon Vector 196836881

In this section, we are going to start developing some shortcuts for computing derivatives. 1) derivative of a constant: Basic derivative rules in this section, we are going to start developing some shortcuts for computing. Differentiate each piece separately, according to the shortcuts above, to get a piecewise formula for the derivative. 2) derivative of a linear function:

Solved Chapter 3 Shortcuts to Differentiation 3.1 Derivative

[c]’ = 0 or dc/dx = 0 in leibniz form. In this section, we are going to start developing some shortcuts for computing derivatives. 2) derivative of a linear function: [mx + b]’ = m =. Differentiate each piece separately, according to the shortcuts above, to get a piecewise formula for the derivative.

Differentiate Each Piece Separately, According To The Shortcuts Above, To Get A Piecewise Formula For The Derivative.

Basic derivative rules in this section, we are going to start developing some shortcuts for computing. Using the definition of derivative in calculus is tedious, so differentiation shortcuts are developed. [mx + b]’ = m =. In this section, we are going to start developing some shortcuts for computing derivatives.