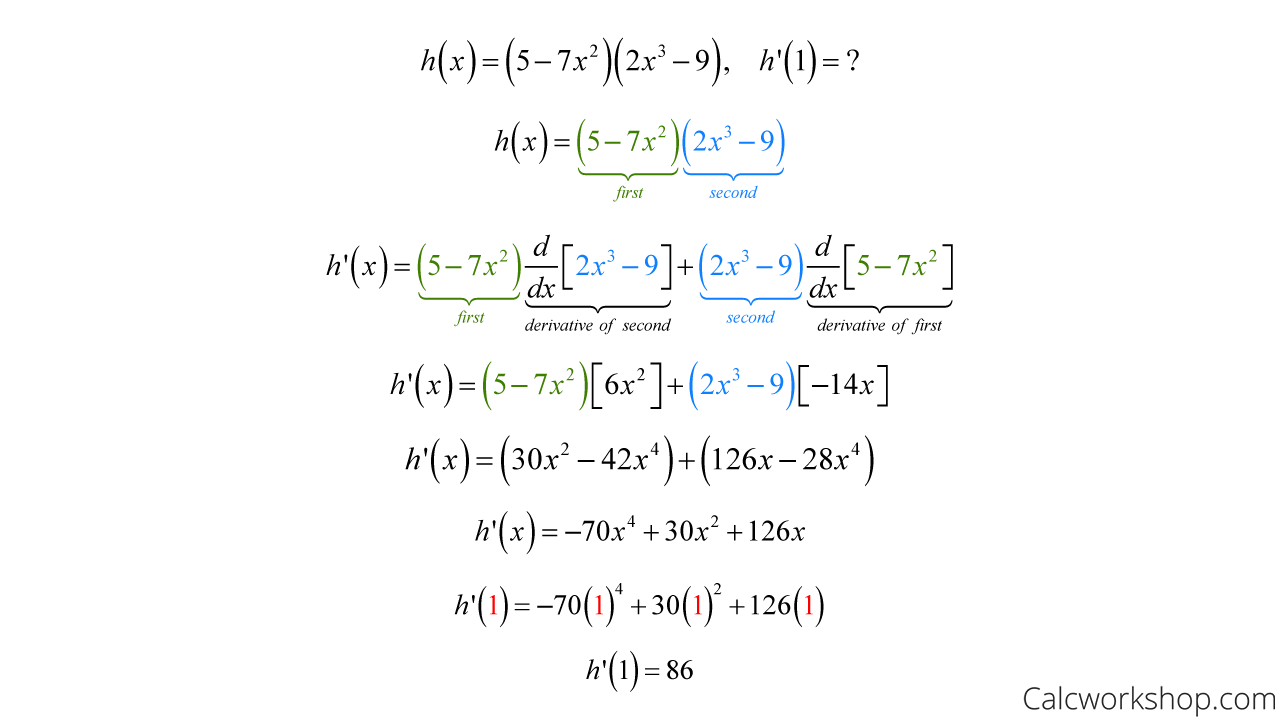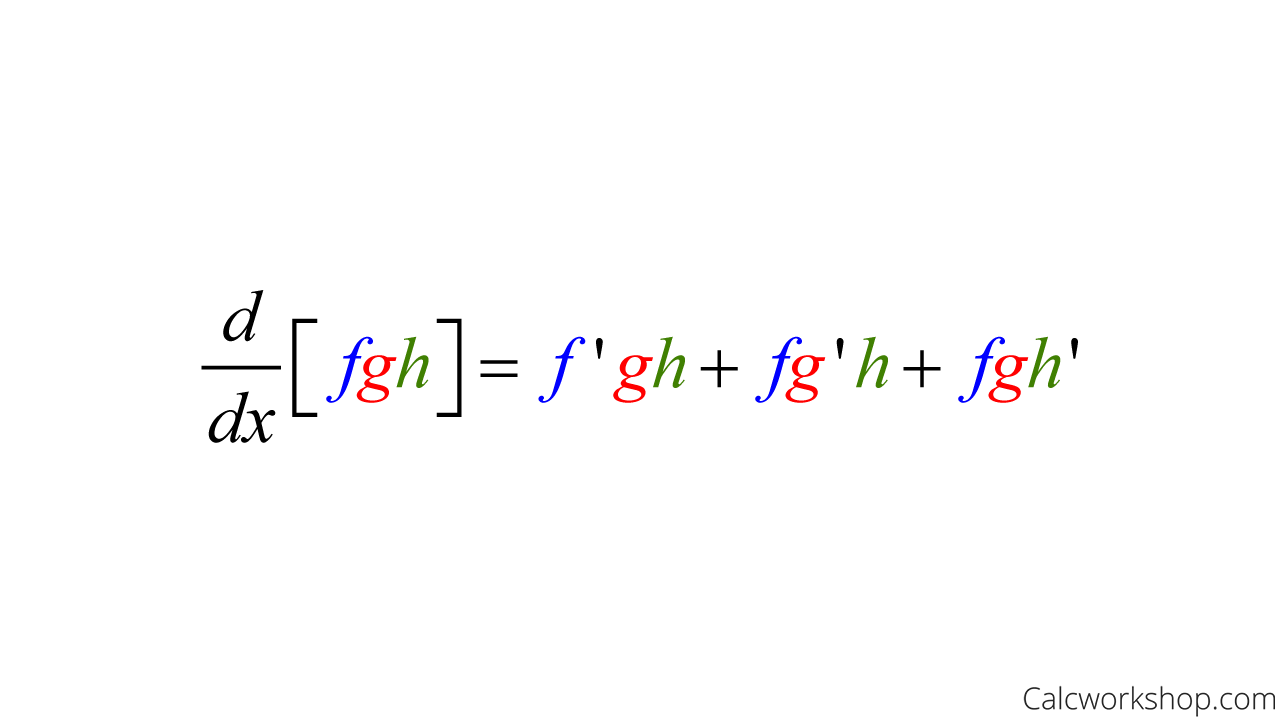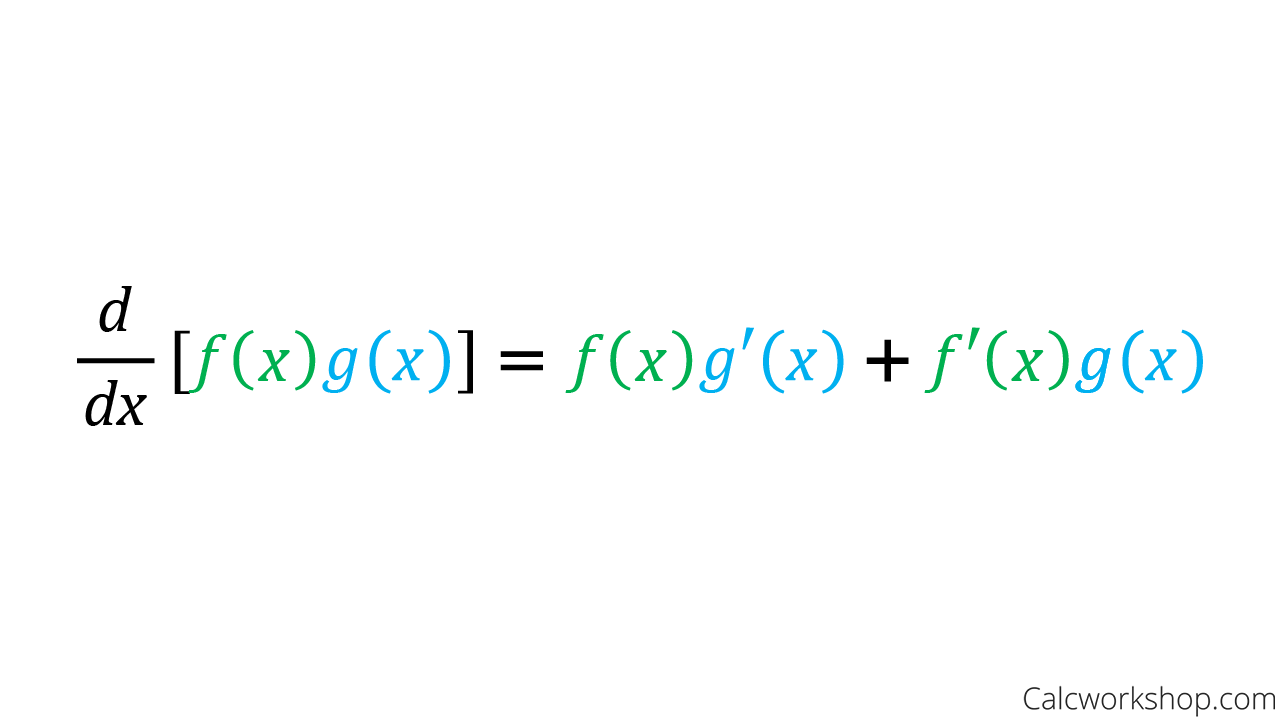Differentiation Product Rule Proof - Using leibniz's notation for derivatives, this can be written as: How i do i prove the product rule for derivatives? The product rule follows the concept of limits and derivatives in differentiation directly. If the two functions \ (f\left ( x \right)\) and \ (g\left ( x \right)\) are differentiable (i.e. Let us understand the product rule formula, its proof. \({\left( {f\,g} \right)^\prime } = f'\,g + f\,g'\) as with the power rule above, the product rule can be proved. In calculus, the product rule (or leibniz rule[1] or leibniz product rule) is a formula used to find the derivatives of products of two or more. $\map {\dfrac \d {\d x} } {y \, z} = y \dfrac {\d z} {\d x} + \dfrac. The derivative exist) then the product is. All we need to do is use the definition of the derivative alongside a simple algebraic trick.
All we need to do is use the definition of the derivative alongside a simple algebraic trick. The product rule follows the concept of limits and derivatives in differentiation directly. The derivative exist) then the product is. How i do i prove the product rule for derivatives? Let us understand the product rule formula, its proof. \({\left( {f\,g} \right)^\prime } = f'\,g + f\,g'\) as with the power rule above, the product rule can be proved. $\map {\dfrac \d {\d x} } {y \, z} = y \dfrac {\d z} {\d x} + \dfrac. Using leibniz's notation for derivatives, this can be written as: If the two functions \ (f\left ( x \right)\) and \ (g\left ( x \right)\) are differentiable (i.e. In calculus, the product rule (or leibniz rule[1] or leibniz product rule) is a formula used to find the derivatives of products of two or more.
Using leibniz's notation for derivatives, this can be written as: All we need to do is use the definition of the derivative alongside a simple algebraic trick. Let us understand the product rule formula, its proof. In calculus, the product rule (or leibniz rule[1] or leibniz product rule) is a formula used to find the derivatives of products of two or more. If the two functions \ (f\left ( x \right)\) and \ (g\left ( x \right)\) are differentiable (i.e. $\map {\dfrac \d {\d x} } {y \, z} = y \dfrac {\d z} {\d x} + \dfrac. The product rule follows the concept of limits and derivatives in differentiation directly. How i do i prove the product rule for derivatives? The derivative exist) then the product is. \({\left( {f\,g} \right)^\prime } = f'\,g + f\,g'\) as with the power rule above, the product rule can be proved.
Differentiation, Product rule Teaching Resources
Using leibniz's notation for derivatives, this can be written as: \({\left( {f\,g} \right)^\prime } = f'\,g + f\,g'\) as with the power rule above, the product rule can be proved. The derivative exist) then the product is. In calculus, the product rule (or leibniz rule[1] or leibniz product rule) is a formula used to find the derivatives of products of.
Product Rule of Differentiation
The product rule follows the concept of limits and derivatives in differentiation directly. Using leibniz's notation for derivatives, this can be written as: \({\left( {f\,g} \right)^\prime } = f'\,g + f\,g'\) as with the power rule above, the product rule can be proved. Let us understand the product rule formula, its proof. How i do i prove the product rule.
Mathematics 数学分享站 【Differentiation】Product Rule PROOF
All we need to do is use the definition of the derivative alongside a simple algebraic trick. The derivative exist) then the product is. In calculus, the product rule (or leibniz rule[1] or leibniz product rule) is a formula used to find the derivatives of products of two or more. The product rule follows the concept of limits and derivatives.
Product Rule For Calculus (w/ StepbyStep Examples!)
How i do i prove the product rule for derivatives? Using leibniz's notation for derivatives, this can be written as: $\map {\dfrac \d {\d x} } {y \, z} = y \dfrac {\d z} {\d x} + \dfrac. The product rule follows the concept of limits and derivatives in differentiation directly. If the two functions \ (f\left ( x \right)\).
Product Rule For Calculus (w/ StepbyStep Examples!)
In calculus, the product rule (or leibniz rule[1] or leibniz product rule) is a formula used to find the derivatives of products of two or more. Let us understand the product rule formula, its proof. The product rule follows the concept of limits and derivatives in differentiation directly. If the two functions \ (f\left ( x \right)\) and \ (g\left.
Proof of Product Rule of Differentiation
All we need to do is use the definition of the derivative alongside a simple algebraic trick. $\map {\dfrac \d {\d x} } {y \, z} = y \dfrac {\d z} {\d x} + \dfrac. The product rule follows the concept of limits and derivatives in differentiation directly. How i do i prove the product rule for derivatives? The derivative.
Product Rule For Calculus (w/ StepbyStep Examples!)
The derivative exist) then the product is. $\map {\dfrac \d {\d x} } {y \, z} = y \dfrac {\d z} {\d x} + \dfrac. Using leibniz's notation for derivatives, this can be written as: Let us understand the product rule formula, its proof. How i do i prove the product rule for derivatives?
Proof Differentiation PDF
The derivative exist) then the product is. \({\left( {f\,g} \right)^\prime } = f'\,g + f\,g'\) as with the power rule above, the product rule can be proved. The product rule follows the concept of limits and derivatives in differentiation directly. All we need to do is use the definition of the derivative alongside a simple algebraic trick. $\map {\dfrac \d.
calculus Product rule, help me understand this proof Mathematics
Using leibniz's notation for derivatives, this can be written as: Let us understand the product rule formula, its proof. \({\left( {f\,g} \right)^\prime } = f'\,g + f\,g'\) as with the power rule above, the product rule can be proved. The derivative exist) then the product is. How i do i prove the product rule for derivatives?
Mathematics 数学分享站 【Differentiation】Product Rule PROOF
If the two functions \ (f\left ( x \right)\) and \ (g\left ( x \right)\) are differentiable (i.e. All we need to do is use the definition of the derivative alongside a simple algebraic trick. Let us understand the product rule formula, its proof. $\map {\dfrac \d {\d x} } {y \, z} = y \dfrac {\d z} {\d x}.
\({\Left( {F\,G} \Right)^\Prime } = F'\,G + F\,G'\) As With The Power Rule Above, The Product Rule Can Be Proved.
All we need to do is use the definition of the derivative alongside a simple algebraic trick. The product rule follows the concept of limits and derivatives in differentiation directly. The derivative exist) then the product is. If the two functions \ (f\left ( x \right)\) and \ (g\left ( x \right)\) are differentiable (i.e.
How I Do I Prove The Product Rule For Derivatives?
Let us understand the product rule formula, its proof. $\map {\dfrac \d {\d x} } {y \, z} = y \dfrac {\d z} {\d x} + \dfrac. In calculus, the product rule (or leibniz rule[1] or leibniz product rule) is a formula used to find the derivatives of products of two or more. Using leibniz's notation for derivatives, this can be written as: