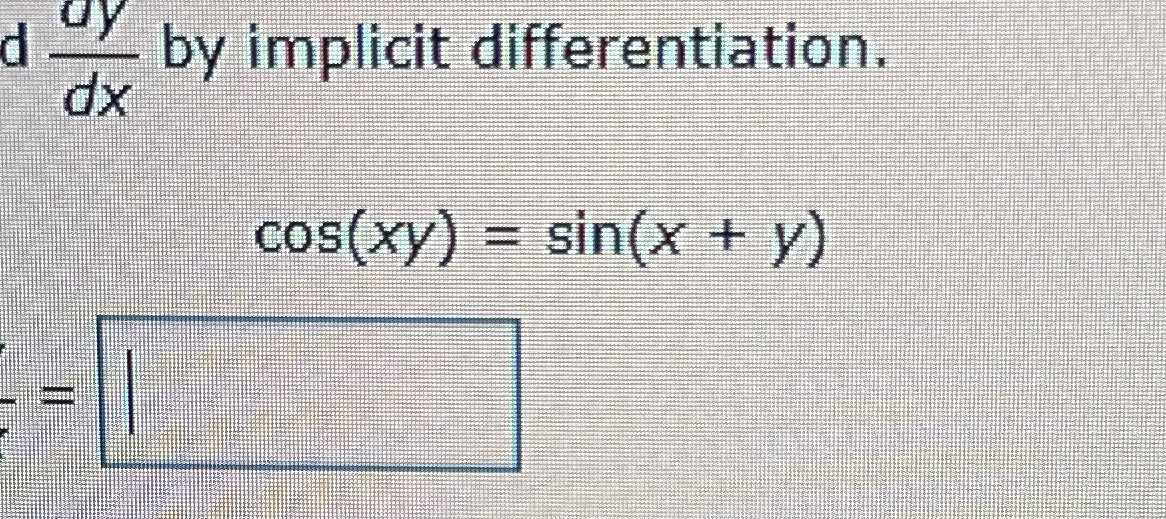Differentiation Of Cos Xy - Free math problem solver answers your algebra, geometry, trigonometry, calculus, and statistics. The differentiation of trigonometric functions is the mathematical process of finding the derivative of a trigonometric function, or its rate of change. Differentiate using the chain rule, which states that d dx [f (g(x))] d d x [f (g (x))] is f '(g(x))g'(x) f ′ (g (x)) g ′ (x) where f (x) = cos(x) f (x) = cos (x). D dx cos(xy) = −(y + x dy dx)sin(xy) use the chain rule: \int e^x\cos (x)dx \int_{0}^{\pi}\sin(x)dx \sum_{n=0}^{\infty}\frac{3}{2^n} show more D dx cos(xy) = −sin(xy) ⋅ d dx (xy) then the. What is the derivative of cos(xy)? Replace y' y ′ with dy dx d y d x.
Differentiate using the chain rule, which states that d dx [f (g(x))] d d x [f (g (x))] is f '(g(x))g'(x) f ′ (g (x)) g ′ (x) where f (x) = cos(x) f (x) = cos (x). D dx cos(xy) = −sin(xy) ⋅ d dx (xy) then the. \int e^x\cos (x)dx \int_{0}^{\pi}\sin(x)dx \sum_{n=0}^{\infty}\frac{3}{2^n} show more Replace y' y ′ with dy dx d y d x. Free math problem solver answers your algebra, geometry, trigonometry, calculus, and statistics. The differentiation of trigonometric functions is the mathematical process of finding the derivative of a trigonometric function, or its rate of change. D dx cos(xy) = −(y + x dy dx)sin(xy) use the chain rule: What is the derivative of cos(xy)?
Replace y' y ′ with dy dx d y d x. D dx cos(xy) = −sin(xy) ⋅ d dx (xy) then the. D dx cos(xy) = −(y + x dy dx)sin(xy) use the chain rule: What is the derivative of cos(xy)? Differentiate using the chain rule, which states that d dx [f (g(x))] d d x [f (g (x))] is f '(g(x))g'(x) f ′ (g (x)) g ′ (x) where f (x) = cos(x) f (x) = cos (x). The differentiation of trigonometric functions is the mathematical process of finding the derivative of a trigonometric function, or its rate of change. \int e^x\cos (x)dx \int_{0}^{\pi}\sin(x)dx \sum_{n=0}^{\infty}\frac{3}{2^n} show more Free math problem solver answers your algebra, geometry, trigonometry, calculus, and statistics.
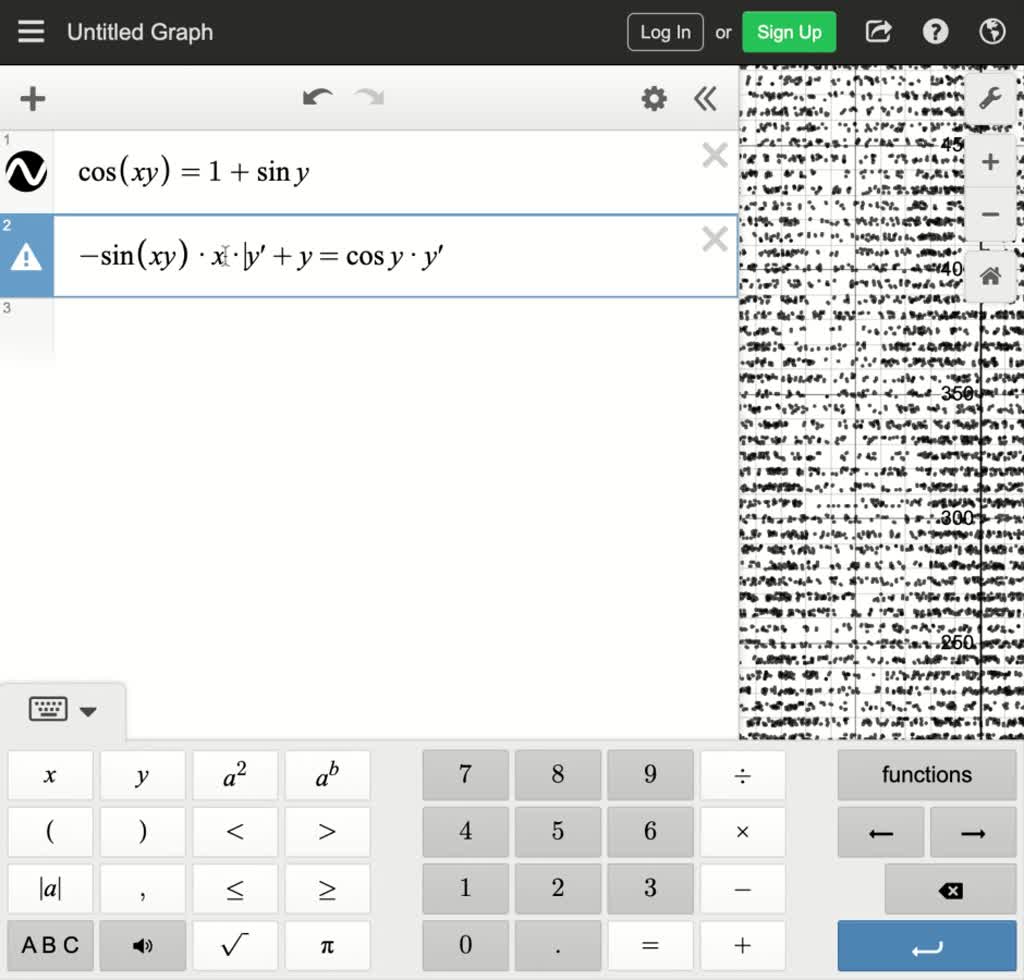
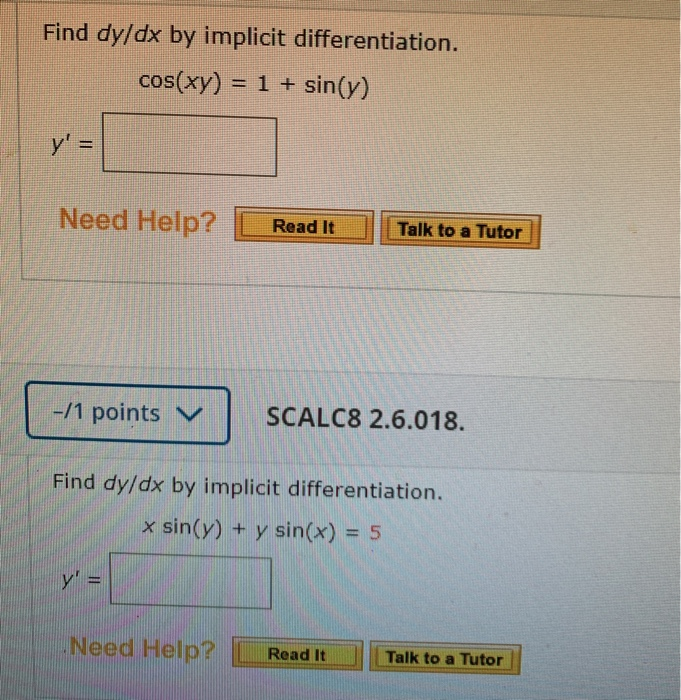
SOLVED Find dy/dx by implicit differentiation. cos(xy) = 1 + siny
What is the derivative of cos(xy)? Replace y' y ′ with dy dx d y d x. D dx cos(xy) = −sin(xy) ⋅ d dx (xy) then the. \int e^x\cos (x)dx \int_{0}^{\pi}\sin(x)dx \sum_{n=0}^{\infty}\frac{3}{2^n} show more Free math problem solver answers your algebra, geometry, trigonometry, calculus, and statistics.
Solved Find dy/dx by implicit differentiation. cos (xy)=sin (x+y) dy
D dx cos(xy) = −sin(xy) ⋅ d dx (xy) then the. Free math problem solver answers your algebra, geometry, trigonometry, calculus, and statistics. Replace y' y ′ with dy dx d y d x. The differentiation of trigonometric functions is the mathematical process of finding the derivative of a trigonometric function, or its rate of change. Differentiate using the chain.
Solved Given the following differential equation ( cos(xy?)
D dx cos(xy) = −sin(xy) ⋅ d dx (xy) then the. \int e^x\cos (x)dx \int_{0}^{\pi}\sin(x)dx \sum_{n=0}^{\infty}\frac{3}{2^n} show more The differentiation of trigonometric functions is the mathematical process of finding the derivative of a trigonometric function, or its rate of change. Free math problem solver answers your algebra, geometry, trigonometry, calculus, and statistics. Differentiate using the chain rule, which states that.
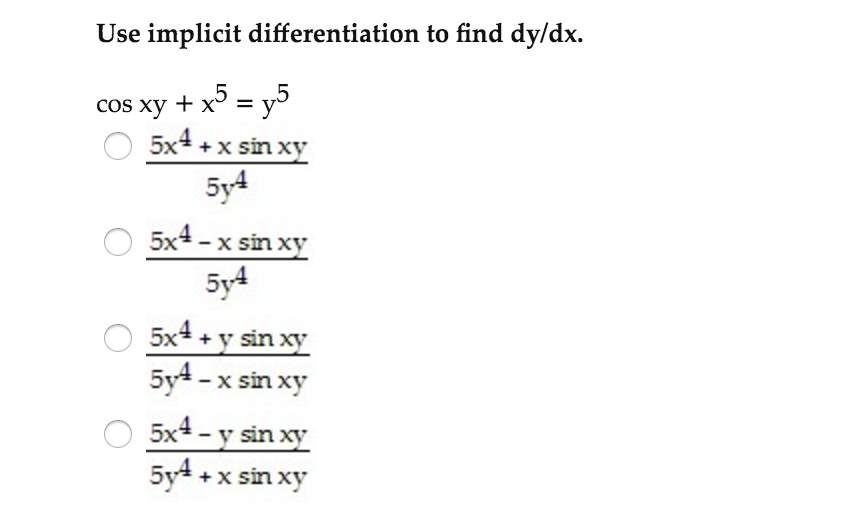
Solved Use implicit differentiation to find dy/dx. Cos xy +
D dx cos(xy) = −sin(xy) ⋅ d dx (xy) then the. \int e^x\cos (x)dx \int_{0}^{\pi}\sin(x)dx \sum_{n=0}^{\infty}\frac{3}{2^n} show more What is the derivative of cos(xy)? The differentiation of trigonometric functions is the mathematical process of finding the derivative of a trigonometric function, or its rate of change. Free math problem solver answers your algebra, geometry, trigonometry, calculus, and statistics.
[Solved] Find dy / dx by implicit differentiation. cos( xy ) = 1 + sin
Differentiate using the chain rule, which states that d dx [f (g(x))] d d x [f (g (x))] is f '(g(x))g'(x) f ′ (g (x)) g ′ (x) where f (x) = cos(x) f (x) = cos (x). Replace y' y ′ with dy dx d y d x. D dx cos(xy) = −(y + x dy dx)sin(xy) use the.
Solved ddydx by implicit differentiation.cos(xy)=sin(x+y)
D dx cos(xy) = −(y + x dy dx)sin(xy) use the chain rule: Differentiate using the chain rule, which states that d dx [f (g(x))] d d x [f (g (x))] is f '(g(x))g'(x) f ′ (g (x)) g ′ (x) where f (x) = cos(x) f (x) = cos (x). \int e^x\cos (x)dx \int_{0}^{\pi}\sin(x)dx \sum_{n=0}^{\infty}\frac{3}{2^n} show more The differentiation.
Solved Find dy/dx by implicit differentiation. cos(xy) = 1 +
The differentiation of trigonometric functions is the mathematical process of finding the derivative of a trigonometric function, or its rate of change. D dx cos(xy) = −sin(xy) ⋅ d dx (xy) then the. Replace y' y ′ with dy dx d y d x. What is the derivative of cos(xy)? Differentiate using the chain rule, which states that d dx.
Solved Given the following differential equation (cos(xy) +
Replace y' y ′ with dy dx d y d x. What is the derivative of cos(xy)? The differentiation of trigonometric functions is the mathematical process of finding the derivative of a trigonometric function, or its rate of change. \int e^x\cos (x)dx \int_{0}^{\pi}\sin(x)dx \sum_{n=0}^{\infty}\frac{3}{2^n} show more Free math problem solver answers your algebra, geometry, trigonometry, calculus, and statistics.
[Solved] Find dy / dx by implicit differentiation. cos( xy ) = 1 + sin
Differentiate using the chain rule, which states that d dx [f (g(x))] d d x [f (g (x))] is f '(g(x))g'(x) f ′ (g (x)) g ′ (x) where f (x) = cos(x) f (x) = cos (x). Replace y' y ′ with dy dx d y d x. \int e^x\cos (x)dx \int_{0}^{\pi}\sin(x)dx \sum_{n=0}^{\infty}\frac{3}{2^n} show more What is the derivative.
Solved If y=x+sin(xy), then
The differentiation of trigonometric functions is the mathematical process of finding the derivative of a trigonometric function, or its rate of change. D dx cos(xy) = −sin(xy) ⋅ d dx (xy) then the. \int e^x\cos (x)dx \int_{0}^{\pi}\sin(x)dx \sum_{n=0}^{\infty}\frac{3}{2^n} show more Replace y' y ′ with dy dx d y d x. Differentiate using the chain rule, which states that d.
Free Math Problem Solver Answers Your Algebra, Geometry, Trigonometry, Calculus, And Statistics.
D dx cos(xy) = −sin(xy) ⋅ d dx (xy) then the. What is the derivative of cos(xy)? \int e^x\cos (x)dx \int_{0}^{\pi}\sin(x)dx \sum_{n=0}^{\infty}\frac{3}{2^n} show more Differentiate using the chain rule, which states that d dx [f (g(x))] d d x [f (g (x))] is f '(g(x))g'(x) f ′ (g (x)) g ′ (x) where f (x) = cos(x) f (x) = cos (x).
D Dx Cos(Xy) = −(Y + X Dy Dx)Sin(Xy) Use The Chain Rule:
The differentiation of trigonometric functions is the mathematical process of finding the derivative of a trigonometric function, or its rate of change. Replace y' y ′ with dy dx d y d x.