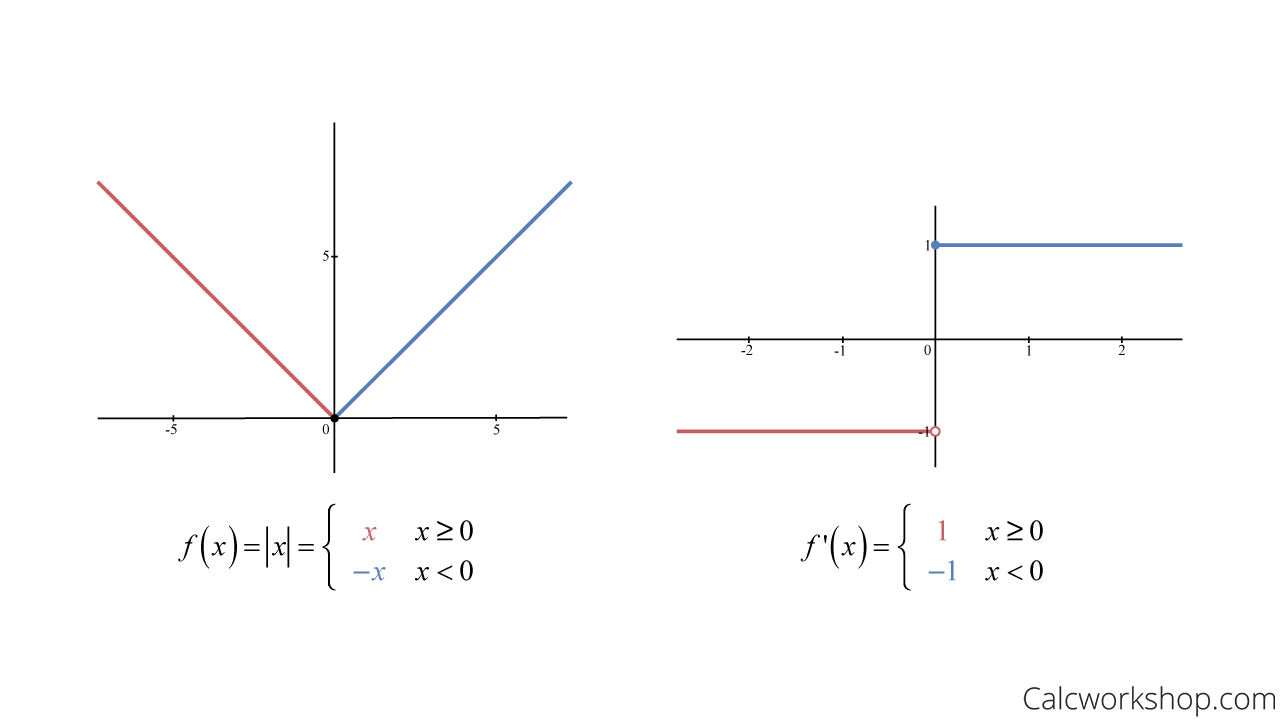
Differentiation Of Absolute Function - $\dfrac \d {\d x} \size x = \dfrac x {\size x}$ for $x \ne 0$. Let $\size x$ be the absolute value of $x$ for real $x$. What is the derivative of an absolute value? Y' = 2(x −2) 2√(x − 2)2 → power rule. The absolute value function | x | is defined. D dx |u| = u |u| ⋅ du dx. Since an absolute value function is represented by the graph of two “linear” equations coming together to form a “v” the derivative is a. The derivative of absolute value (function) is defined as the rate of change or the slope of a function at a specific point. To understand the derivative of the absolute value function | x |, let’s break it down step by step.
Since an absolute value function is represented by the graph of two “linear” equations coming together to form a “v” the derivative is a. Y' = 2(x −2) 2√(x − 2)2 → power rule. $\dfrac \d {\d x} \size x = \dfrac x {\size x}$ for $x \ne 0$. D dx |u| = u |u| ⋅ du dx. The derivative of absolute value (function) is defined as the rate of change or the slope of a function at a specific point. Let $\size x$ be the absolute value of $x$ for real $x$. The absolute value function | x | is defined. What is the derivative of an absolute value? To understand the derivative of the absolute value function | x |, let’s break it down step by step.
The absolute value function | x | is defined. What is the derivative of an absolute value? Since an absolute value function is represented by the graph of two “linear” equations coming together to form a “v” the derivative is a. The derivative of absolute value (function) is defined as the rate of change or the slope of a function at a specific point. To understand the derivative of the absolute value function | x |, let’s break it down step by step. D dx |u| = u |u| ⋅ du dx. Let $\size x$ be the absolute value of $x$ for real $x$. Y' = 2(x −2) 2√(x − 2)2 → power rule. $\dfrac \d {\d x} \size x = \dfrac x {\size x}$ for $x \ne 0$.
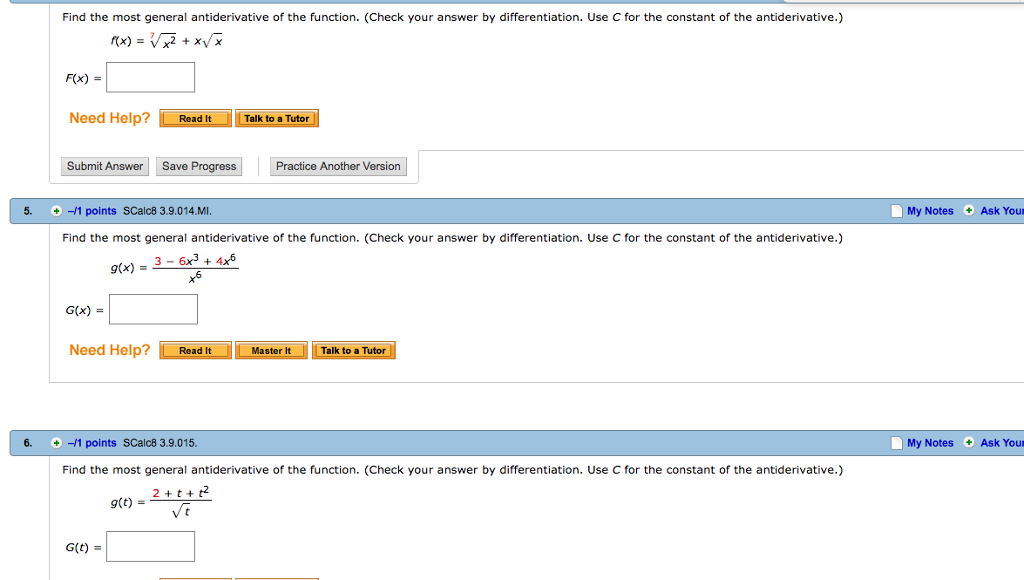
Solved Find the most general antiderivative of the function
The absolute value function | x | is defined. What is the derivative of an absolute value? Since an absolute value function is represented by the graph of two “linear” equations coming together to form a “v” the derivative is a. Y' = 2(x −2) 2√(x − 2)2 → power rule. To understand the derivative of the absolute value function.
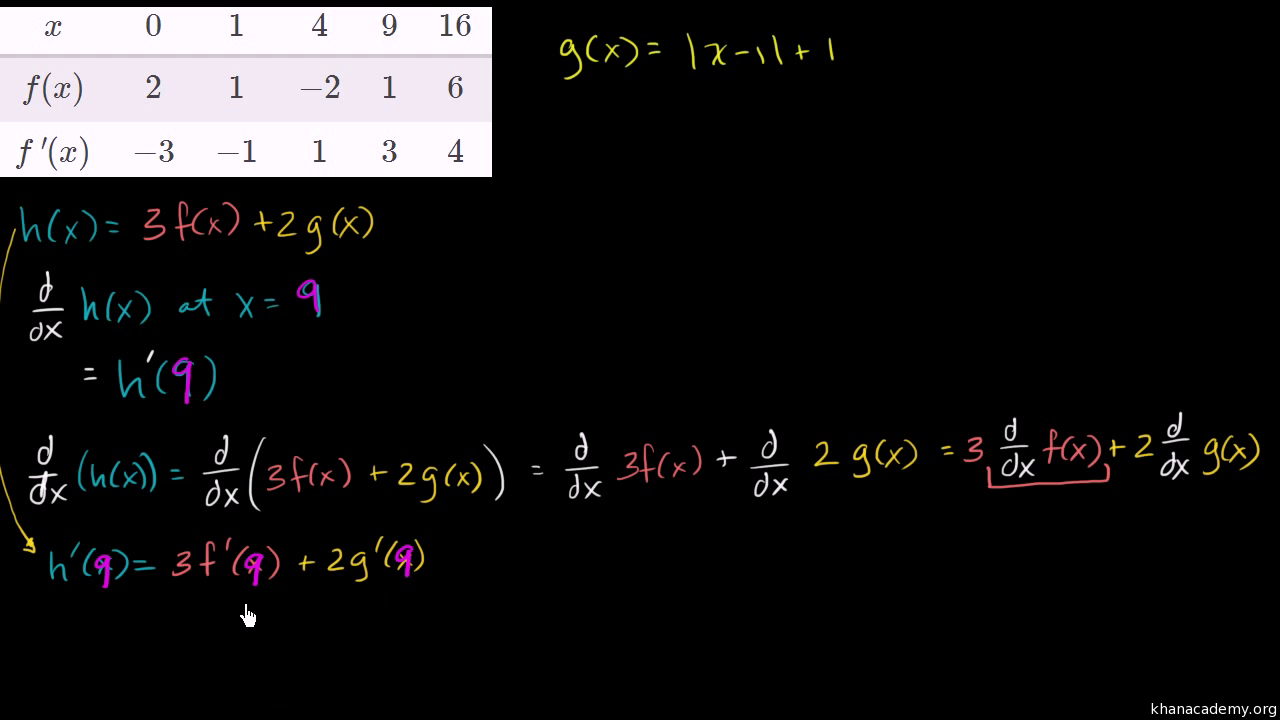
Absolute Value Function Khan Academy at Lemuel McLaughlin blog
The derivative of absolute value (function) is defined as the rate of change or the slope of a function at a specific point. $\dfrac \d {\d x} \size x = \dfrac x {\size x}$ for $x \ne 0$. The absolute value function | x | is defined. Since an absolute value function is represented by the graph of two “linear”.
Differentiate Absolute Value Function
To understand the derivative of the absolute value function | x |, let’s break it down step by step. Let $\size x$ be the absolute value of $x$ for real $x$. Y' = 2(x −2) 2√(x − 2)2 → power rule. The derivative of absolute value (function) is defined as the rate of change or the slope of a function.
Differentiate Absolute Value Function
The absolute value function | x | is defined. The derivative of absolute value (function) is defined as the rate of change or the slope of a function at a specific point. $\dfrac \d {\d x} \size x = \dfrac x {\size x}$ for $x \ne 0$. D dx |u| = u |u| ⋅ du dx. What is the derivative.
Finding the Derivative of Absolute Value Overview & Examples Video
The derivative of absolute value (function) is defined as the rate of change or the slope of a function at a specific point. Since an absolute value function is represented by the graph of two “linear” equations coming together to form a “v” the derivative is a. What is the derivative of an absolute value? To understand the derivative of.
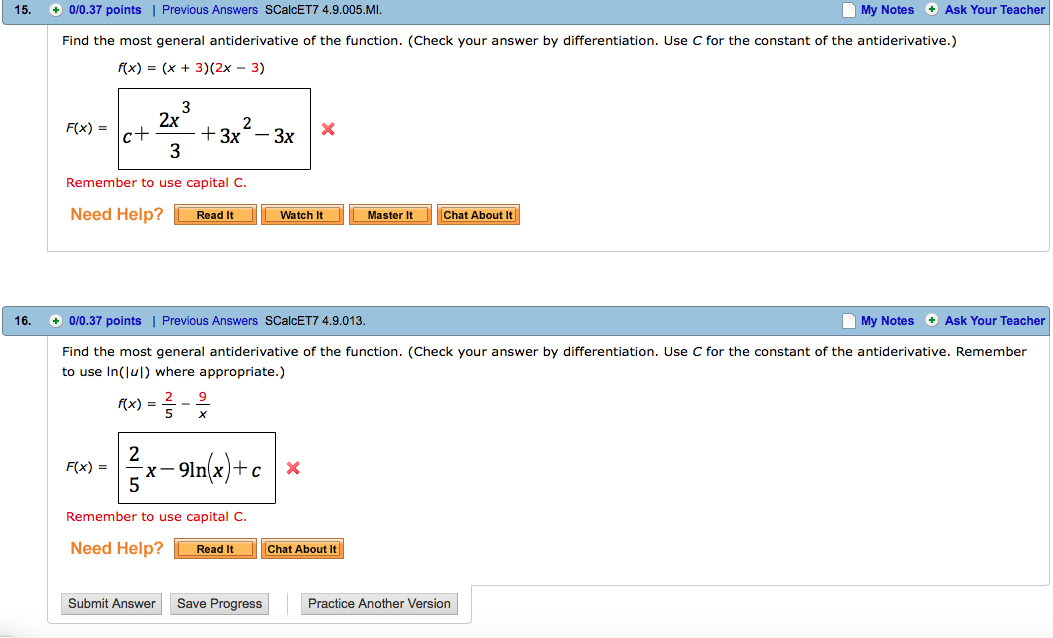
Solved Find the most general antiderivative of the function.
The derivative of absolute value (function) is defined as the rate of change or the slope of a function at a specific point. Let $\size x$ be the absolute value of $x$ for real $x$. $\dfrac \d {\d x} \size x = \dfrac x {\size x}$ for $x \ne 0$. The absolute value function | x | is defined. Y'.
1 Absolute Function and Graph It PDF
The derivative of absolute value (function) is defined as the rate of change or the slope of a function at a specific point. Y' = 2(x −2) 2√(x − 2)2 → power rule. Since an absolute value function is represented by the graph of two “linear” equations coming together to form a “v” the derivative is a. D dx |u|.
Differentiate Absolute Value Function
What is the derivative of an absolute value? The absolute value function | x | is defined. To understand the derivative of the absolute value function | x |, let’s break it down step by step. Since an absolute value function is represented by the graph of two “linear” equations coming together to form a “v” the derivative is a..
Solved Find the most general antiderivative of the function.
Let $\size x$ be the absolute value of $x$ for real $x$. To understand the derivative of the absolute value function | x |, let’s break it down step by step. Since an absolute value function is represented by the graph of two “linear” equations coming together to form a “v” the derivative is a. Y' = 2(x −2) 2√(x.
Download Example Of Absolute Function ClipartKey
To understand the derivative of the absolute value function | x |, let’s break it down step by step. Since an absolute value function is represented by the graph of two “linear” equations coming together to form a “v” the derivative is a. The absolute value function | x | is defined. Let $\size x$ be the absolute value of.
$\Dfrac \D {\D X} \Size X = \Dfrac X {\Size X}$ For $X \Ne 0$.
The absolute value function | x | is defined. What is the derivative of an absolute value? Let $\size x$ be the absolute value of $x$ for real $x$. Since an absolute value function is represented by the graph of two “linear” equations coming together to form a “v” the derivative is a.
Y' = 2(X −2) 2√(X − 2)2 → Power Rule.
The derivative of absolute value (function) is defined as the rate of change or the slope of a function at a specific point. D dx |u| = u |u| ⋅ du dx. To understand the derivative of the absolute value function | x |, let’s break it down step by step.