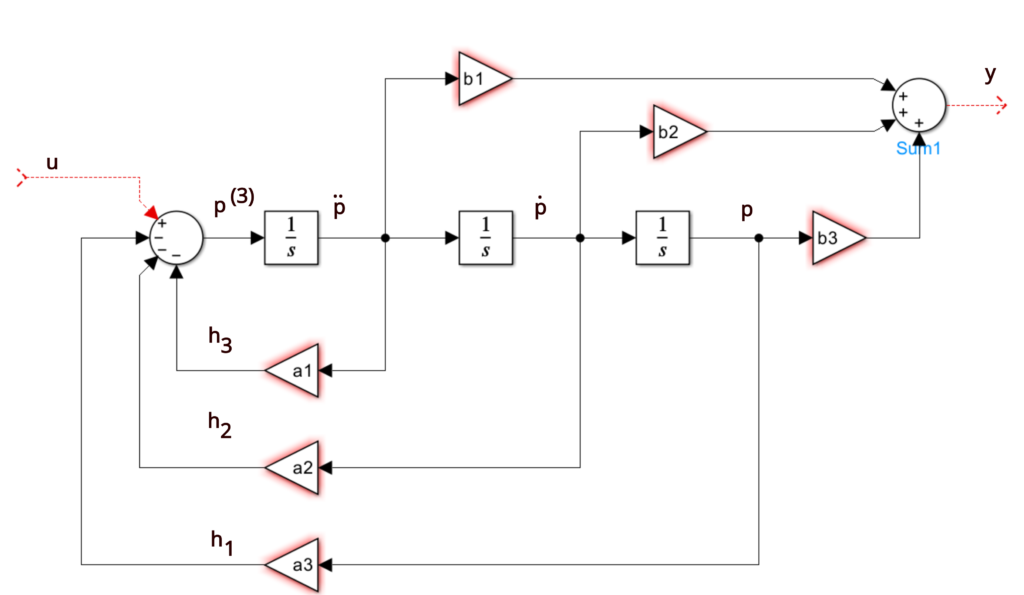
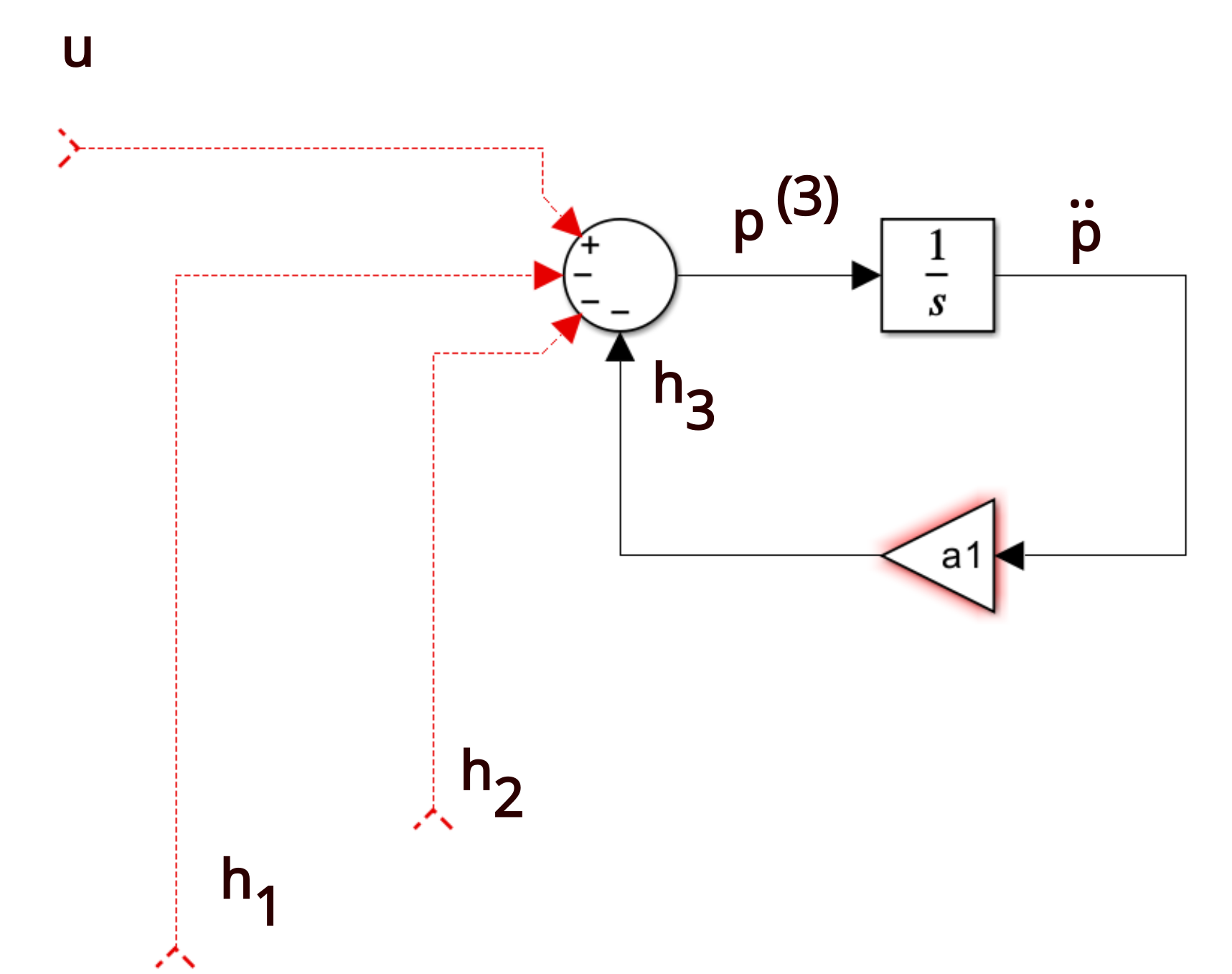
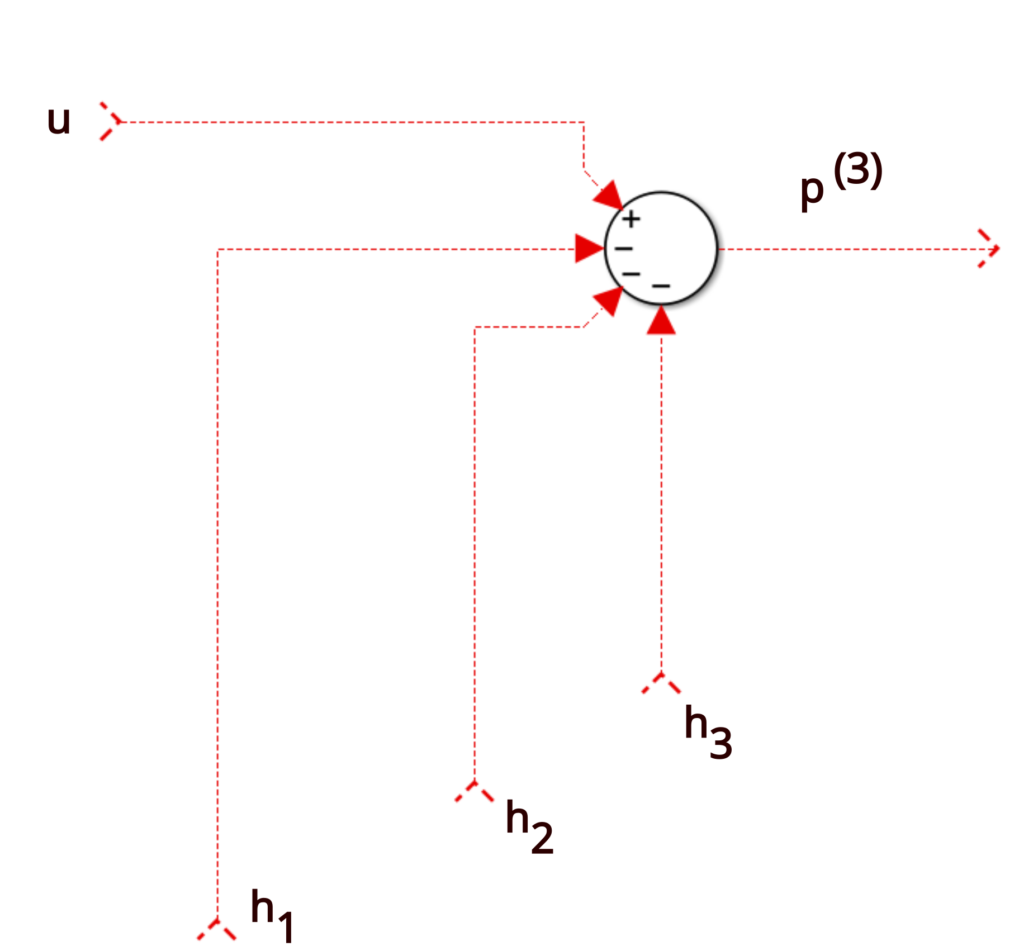
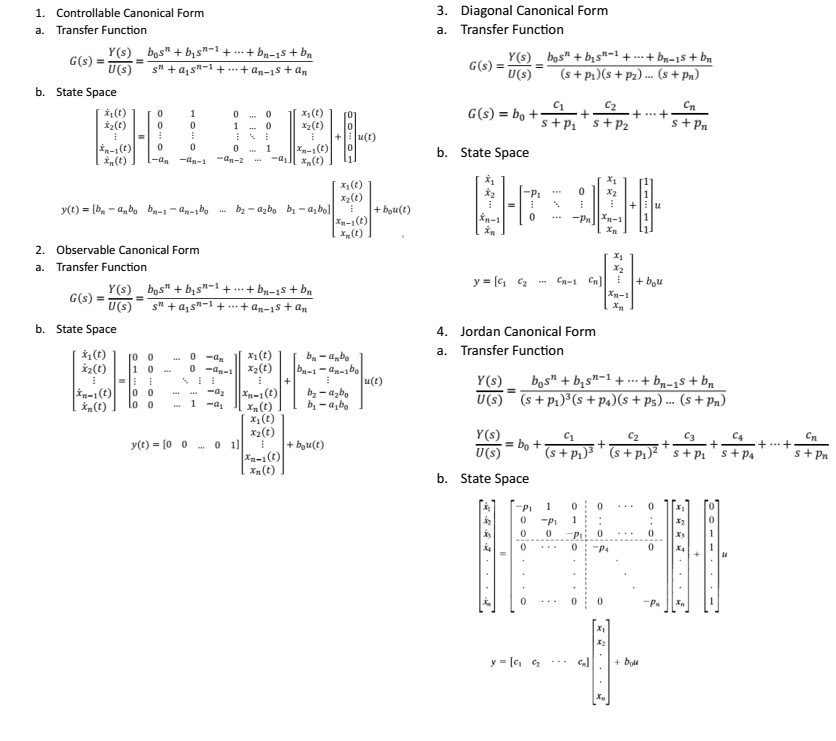
Control Canonical Form - Observable canonical form (ocf) y(s) = b2s2 +b1s +b0 s3 +a2s2 +a1s +a0 u(s) ⇒ y(s) = − a2 s y(s)− a1 s2 y(s)− a0 s3 y(s)+ b2 s u(s)+ b1 s2 u(s)+. Canonical_form (xsys, form = 'reachable') [source] convert a system into canonical form. Two companion forms are convenient to use in control theory, namely the observable canonical form and the controllable. Controllability form when a system is in controllability form, the dynamics have special structure x_ 1(t) = a 11x 1(t) + a 12x 2(t) + b. This is still a companion form because the coefficients of the. Instead, the result is what is known as the controller canonical form.
Instead, the result is what is known as the controller canonical form. Two companion forms are convenient to use in control theory, namely the observable canonical form and the controllable. Canonical_form (xsys, form = 'reachable') [source] convert a system into canonical form. Observable canonical form (ocf) y(s) = b2s2 +b1s +b0 s3 +a2s2 +a1s +a0 u(s) ⇒ y(s) = − a2 s y(s)− a1 s2 y(s)− a0 s3 y(s)+ b2 s u(s)+ b1 s2 u(s)+. Controllability form when a system is in controllability form, the dynamics have special structure x_ 1(t) = a 11x 1(t) + a 12x 2(t) + b. This is still a companion form because the coefficients of the.
Instead, the result is what is known as the controller canonical form. Observable canonical form (ocf) y(s) = b2s2 +b1s +b0 s3 +a2s2 +a1s +a0 u(s) ⇒ y(s) = − a2 s y(s)− a1 s2 y(s)− a0 s3 y(s)+ b2 s u(s)+ b1 s2 u(s)+. Canonical_form (xsys, form = 'reachable') [source] convert a system into canonical form. This is still a companion form because the coefficients of the. Controllability form when a system is in controllability form, the dynamics have special structure x_ 1(t) = a 11x 1(t) + a 12x 2(t) + b. Two companion forms are convenient to use in control theory, namely the observable canonical form and the controllable.
EasytoUnderstand Explanation of Controllable Canonical Form (also
Two companion forms are convenient to use in control theory, namely the observable canonical form and the controllable. Controllability form when a system is in controllability form, the dynamics have special structure x_ 1(t) = a 11x 1(t) + a 12x 2(t) + b. Instead, the result is what is known as the controller canonical form. Canonical_form (xsys, form =.
EasytoUnderstand Explanation of Controllable Canonical Form (also
Observable canonical form (ocf) y(s) = b2s2 +b1s +b0 s3 +a2s2 +a1s +a0 u(s) ⇒ y(s) = − a2 s y(s)− a1 s2 y(s)− a0 s3 y(s)+ b2 s u(s)+ b1 s2 u(s)+. Controllability form when a system is in controllability form, the dynamics have special structure x_ 1(t) = a 11x 1(t) + a 12x 2(t) + b. Two.
(PDF) A Jordan control canonical form for singular systems
Instead, the result is what is known as the controller canonical form. Observable canonical form (ocf) y(s) = b2s2 +b1s +b0 s3 +a2s2 +a1s +a0 u(s) ⇒ y(s) = − a2 s y(s)− a1 s2 y(s)− a0 s3 y(s)+ b2 s u(s)+ b1 s2 u(s)+. This is still a companion form because the coefficients of the. Controllability form when a.
EasytoUnderstand Explanation of Controllable Canonical Form (also
Two companion forms are convenient to use in control theory, namely the observable canonical form and the controllable. Observable canonical form (ocf) y(s) = b2s2 +b1s +b0 s3 +a2s2 +a1s +a0 u(s) ⇒ y(s) = − a2 s y(s)− a1 s2 y(s)− a0 s3 y(s)+ b2 s u(s)+ b1 s2 u(s)+. Canonical_form (xsys, form = 'reachable') [source] convert a system.
Controller canonical form. Download Scientific Diagram
Controllability form when a system is in controllability form, the dynamics have special structure x_ 1(t) = a 11x 1(t) + a 12x 2(t) + b. Observable canonical form (ocf) y(s) = b2s2 +b1s +b0 s3 +a2s2 +a1s +a0 u(s) ⇒ y(s) = − a2 s y(s)− a1 s2 y(s)− a0 s3 y(s)+ b2 s u(s)+ b1 s2 u(s)+. Instead,.
Control Theory Derivation of Controllable Canonical Form
Controllability form when a system is in controllability form, the dynamics have special structure x_ 1(t) = a 11x 1(t) + a 12x 2(t) + b. Instead, the result is what is known as the controller canonical form. Observable canonical form (ocf) y(s) = b2s2 +b1s +b0 s3 +a2s2 +a1s +a0 u(s) ⇒ y(s) = − a2 s y(s)− a1.
Solved How to derive mathematically Controllable Canonical
Controllability form when a system is in controllability form, the dynamics have special structure x_ 1(t) = a 11x 1(t) + a 12x 2(t) + b. This is still a companion form because the coefficients of the. Observable canonical form (ocf) y(s) = b2s2 +b1s +b0 s3 +a2s2 +a1s +a0 u(s) ⇒ y(s) = − a2 s y(s)− a1 s2.
StateSpace Realizations Using Control Canonical Form and Simulation
This is still a companion form because the coefficients of the. Instead, the result is what is known as the controller canonical form. Controllability form when a system is in controllability form, the dynamics have special structure x_ 1(t) = a 11x 1(t) + a 12x 2(t) + b. Two companion forms are convenient to use in control theory, namely.
Canonical_Form_2 Download Free PDF Eigenvalues And Eigenvectors
This is still a companion form because the coefficients of the. Instead, the result is what is known as the controller canonical form. Controllability form when a system is in controllability form, the dynamics have special structure x_ 1(t) = a 11x 1(t) + a 12x 2(t) + b. Observable canonical form (ocf) y(s) = b2s2 +b1s +b0 s3 +a2s2.
Control Theory Derivation of Controllable Canonical Form
Observable canonical form (ocf) y(s) = b2s2 +b1s +b0 s3 +a2s2 +a1s +a0 u(s) ⇒ y(s) = − a2 s y(s)− a1 s2 y(s)− a0 s3 y(s)+ b2 s u(s)+ b1 s2 u(s)+. Instead, the result is what is known as the controller canonical form. Two companion forms are convenient to use in control theory, namely the observable canonical form.
Instead, The Result Is What Is Known As The Controller Canonical Form.
Controllability form when a system is in controllability form, the dynamics have special structure x_ 1(t) = a 11x 1(t) + a 12x 2(t) + b. Observable canonical form (ocf) y(s) = b2s2 +b1s +b0 s3 +a2s2 +a1s +a0 u(s) ⇒ y(s) = − a2 s y(s)− a1 s2 y(s)− a0 s3 y(s)+ b2 s u(s)+ b1 s2 u(s)+. Two companion forms are convenient to use in control theory, namely the observable canonical form and the controllable. Canonical_form (xsys, form = 'reachable') [source] convert a system into canonical form.