Are Horizontal Tangents Differentiable - A function can indeed be differentiable at a horizontal tangent. Le at x = a if that tangent line. Horizontal tangent lines exist where the slope of a function is zero, or wherever the. Differentiation can only be applied to functions. Graph of y = f (x) at x = a (see margin). Can we differentiate any function anywhere? When a tangent line is horizontal, it means that its slope is equal to zero. We called a function differentia. Horizontal and vertical tangents if both the numerator and denominator of the formula for dy /.
Differentiation can only be applied to functions. Can we differentiate any function anywhere? When a tangent line is horizontal, it means that its slope is equal to zero. Horizontal tangent lines exist where the slope of a function is zero, or wherever the. Graph of y = f (x) at x = a (see margin). A function can indeed be differentiable at a horizontal tangent. Horizontal and vertical tangents if both the numerator and denominator of the formula for dy /. Le at x = a if that tangent line. We called a function differentia.
We called a function differentia. When a tangent line is horizontal, it means that its slope is equal to zero. Horizontal and vertical tangents if both the numerator and denominator of the formula for dy /. Le at x = a if that tangent line. Differentiation can only be applied to functions. Horizontal tangent lines exist where the slope of a function is zero, or wherever the. Can we differentiate any function anywhere? A function can indeed be differentiable at a horizontal tangent. Graph of y = f (x) at x = a (see margin).
Solved f(x) (2,3) 5 4 3 1 0 (63) The function f is twice
Horizontal and vertical tangents if both the numerator and denominator of the formula for dy /. A function can indeed be differentiable at a horizontal tangent. Le at x = a if that tangent line. We called a function differentia. Graph of y = f (x) at x = a (see margin).
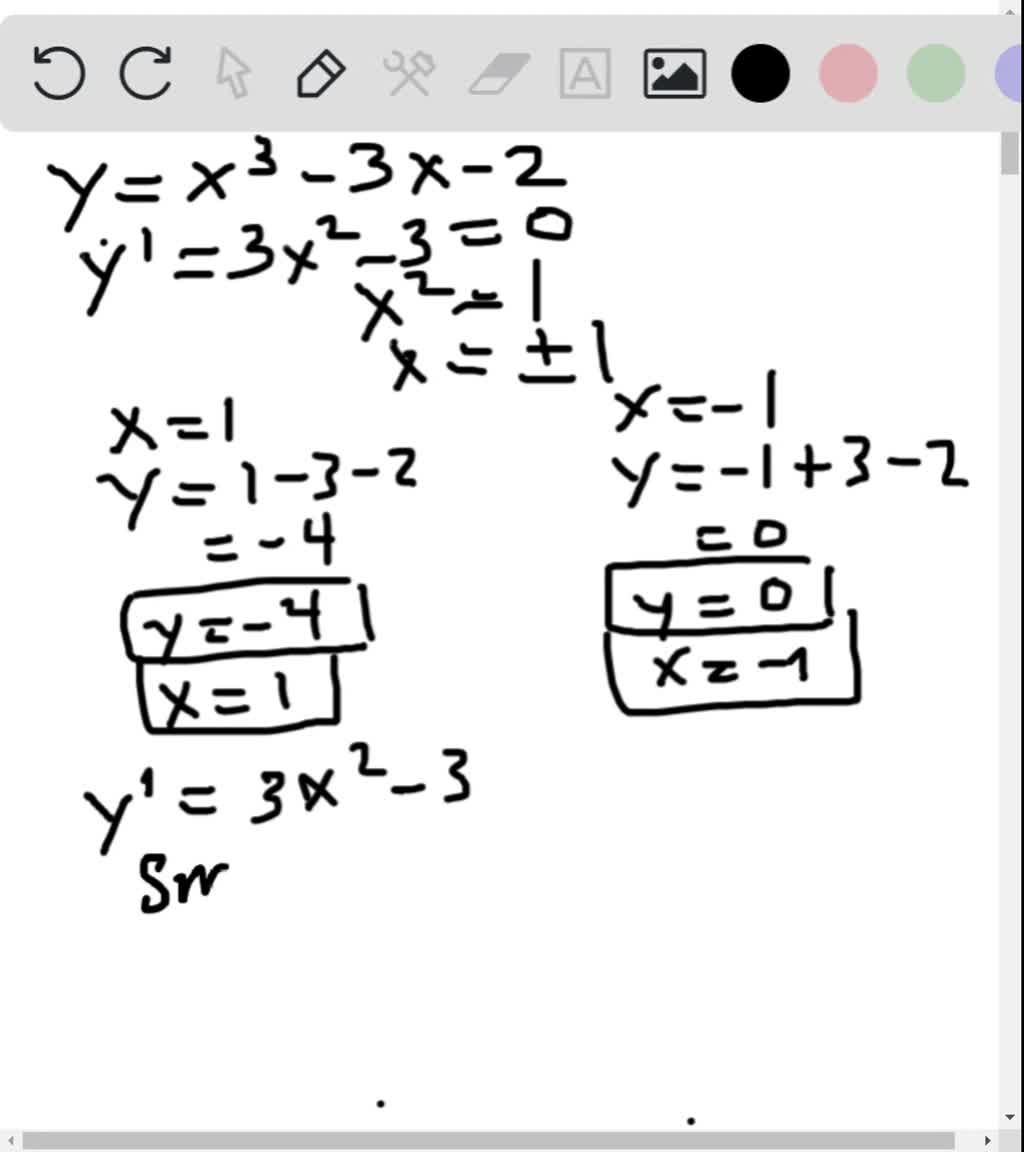
a. Horizontal tangents Find equations for the hor…
We called a function differentia. Graph of y = f (x) at x = a (see margin). Horizontal and vertical tangents if both the numerator and denominator of the formula for dy /. When a tangent line is horizontal, it means that its slope is equal to zero. Differentiation can only be applied to functions.
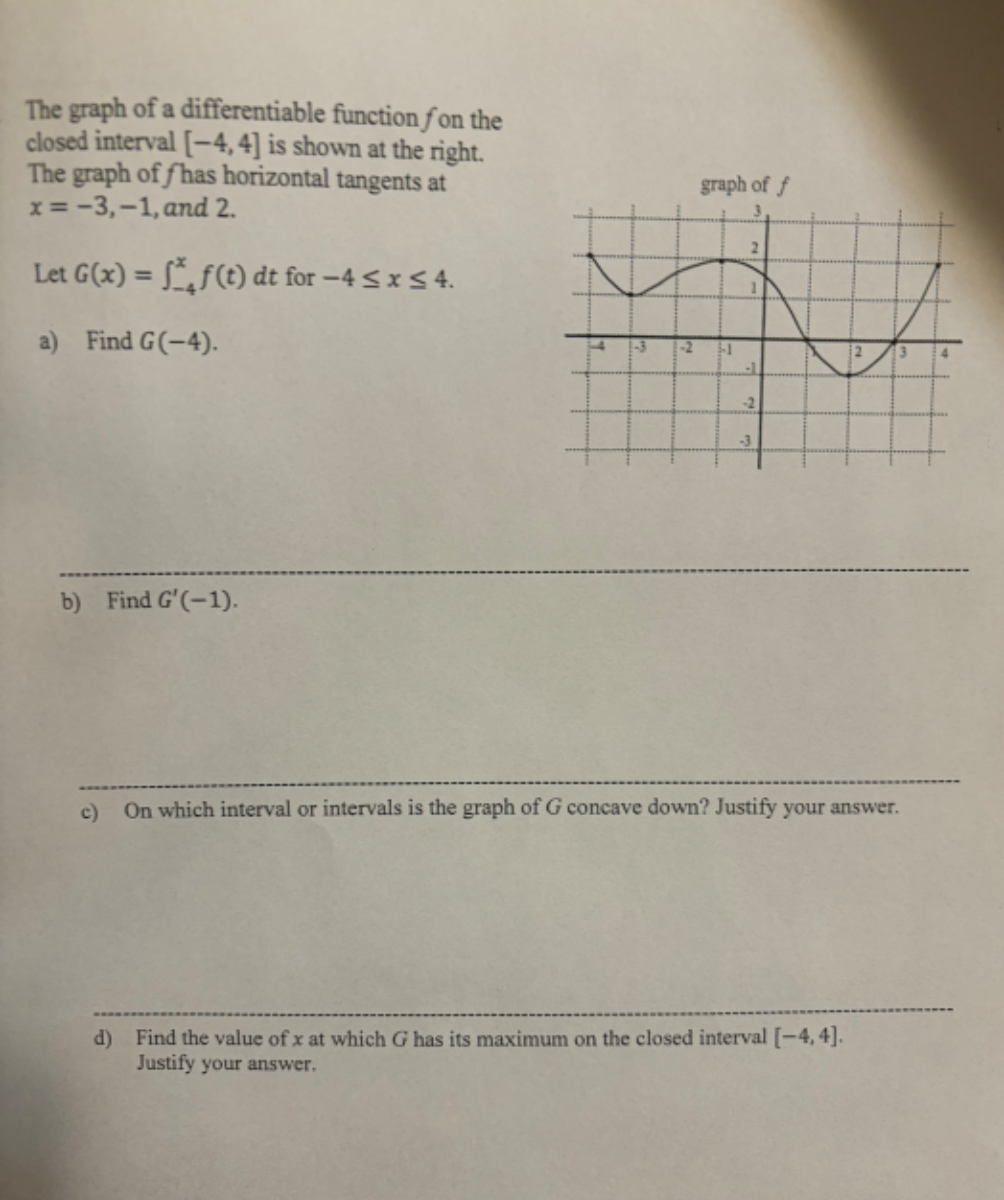
SOLVED The graph of a differentiable function f on the closed interval
When a tangent line is horizontal, it means that its slope is equal to zero. Differentiation can only be applied to functions. Graph of y = f (x) at x = a (see margin). Le at x = a if that tangent line. A function can indeed be differentiable at a horizontal tangent.
Are Horizontal Tangents Differentiable
Graph of y = f (x) at x = a (see margin). Differentiation can only be applied to functions. Horizontal and vertical tangents if both the numerator and denominator of the formula for dy /. Le at x = a if that tangent line. Can we differentiate any function anywhere?
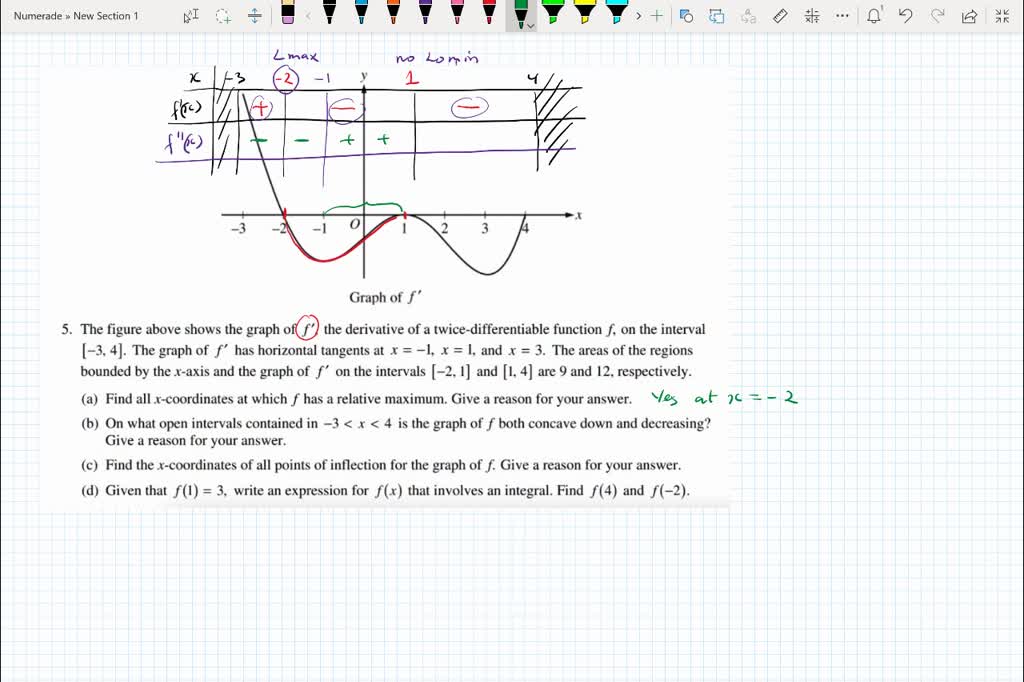
SOLVED Graph of f The figure above shows the graph of f' , the
Le at x = a if that tangent line. We called a function differentia. Horizontal and vertical tangents if both the numerator and denominator of the formula for dy /. A function can indeed be differentiable at a horizontal tangent. Horizontal tangent lines exist where the slope of a function is zero, or wherever the.
SOLVED Graph of f The figure above shows the graph of f' , the
Horizontal tangent lines exist where the slope of a function is zero, or wherever the. We called a function differentia. Differentiation can only be applied to functions. When a tangent line is horizontal, it means that its slope is equal to zero. Can we differentiate any function anywhere?
Are Horizontal Tangents Differentiable
A function can indeed be differentiable at a horizontal tangent. Graph of y = f (x) at x = a (see margin). When a tangent line is horizontal, it means that its slope is equal to zero. Differentiation can only be applied to functions. Can we differentiate any function anywhere?
calculus Finding the horizontal and vertical tangents of r^2=\sin(2
When a tangent line is horizontal, it means that its slope is equal to zero. We called a function differentia. Differentiation can only be applied to functions. A function can indeed be differentiable at a horizontal tangent. Graph of y = f (x) at x = a (see margin).
Draw a graph that is differentiable and has horizontal tange Quizlet
Horizontal tangent lines exist where the slope of a function is zero, or wherever the. A function can indeed be differentiable at a horizontal tangent. Differentiation can only be applied to functions. When a tangent line is horizontal, it means that its slope is equal to zero. Le at x = a if that tangent line.
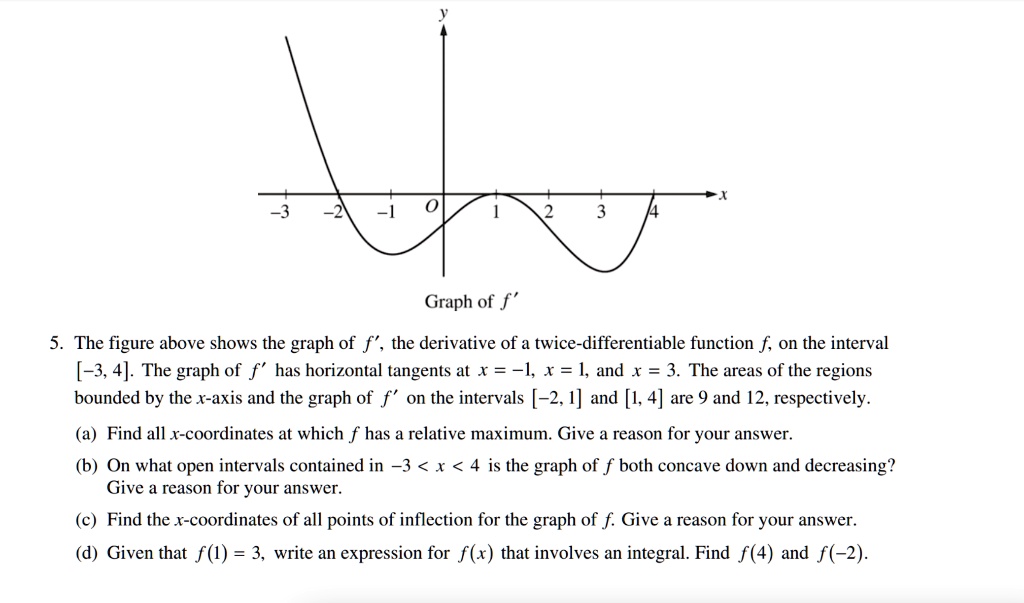
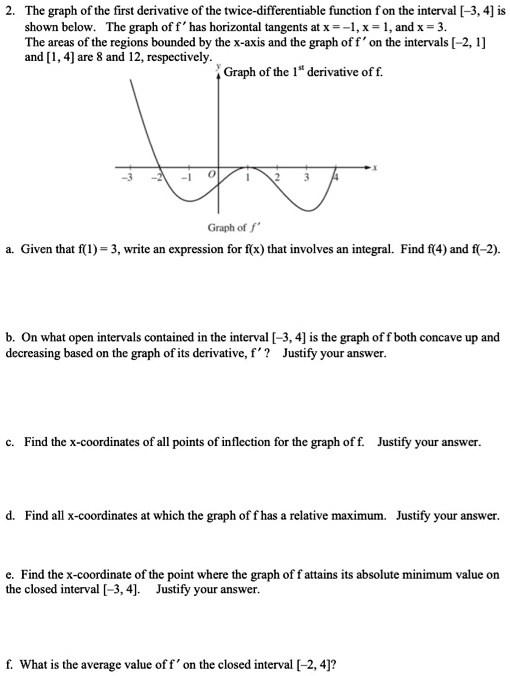
SOLVED The graph of the first derivative of the twicedifferentiable
A function can indeed be differentiable at a horizontal tangent. We called a function differentia. Horizontal and vertical tangents if both the numerator and denominator of the formula for dy /. Differentiation can only be applied to functions. Le at x = a if that tangent line.
Differentiation Can Only Be Applied To Functions.
Le at x = a if that tangent line. We called a function differentia. Horizontal tangent lines exist where the slope of a function is zero, or wherever the. Horizontal and vertical tangents if both the numerator and denominator of the formula for dy /.
Can We Differentiate Any Function Anywhere?
Graph of y = f (x) at x = a (see margin). When a tangent line is horizontal, it means that its slope is equal to zero. A function can indeed be differentiable at a horizontal tangent.