Are All Polynomials Differentiable - The correct definition of differentiable functions eventually shows that polynomials are. A polynomial of degree $n$ is the sum of terms of the form $a_kx^k$ where $0\leq. Yes, polynomials are infinitely many times differentiable, and yes, after some finite number. All of the standard functions are differentiable except at certain singular points, as follows:. In this article, we'll explore what it means for a function to be differentiable in simple terms. A differentiable function is a function whose derivative exists at each point in the. All polynomial functions are infinitely differentiable (graphed at desmos.com) an infinitely. Polynomials are differentiable onr) for all n∈n, the monomial.
All of the standard functions are differentiable except at certain singular points, as follows:. Polynomials are differentiable onr) for all n∈n, the monomial. Yes, polynomials are infinitely many times differentiable, and yes, after some finite number. A polynomial of degree $n$ is the sum of terms of the form $a_kx^k$ where $0\leq. A differentiable function is a function whose derivative exists at each point in the. In this article, we'll explore what it means for a function to be differentiable in simple terms. All polynomial functions are infinitely differentiable (graphed at desmos.com) an infinitely. The correct definition of differentiable functions eventually shows that polynomials are.
All polynomial functions are infinitely differentiable (graphed at desmos.com) an infinitely. A differentiable function is a function whose derivative exists at each point in the. In this article, we'll explore what it means for a function to be differentiable in simple terms. The correct definition of differentiable functions eventually shows that polynomials are. Yes, polynomials are infinitely many times differentiable, and yes, after some finite number. A polynomial of degree $n$ is the sum of terms of the form $a_kx^k$ where $0\leq. All of the standard functions are differentiable except at certain singular points, as follows:. Polynomials are differentiable onr) for all n∈n, the monomial.
Dividing Polynomials Worksheets Divide each polynomial Algebra 1
A polynomial of degree $n$ is the sum of terms of the form $a_kx^k$ where $0\leq. All of the standard functions are differentiable except at certain singular points, as follows:. Polynomials are differentiable onr) for all n∈n, the monomial. The correct definition of differentiable functions eventually shows that polynomials are. In this article, we'll explore what it means for a.
Names Of Polynomials By Degree iCalculator™
Polynomials are differentiable onr) for all n∈n, the monomial. A polynomial of degree $n$ is the sum of terms of the form $a_kx^k$ where $0\leq. The correct definition of differentiable functions eventually shows that polynomials are. A differentiable function is a function whose derivative exists at each point in the. Yes, polynomials are infinitely many times differentiable, and yes, after.
(PDF) Chaotic polynomials in spaces of continuous and differentiable
A polynomial of degree $n$ is the sum of terms of the form $a_kx^k$ where $0\leq. The correct definition of differentiable functions eventually shows that polynomials are. Polynomials are differentiable onr) for all n∈n, the monomial. A differentiable function is a function whose derivative exists at each point in the. Yes, polynomials are infinitely many times differentiable, and yes, after.
Algebra Dividing Polynomials Math Workbook 100 Worksheets Hands
A polynomial of degree $n$ is the sum of terms of the form $a_kx^k$ where $0\leq. Polynomials are differentiable onr) for all n∈n, the monomial. In this article, we'll explore what it means for a function to be differentiable in simple terms. All polynomial functions are infinitely differentiable (graphed at desmos.com) an infinitely. All of the standard functions are differentiable.
Factoring All Types Of Polynomials Worksheet
In this article, we'll explore what it means for a function to be differentiable in simple terms. All polynomial functions are infinitely differentiable (graphed at desmos.com) an infinitely. All of the standard functions are differentiable except at certain singular points, as follows:. Yes, polynomials are infinitely many times differentiable, and yes, after some finite number. A polynomial of degree $n$.
Differentiation of Polynomials Questions
A polynomial of degree $n$ is the sum of terms of the form $a_kx^k$ where $0\leq. All of the standard functions are differentiable except at certain singular points, as follows:. The correct definition of differentiable functions eventually shows that polynomials are. In this article, we'll explore what it means for a function to be differentiable in simple terms. All polynomial.
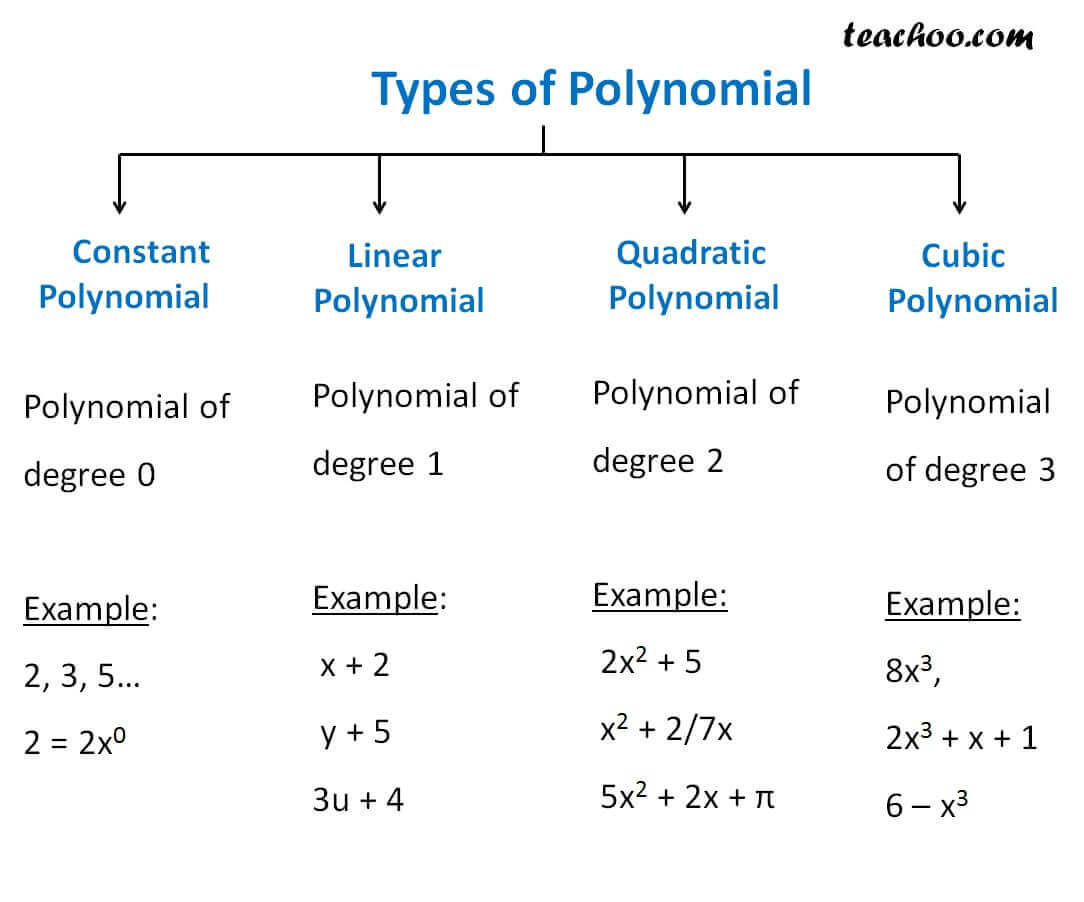
Types of Polynomials
All polynomial functions are infinitely differentiable (graphed at desmos.com) an infinitely. In this article, we'll explore what it means for a function to be differentiable in simple terms. A polynomial of degree $n$ is the sum of terms of the form $a_kx^k$ where $0\leq. A differentiable function is a function whose derivative exists at each point in the. The correct.
Polynomials
Yes, polynomials are infinitely many times differentiable, and yes, after some finite number. A polynomial of degree $n$ is the sum of terms of the form $a_kx^k$ where $0\leq. The correct definition of differentiable functions eventually shows that polynomials are. A differentiable function is a function whose derivative exists at each point in the. Polynomials are differentiable onr) for all.
Differentiable function Wikiwand
Polynomials are differentiable onr) for all n∈n, the monomial. The correct definition of differentiable functions eventually shows that polynomials are. A polynomial of degree $n$ is the sum of terms of the form $a_kx^k$ where $0\leq. Yes, polynomials are infinitely many times differentiable, and yes, after some finite number. A differentiable function is a function whose derivative exists at each.
algebraic geometry Are tropical polynomials differentiable
A differentiable function is a function whose derivative exists at each point in the. Yes, polynomials are infinitely many times differentiable, and yes, after some finite number. All of the standard functions are differentiable except at certain singular points, as follows:. The correct definition of differentiable functions eventually shows that polynomials are. Polynomials are differentiable onr) for all n∈n, the.
In This Article, We'll Explore What It Means For A Function To Be Differentiable In Simple Terms.
A differentiable function is a function whose derivative exists at each point in the. All of the standard functions are differentiable except at certain singular points, as follows:. The correct definition of differentiable functions eventually shows that polynomials are. Polynomials are differentiable onr) for all n∈n, the monomial.
All Polynomial Functions Are Infinitely Differentiable (Graphed At Desmos.com) An Infinitely.
A polynomial of degree $n$ is the sum of terms of the form $a_kx^k$ where $0\leq. Yes, polynomials are infinitely many times differentiable, and yes, after some finite number.