Differentiate Y Sec Θ Tan Θ - To differentiate the expression y = sec θ tan θ, we need to use the product rule of differentiation, which is (u.v)' = u'.v + u.v', where u = sec θ and v = tan θ. Since sec(θ)tan(θ) sec (θ) tan (θ) is constant with respect to ??, the derivative of sec(θ)tan(θ) sec (θ) tan (θ) with respect to ?? There are 2 steps to solve this one. The product rule states that if we have two functions u(θ) and v(θ), then the. Not the question you’re looking for? Free math problem solver answers your. To find the derivative of the function y = sec(θ)tan(θ), we use the product rule of differentiation.
To find the derivative of the function y = sec(θ)tan(θ), we use the product rule of differentiation. The product rule states that if we have two functions u(θ) and v(θ), then the. Since sec(θ)tan(θ) sec (θ) tan (θ) is constant with respect to ??, the derivative of sec(θ)tan(θ) sec (θ) tan (θ) with respect to ?? To differentiate the expression y = sec θ tan θ, we need to use the product rule of differentiation, which is (u.v)' = u'.v + u.v', where u = sec θ and v = tan θ. Not the question you’re looking for? There are 2 steps to solve this one. Free math problem solver answers your.
Since sec(θ)tan(θ) sec (θ) tan (θ) is constant with respect to ??, the derivative of sec(θ)tan(θ) sec (θ) tan (θ) with respect to ?? Free math problem solver answers your. There are 2 steps to solve this one. To find the derivative of the function y = sec(θ)tan(θ), we use the product rule of differentiation. Not the question you’re looking for? The product rule states that if we have two functions u(θ) and v(θ), then the. To differentiate the expression y = sec θ tan θ, we need to use the product rule of differentiation, which is (u.v)' = u'.v + u.v', where u = sec θ and v = tan θ.
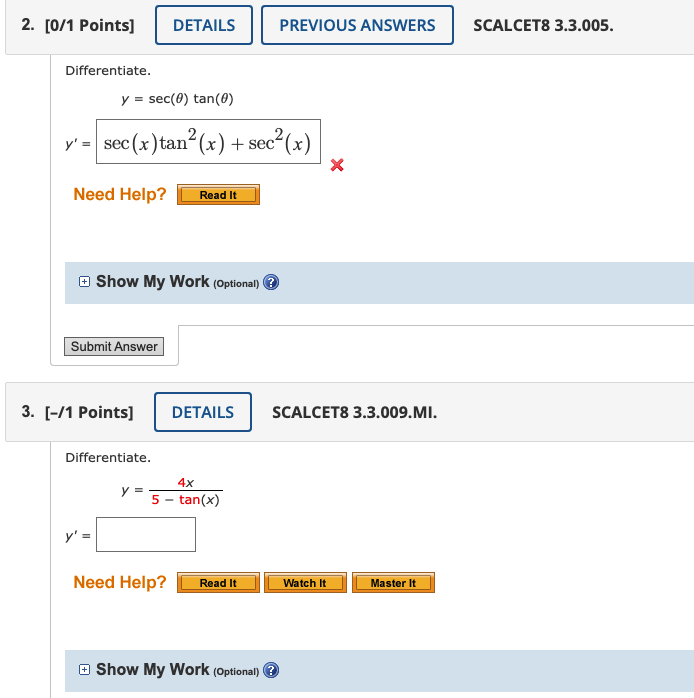
Solved Differentiate. y=sec(θ)tan(θ)y′=sec(x)tan2(x)+sec2(x)
Free math problem solver answers your. The product rule states that if we have two functions u(θ) and v(θ), then the. There are 2 steps to solve this one. Since sec(θ)tan(θ) sec (θ) tan (θ) is constant with respect to ??, the derivative of sec(θ)tan(θ) sec (θ) tan (θ) with respect to ?? To find the derivative of the function.
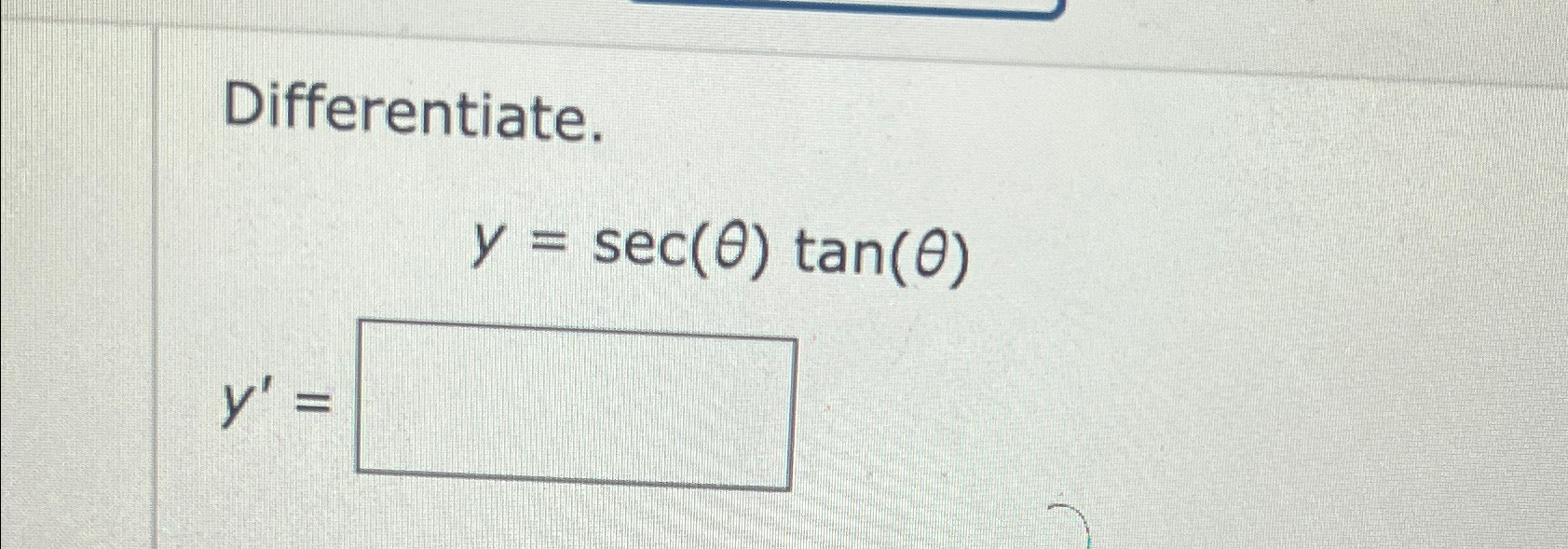
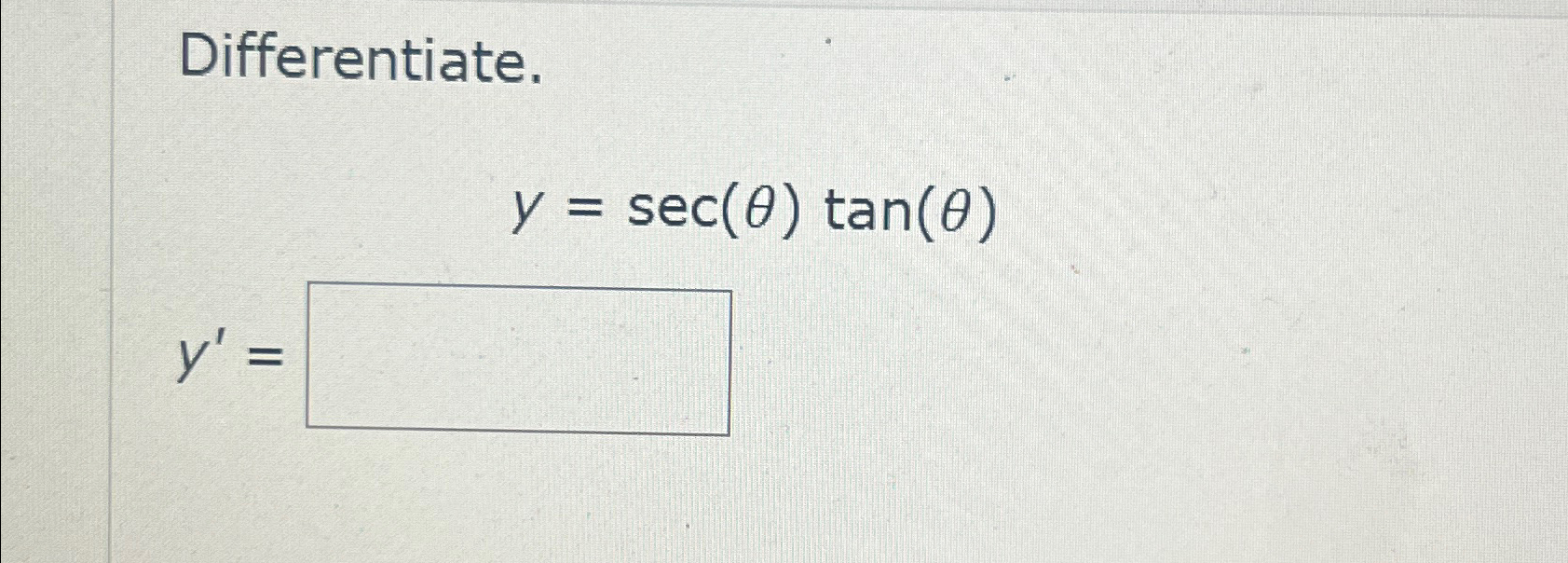
Solved Differentiate.y=sec(θ)tan(θ)y'=
To find the derivative of the function y = sec(θ)tan(θ), we use the product rule of differentiation. There are 2 steps to solve this one. To differentiate the expression y = sec θ tan θ, we need to use the product rule of differentiation, which is (u.v)' = u'.v + u.v', where u = sec θ and v = tan.
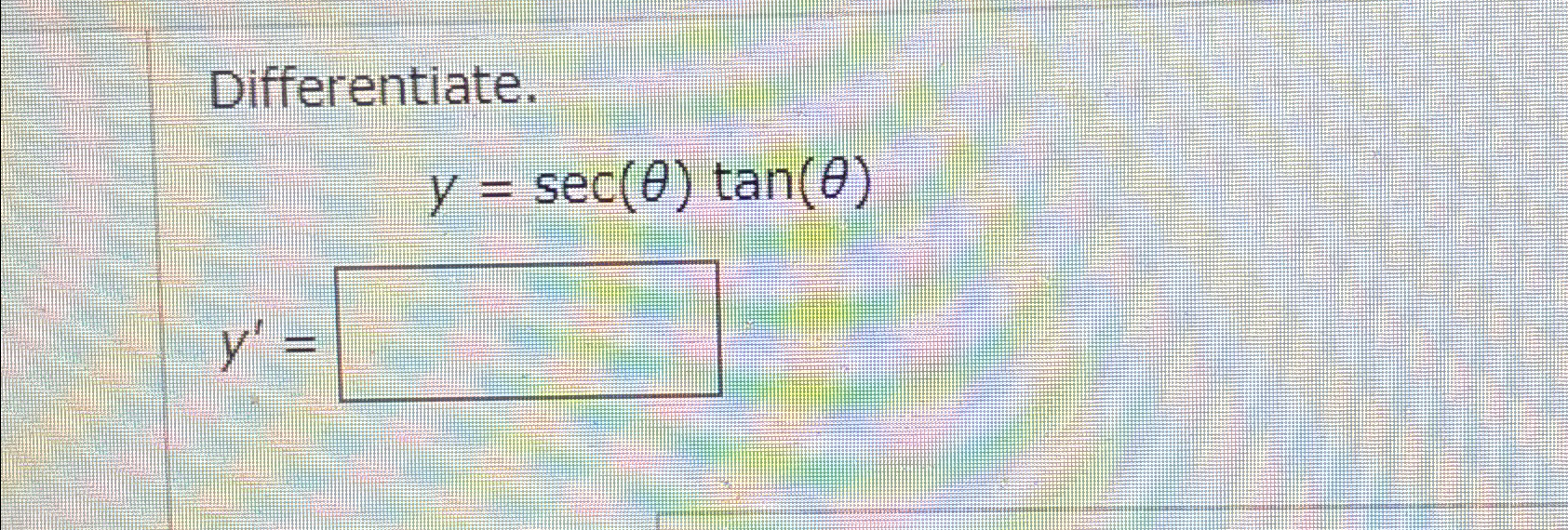
Solved Differentiate.y=sec(θ)tan(θ)y'=
Not the question you’re looking for? The product rule states that if we have two functions u(θ) and v(θ), then the. There are 2 steps to solve this one. Free math problem solver answers your. To differentiate the expression y = sec θ tan θ, we need to use the product rule of differentiation, which is (u.v)' = u'.v +.
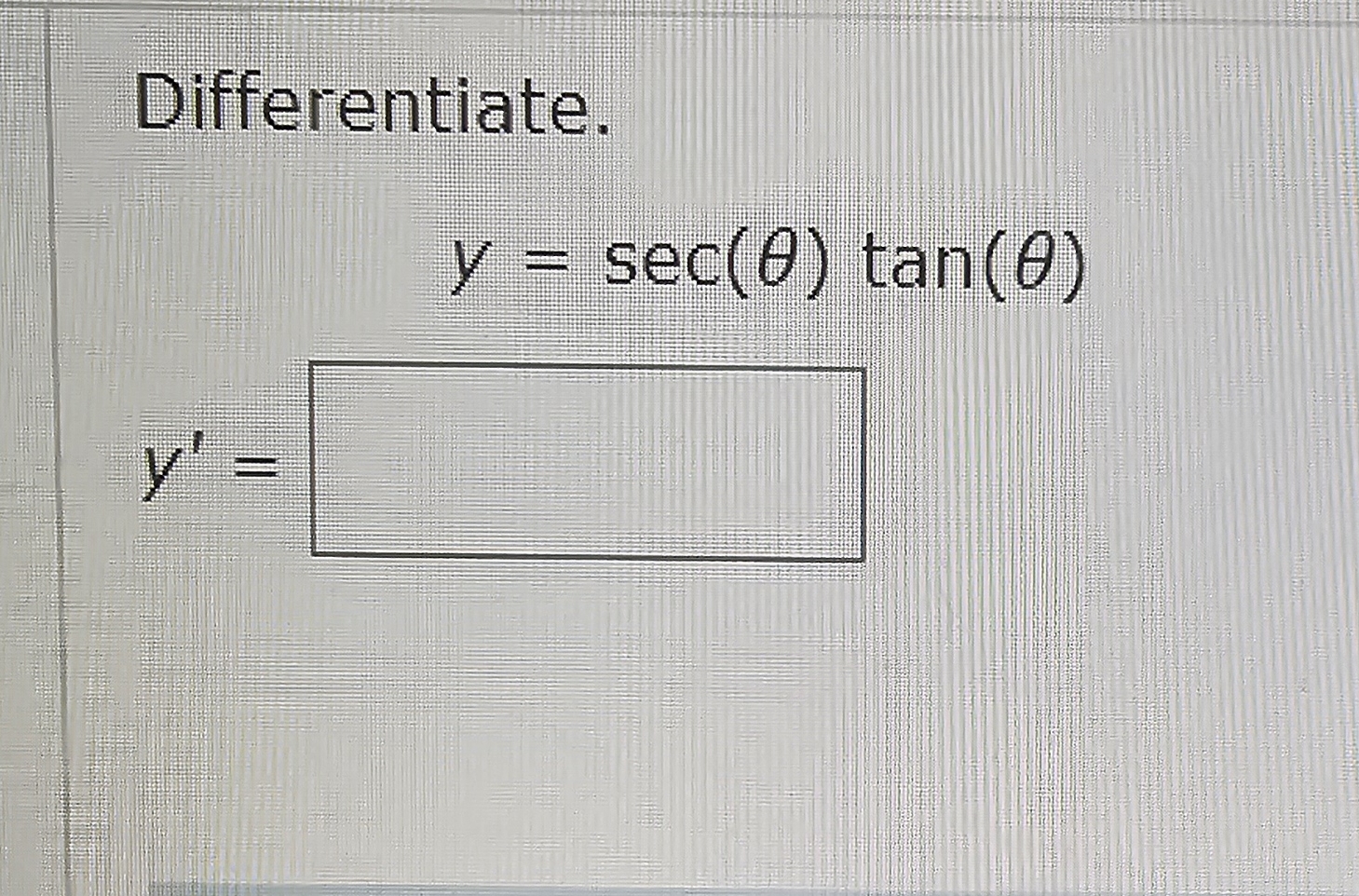
Solved Differentiate.y=sec(θ)tan(θ)y'=
To find the derivative of the function y = sec(θ)tan(θ), we use the product rule of differentiation. There are 2 steps to solve this one. Not the question you’re looking for? Free math problem solver answers your. The product rule states that if we have two functions u(θ) and v(θ), then the.
Solved Differentiate.y=sec(θ)tan(θ)y'=
To differentiate the expression y = sec θ tan θ, we need to use the product rule of differentiation, which is (u.v)' = u'.v + u.v', where u = sec θ and v = tan θ. Since sec(θ)tan(θ) sec (θ) tan (θ) is constant with respect to ??, the derivative of sec(θ)tan(θ) sec (θ) tan (θ) with respect to ??.
Solved Differentiate y = 1sec x/tan x sec x (1sec x)/tan^2
Free math problem solver answers your. To find the derivative of the function y = sec(θ)tan(θ), we use the product rule of differentiation. Since sec(θ)tan(θ) sec (θ) tan (θ) is constant with respect to ??, the derivative of sec(θ)tan(θ) sec (θ) tan (θ) with respect to ?? There are 2 steps to solve this one. To differentiate the expression y.
Solved Differentiate y = 1sec x/tan x sec x (1sec x)/tan^2
To differentiate the expression y = sec θ tan θ, we need to use the product rule of differentiation, which is (u.v)' = u'.v + u.v', where u = sec θ and v = tan θ. Not the question you’re looking for? Free math problem solver answers your. There are 2 steps to solve this one. To find the derivative.
Solved Differentiate.y=sec(θ)tan(θ)y'=
Free math problem solver answers your. To differentiate the expression y = sec θ tan θ, we need to use the product rule of differentiation, which is (u.v)' = u'.v + u.v', where u = sec θ and v = tan θ. To find the derivative of the function y = sec(θ)tan(θ), we use the product rule of differentiation. There.
Solved Differentiate the following function. y=sec (θ )(θ tan (θ
To differentiate the expression y = sec θ tan θ, we need to use the product rule of differentiation, which is (u.v)' = u'.v + u.v', where u = sec θ and v = tan θ. Since sec(θ)tan(θ) sec (θ) tan (θ) is constant with respect to ??, the derivative of sec(θ)tan(θ) sec (θ) tan (θ) with respect to ??.
Solved Differentiate.y=sec(θ)tan(θ)y'=
Not the question you’re looking for? Since sec(θ)tan(θ) sec (θ) tan (θ) is constant with respect to ??, the derivative of sec(θ)tan(θ) sec (θ) tan (θ) with respect to ?? There are 2 steps to solve this one. To differentiate the expression y = sec θ tan θ, we need to use the product rule of differentiation, which is (u.v)'.
Not The Question You’re Looking For?
Free math problem solver answers your. Since sec(θ)tan(θ) sec (θ) tan (θ) is constant with respect to ??, the derivative of sec(θ)tan(θ) sec (θ) tan (θ) with respect to ?? There are 2 steps to solve this one. To differentiate the expression y = sec θ tan θ, we need to use the product rule of differentiation, which is (u.v)' = u'.v + u.v', where u = sec θ and v = tan θ.
The Product Rule States That If We Have Two Functions U(Θ) And V(Θ), Then The.
To find the derivative of the function y = sec(θ)tan(θ), we use the product rule of differentiation.